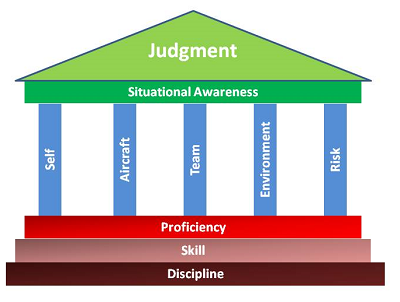
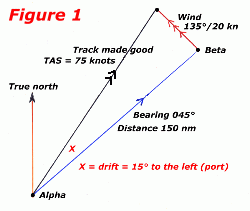
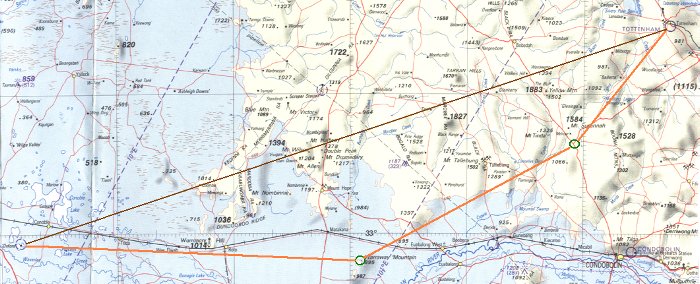
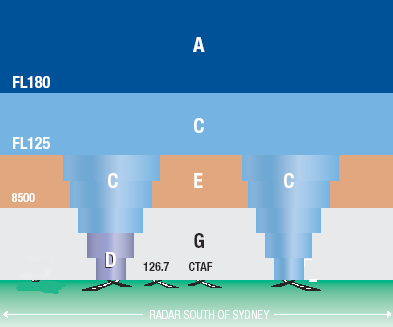
-
Posts
9,288 -
Joined
-
Last visited
-
Days Won
108
Content Type
Profiles
Forums
Gallery
Downloads
Blogs
Events
Store
Aircraft
Resources
Tutorials
Articles
Classifieds
Movies
Books
Community Map
Quizzes
Videos Directory
Everything posted by Admin
-
-
Granted we have been in a pandemic this year however has the writing been on the wall for recreational aviation for some time now and the pandemic has just been another nail in the coffin? Years ago we had many different aircraft avialable to buy and now there is only a handful of distributors and those are mostly plastic fantastics. The number of flying schools seems to have decreased and the costs have dramatically increased. Has this all come about since RAAus became a business and thus the demise of the Association of members, the social aspects of recreational aviation. Or, is it thriving and the pandemic has allowed it to thrive in bubbles ready to break back out. What do you think...is recreational aviation declining or thriving and if so why?
-
4.15.1 References CASR 91.225 - Safety during take-off and landing [not yet enabled July 2012] CASR 91.180 - Precautions before flight [not yet enabled July 2012] 4.15.2 Purpose This Advisory Circular (AC) provides general information and advice to enhance the safety of taking off or landing at any place, with emphasis on unlicensed aerodromes. It is intended to give an overview of pilot responsibilities and highlight principles applicable to the operations of smaller aeroplanes, including those not fully supported by manufacturer's take-off and landing performance data. 4.15.3 Status of this AC This AC is the first that has been issued on this subject. Advisory Circulars are intended to provide recommendations and guidance to illustrate a means but not necessarily the only means of complying with the Regulations, or to explain certain regulatory requirements by providing interpretative and explanatory material. Where an AC is referred to in a 'Note' below the regulation, the AC remains as guidance material. ACs should always be read in conjunction with the referenced regulations. 4.15.4 Definitions In this advisory circular, unless the contrary is stated: aerodrome means an area of land or water (including any buildings, installations and equipment), the use of which as an aerodrome is authorised under the regulations, being such an area intended for use wholly or partly for the arrival, departure or movement of aircraft. aircraft landing area (ALA) means a place which may be suitable for the landing and take-off of an aeroplane of appropriate certification and performance but which may not fully meet formal standards of construction, marking, maintenance or reporting. apron means a defined area on a land aerodrome intended to accommodate aircraft for purposes of loading or unloading, fuelling, parking or maintenance. clearway means a defined area in which there are no obstacles penetrating a slope of 2.5% rising from the end of the runway over a width of 45m. demonstrated landing distance (DLD) means an aeroplane?s unfactored landing distance as demonstrated by the aeroplane's manufacturer under a certification process. factor means a safety margin applied as a multiple to an aeroplane manufacturer's minimum take-off or landing distance data to allow for the variables experienced in normal operations (usually expressed as a percentage). float plane means any aeroplane designed for landing or taking-off from water. fly-over area means a portion of ground adjacent to the runway strip which is free of tree stumps, large rocks or stones, fencing or any other obstacles above ground, but which may include ditches or drains below ground level. landing area means a place which may be suitable for the take-off and/or landing of an aircraft under appropriate conditions. large aeroplane means an aeroplane over 5700kg MTOW. lateral clearance area means an area each side of a runway in which obstructions are graded upward away from the runway to reduce wind-shear and provide lateral clearance in the event of divergence from the runway centre-line (see Appendix A, Figure 1). manoeuvring area means that part of an aerodrome to be used for the take-off, landing and taxiing of aircraft, excluding aprons. maximum landing weight (MLW) means the maximum weight at which the aeroplane may land or alight. maximum take-off weight (MTOW) means the maximum weight at which an aeroplane may commence its take-off roll. movement means the taking off or landing of an aircraft. movement area means that part of an aerodrome to be used for the take-off, landing and taxiing of aircraft, consisting of the manoeuvring area and the apron(s). obstacle free area means an area where there are no wires or any other form of obstacles above the approach and take-off areas, runways, runway strips, fly-over areas or water channels. runway means a defined rectangular area on a land aerodrome which is prepared for the landing and take-off of aircraft. runway strip means a portion of ground between the runway and fly-over area which is in a condition that ensures minimal damage to an aeroplane which may run off a runway during take-off or landing. small aeroplane means an aeroplane not more than 5700kg MTOW. alighting area means a suitable stretch of water for the landing or taking-off of a float plane or amphibian under specific conditions. 4.15.5 General 4.15.5.1 CASRs 91.180 and 91.225 require pilots to assess aircraft performance in the prevailing circumstances in order to assure the safety of their aircraft when taking off or landing. The assessment of aircraft performance must include appropriate allowances for the variables that occur in normal operations. 4.15.5.2 Aircraft performance data is supplied in the Aircraft Flight Manual (AFM), Owners Manual, Pilot's Operating Handbook or an equivalent publication. 4.15.5.3 There is no legal obligation on pilots of private aeroplanes below 5700kg to apply safety margins (factors) to the take-off or landing distances recommended by the aeroplane manufacturer. However, the pilot cannot reasonably expect to meet optimum performance standards during normal field operations, and if recommended safety factors are not applied, he or she must accept a greater level of personal responsibility for ensuring that safe runway distances are available in the prevailing circumstances. *See the standard safety factors recommended for small aircraft at Table 1 to Appendix A. 4.15.6 Permission to operate and responsibilities 4.15.6.1 Permission to operate. There is ownership and management of almost every potential landing place, with the possible exception of open areas of water. Unless a landing place is unambiguously open to public use for aviation the pilot should assume that approval is required before using land or water for an aircraft movement. General examples of places where approval is required would be: an unlicensed landing ground managed by local council or private organisation/landowner private farmland roads, parks or fairways owned by local authorities or private interests water, land or dry lakes managed by a state authority such as National Parks, Waterways Authority, Lands Department, etc. 4.15.6.2 Penalties and liability. Use of a public facility such as a road or park for landing may attract a penalty from local authorities even if the physical requirements for a landing area are satisfied. Where land is not actively managed an unauthorised landing might still be considered a trespass. A liability for reckless or negligent operation may also arise if appropriate precautions were not taken to avoid conflict with other users. 4.15.6.2.1 While the law generally recognises a person's right to take any reasonable action to save themselves in an emergency, pilots should remember that nothing in the CASRs acts to protect him/her against civil liability in the case of damage to persons or property. 4.15.6.3 Pilot responsibilities. The pilot in command has responsibility for the safety of the aircraft and those on board. He or she is required to obtain and assess all the information needed for the safety of the flight, and must not take off or land unless the information indicates that the aeroplane can operate safely. 4.15.6.4 Aerodrome information. Whether operating at an aerodrome or an ALA the pilot of an aeroplane needs to know: the location of the aerodrome or ALA and the features that can be used to positively identify it as the aerodrome intended for landing. the means of identifying the boundaries of the manoeuvring area whether people, machines, stock or wildlife are likely to be present at the time of movement the length of (suitable) runway available the width of the runway the nature of the runway and movement area surface the runway elevation the runway direction the runway slope recency and type of usage: eg., use as agricultural strip, any current fixed-wing, gliding or parachute operations etc surface type: eg., seal, broken seal, black soil, sandy loam, naturally soft, naturally hard, gravel, small/larger stones, etc surface conditions: eg., cracked, sandy, soft gravel, muddy, recently ploughed, hardened mud (rutted or stock-pitted), heavily grassed, lightly grassed, etc. surface moisture levels: eg., dry, moist, wet, muddy ambient conditions: temperature, wind, general conditions obstructions in the approach, take-off and lateral transition areas power lines near the aerodrome any management limits on the use of the landing place any special procedures applicable at the landing place: eg one off activities NOTAMs or AIP Supplements applicable to the area 4.15.6.5 No-go situations. Every pilot must learn to resist personal and external pressures to proceed without essential safety information, or when evidence suggests safety is not reasonably assured. It is also important that other persons involved in the operation are made aware that no decision to proceed will be made until all required information has been assessed. Unless and until the operation is potentially safe both common sense and regulatory requirements mean that the take-off or landing must not be attempted. *Appendix A shows the operational elements that should be considered when assessing the safety of a proposed movement. 4.15.6.6 Landing area manager's responsibilities. A person who gives an approval to operate must be careful to stay within his or her level of expertise. The law will usually see the provision of information as an invitation to operate, and the person giving an invitation takes on a duty of care for the welfare of the person invited. In a matter of negligence the question to be answered will usually be "was the risk reasonably foreseeable to the visitor or the class of persons to whom the visitor belongs?" Nothing by way of conjecture should be said, and only information known beyond reasonable doubt to be accurate should be passed. If the person who is asked for advice about a landing place is not clearly aware of the facts needed to answer a question, he or she would be well advised not to volunteer an answer. Information found to be wrong should be corrected even if the pilot is on the way. It is essential that a person who issues an approval to land or take off an aeroplane either: (a) limit themselves to issuing approval to operate plus providing only known fixed information such as surveyed length of runway, aerodrome elevation and compass orientation; or (b) given an arguable awareness of the requirements on the basis of training and experience, provide fixed information plus variable surface conditions and weather information they have reasonable grounds to believe are correct. The duty of care ?Whenever a person gives information or advice to another upon a serious matter in circumstances where the speaker realises, or ought to realise, that he is being trusted to give the best of his information or advice for action on the part of the other party to act on that information or advice, the speaker comes under a duty to exercise reasonable care in the provision of the information or advice he chooses to give? L. Shaddock & Associates Pty Ltd v Parramatta City Council (1981) 150 CLR 225 In all cases the responsibility for safe operation rests with the pilot, and while the owner or manager has an obligation to provide factual information, he or she is not required to guarantee that an aerodrome is safe for landing or take-off. A landing area's owner or manager is less likely to be held responsible if the visitor is without a lawful right to use the aerodrome. However, the owner or manager of a clearly marked aerodrome, which is unserviceable or hazardous, would be wise to provide warning to others about its status. He or she could probably discharge any duty of care by removing the markings or by displaying an unserviceability cross on the aerodrome. 4.15.7 Assessing the suitability of an ALA 4.15.7.1 The suitability of a landing place depends on its characteristics, the aircraft to be used and the pilot's qualifications and skills. The landing place must meet the aircraft manufacturer's recommended minimum standards for dimensions, slope, surface bearing capacity, obstacle-free gradients and so forth. The weather conditions must also be considered. 4.15.7.2 A pilot is authorised by virtue of his or her licence to assess these factors before deciding whether a particular movement should take place. If a pilot fails to discover or consider any significant factor affecting the safety of a movement, he or she may be liable to action in negligence. 4.15.8 Criteria for a landing area Each aircraft movement calls for a landing and take-off area of certain dimensions. The individual aircraft's flight manual shows the dimensions required for given combinations of weight, altitude and temperature. *Appendix A, 'Dimensional and Other Guidelines for Aircraft Landing Areas (ALAs)' shows the minimum ALA physical standards for aeroplanes below 5700kg. Higher standards apply to licensed aerodromes, with the most stringent requirements being for licensed or private aerodromes where high capacity aeroplanes are used in commercial passenger transport operations. 4.15.9 Pressure and density altitude 4.15.9.1 Pressure altitude. A significant characteristic of a landing area is its height above sea level, which, combined with the QNH, gives rise to pressure altitude. The reduction of ambient air pressure with height increases the True Air Speed (TAS) required for a given Indicated Air Speed (IAS), which affects take-off and landing distance requirements. To determine pressure altitude, apply the QNH to the aerodrome elevation. Thus a pilot on a sea level aerodrome with a QNH of 1003 would calculate 1013-1003 = 10hpa x 28ft, giving a pressure altitude of 280ft AMSL. Alternatively a pilot can read pressure altitude directly by setting standard pressure 1013.25 on the altimeter subscale. 4.15.9.2 Density altitude. Increased density altitude markedly reduces engine power output (this effect can be delayed if an aircraft is fitted with a turbocharger, which can maintain a regulated inlet air pressure to flight level heights). If necessary, density altitude can be determined by applying the ambient temperature to the pressure altitude, with each 1 deg C variation from ISA giving rise to 120ft variation in density altitude. Thus a sea level aerodrome with ISA pressure and 25 deg would have a density altitude of 1200ft. The pilot does not usually have to make a separate density altitude calculation because take-off and landing performance charts usually provide integral solutions for density altitude through entries of pressure altitude and temperature. (For more information see 'High density altitude: effect on take-off/landing performance'.) 4.15.10 Aerodrome surface characteristics 4.15.10.1 Rolling resistance. Rolling resistance is determined by tyre pressure, aeroplane mass, and the surface characteristics of the movement area. Up to a point, rolling resistance may be welcome during landing, but unexpected rolling resistance on take-off can lead to a decision to abort the take-off, or possibly an over-run accident. The limits of safety during landing would be that which caused damage to the tyres or aeroplane structure, or loss of directional control. Low tyre pressure can have a very significant effect (note how difficult it is to shift an aeroplane by pushing it about the tarmac with low tyre pressures, or experience the difference between pushing a fully-loaded wheelbarrow with a very low tyre pressure versus the same load on a tyre with higher pressure). 4.15.10.2 Surface. The main variable in rolling resistance is the nature of the runway surface. The surface may be concrete, bitumen, coral, gravel, soil, grass on soil or sand, hard packed sand or a dry salt-bed (salt lake), each with its own characteristics, many of which vary with the weather and season. Obviously the rolling resistance on concrete or bitumen is minimal and predictable, but the rolling resistance on other types of surface varies widely between surface types, and will even vary with changes in surface solidity along the length of a given runway. Rolling resistance can be caused by standing water on any runway surface because it builds up in front of the wheels (witness the braking effect on a car driven across a water-covered causeway). In the case of any natural surface the soil moisture content significantly affects rolling resistance, as does surface looseness, presence of algal growth, grass mass and characteristics, surface irregularities, and subsurface softness. Very dry is helpful on some natural surfaces but detrimental on others. With the exception of beach sand very wet almost invariably gives rise to an unsatisfactory surface. Grass density, greenness and length have a significant effect on the rolling behaviour of an aeroplane (grass can also hide obstructions, holes, water, stones, anthills and erosion trenches). 4.15.10.3 Assessing the variables. Table 1 to Annex A provides some guidance about the effects of various surface conditions, but no table can reasonably cater for all scenarios or all factors, and a pilot must develop an ability to make his/her own assessments. Some of the factors that will affect the safety of take-off are: transverse or lateral slope, which can affect the aerodynamics of flight, and may also result in a longer take-off roll because the pilot has to use asymmetric brake, nosewheel steering or rudder to keep straight gravel, which may mean a longer take-off roll because power may have to be applied slowly during the initial roll to avoid stone-chip damage to the propeller, and may, if very soft, give rise to a wave effect in front of the wheels that resists forward motion sand, which is usually worse than gravel in terms of creating rolling resistance grass, which resists the passage of an aeroplane rolling over it: while attempts are made to predict the effects of certain lengths of grass, rolling resistance will vary not just with the length, but also freshness, moisture content, density of stalks and the mass of material present free water, which not only affects the softness or slipperiness of the surface but can build up in front of an aeroplane?s wheels and cause a resistance to rolling, or at higher speeds, lift the wheels and cause aquaplaning and difficulty in maintaining directional control water in soil, can create mud, which can affect an aircraft's direction control and may choke spats or wheel-wells and restrict rotation of its wheels. In addition, soft spots may allow an aeroplane's wheel(s) to sink enough for the propeller to hit the ground, or may cause erratic rates of acceleration during a take-off. bearing capacity, which is related to the type of runway surface and the aeroplane's weight and tyre pressure. If the bearing capacity is insufficient for the combination of aeroplane, tyres and surface, a form of bogging may occur even in dry conditions (as might be experienced when driving a vehicle over sand or a freshly ploughed paddock). 4.15.10.4 Reliability of information. Be aware that some ALAs may be managed by persons who have limited ability to assess the proposed landing area's operational status. A pilot could get approval to land and related information from the manager of a proposed landing area but may not have full confidence in the quality of the information received. In such a case the pilot has to accept that there is no basis for an operational decision to proceed, and should cancel the flight or operate to the nearest aerodrome (see the definition). 4.15.11 Ambient conditions 4.15.11.1 Wind-speed and direction. Every pilot knows that wind can dramatically affect the length of runway required for take-off or landing. Likewise, every aeroplane should be provided with charts that can be used to calculate the effects of various wind scenarios on performance and runway requirements. 4.15.11.2 Humidity. Light aeroplane data does not usually include a humidity correction, but all engines are adversely affected to some degree by high humidity because water vapour displaces oxygen and reduces temperature rise during combustion. If the aeroplane's documentation provides relevant information the pilot should allow for the effects of humidity in a critical take-off. 4.15.12 Aeroplane characteristics and configuration 4.15.12.1 Performance data. The performance of every certificated aircraft has been evaluated as a part of the certification process. The certification process allows the manufacturer to determine the take-off and landing performance under ideal conditions (see para 14.2). 4.15.12.2 Factoring. 'Factors' are safety margins applied to the calculation of take-off and landing distance requirements to allow for random variables in aircraft, pilot and runway surface performance encountered in normal operations. Safety factors need to be applied because an aeroplane manufacturer's advertised performance figures are determined by a company test pilot on a good surface using a finely tuned machine in ideal weather conditions. Private operations. As mentioned earlier, the regulations don't require the application of any factoring for small aeroplanes in private operations except in certain circumstances such as land and hold short operations (LAHSO), where a common standard has to apply to all participants. *See Appendix A Table 1. 4.15.12.4 Weight Altitude Temperature (WAT) limitations. [Not applicable to ultralight operations] 4.15.12.5 Performance category. [Not applicable to ultralight operations] 4.15.12.6 Flap settings. The use of flap during take-off may be optional, or it may be the standard configuration for the aircraft. The latter is most likely to be the case in aeroplanes with wings optimised for higher indicated airspeeds. Pilots should be aware that while flap generally reduces the take-off roll by permitting lift-off at a slower speed, drag is always increased and the rate of climb after take-off is reduced until flap is retracted. Despite the reduced rate of climb, use of flap will allow a slightly slower take-off safety speed, which usually results in a steeper climb angle than in the clean configuration. Where a take-off flap setting is optional, flap can be used to reduce rolling resistance on a soft or rough runway (and thus the length of the ground roll), but it would normally be retracted at a safe speed after take-off and the aeroplane then allowed to accelerate to the speed for best rate of climb. 4.15.12.7 Braking performance. The nominal braking performance of an aeroplane is a design feature of each aeroplane type. Braking performance is also a function of runway surface conditions, availability of anti-skid, the pilot's braking technique, tyre condition and inflation, brake disc condition and residual temperature, aeroplane speed and mass, and the presence of slush, mud, water and other more subtle factors. In the case of a minimum field landing in an aeroplane, which is not equipped with anti-skid, one of the major difficulties is the pilot's ability to judge the application of maximum brake just short of wheel-skid. All of the relevant factors must be considered when assessing the aeroplane's likely performance in a critical situation. 4.15.12.8 Reverse thrust. [Not applicable to ultralight operations] 4.15.12.9 Propeller strikes and engine damage. A muddy, irregular or watery surface on an aerodrome or taxiing area can cause a propeller tip strike that may not be noticed by the pilot. When operating on a natural surface runway, careful preflight inspections of the propeller(s) are imperative. If a tip-strike happens during taxi for take-off it could easily result in an abandoned take-off, and possibly a major accident. Any propeller strike will probably cause some form of blade bending, distortion or delamination, but a serious strike will bend the tips back, distort the angle of the blades, may damage a gear-case or bend the propeller shaft, and will usually give rise to severe and probably dangerous vibration. If damage has occurred it cannot be ignored and will not correct itself. If a pilot has any reason to at all to suspect that a propeller may have struck the ground during taxi for take-off the only rational thing to do is to shut down the engine and inspect the propeller before attempting take-off. 4.15.12.10 FOD, gravel and dust. Foreign object damage (FOD) to a turbine engine may cause loss of power or complete failure. FOD frequently arises when gravel is sprayed into the engine intake by the nosewheel, or picked up in a vortex under the engine intake at high power. Dust will damage both piston and turbine engines, but can be reduced in piston engines by use of filtered air. Pilots should be aware that full power for take-off is predicated on selection of unfiltered ram air, and that carburettor heat is usually unfiltered. Foreign objects, especially gravel, may cause propeller chipping and may give rise to propeller cracks after being caught up in the propeller tip vortices of an engine doing a run-up, or at high rpm during the early stages of take-off. The possibility of propeller damage by gravel may be a significant operational consideration in a take-off from an unswept bitumen or a natural surface, because while the potential for damage can be greatly reduced by progressive application of power during the take-off roll (thus reducing vortices), the take-off distance required is usually calculated assuming full power at brakes release. 4.15.13 Obstacles on and in the vicinity of an aerodrome 4.15.13.1 Runway ends. The ideal runway will have an extended surface that can be used for bringing an aeroplane to a safe halt after an over-run of the runway. An obstacle-free surface extension that is not sound enough to permit normal operation of an aeroplane may nevertheless minimise structural damage if an aeroplane undershoots or overruns the runway. 4.15.13.2 Obstruction-free areas. Obstruction free areas on a runway extended centre-line provide for low angles of take-off and safe clearance on approach. A significant clear area at the end of a runway may have an important psychological effect on the way a pilot handles an aeroplane during take-off and landing. During take-off, close-in obstructions on the runway extended centre-line may cause a pilot to lift off early and climb at an excessive angle, which will aggravate any problem of poor view of obstructions through the windscreen at high pitch angles, which in turn may lead to a further increase in pitch. During landing, high ground or obstructions in the approach area can cause a pilot to adopt a high approach path. Significant obstacles below the runway such as sea walls, creeks or ditches may also cause a pilot to approach at an excessively high angle and then land long. This effect is likely to be worse when the aeroplane has poor forward visibility or is approaching in a flapless configuration. 4.15.13.3 Emergency alighting areas, and climb in the event of engine failure during take-off. Among the characteristics of a good aerodrome for single-engined aeroplanes is an area accessible from the lift-off point to safe manoeuvring height that is suitable for carrying out a forced landing in the event of engine failure after take-off. Similarly, for twin-engined aeroplanes, a good aerodrome will have an obstacle-free, low-angle departure area where an engine failure could be handled with minimum danger of striking ground or obstacles. 4.15.13.4 Light conditions. Pilots should not underestimate the potential difficulty of taking-off or landing directly into a low sun, and should take into account haze, smoke or low light when manoeuvring in the vicinity of an aerodrome or looking for other traffic. In particular, if a take-off or landing into the sun is known to be likely, the pilot should make every effort to ensure the windscreen is clean. 4.15.14 Aeroplane information 4.15.14.1 Aeroplane certification standard. The certification standard specifies what information must be provided in the aircraft flight manual. Most CASR/FAR Part 23 aeroplanes are not designed for fail-safe operation in the same way that large aeroplanes are meant to perform. Each aeroplane type and category has a certification standard, the operational details of which are reflected in the flight manual. 4.15.14.2 Demonstrated landing distance. Part 23 requires the manufacturer of an aeroplane to demonstrate the landing distance required for the aeroplane. The demonstrated landing distance (DLD) is the figure supplied by the manufacturer for the pilot to use as the basis for assessment of landing distance required for a particular landing. Pilots should be aware that the aeroplane manufacturer has a vested interest in publishing the minimum distance obtained in a landing under the conditions permitted for the tests. The requirements for the test are: the aeroplane must approach at not less than 1.3 times the stall speed in the landing configuration; the aeroplane must cross the threshold at not less than 50ft; the test must be done on a dry, sealed surface; and the aerodrome must be within 2% slope up and 2% down. the manufacturer is also at liberty to have the certification done by an experienced test pilot authorised to fly as aggressively as possible short of damaging the aeroplane. It follows that pilots conducting normal operations are highly unlikely to replicate the aeroplane manufacturer's DLD in normal operations, and therefore have a duty of care to apply appropriate safety margins. 4.15.14.3 Performance information. The aircraft's flight manual, owner?s manual, operating handbook or placarding should provide relevant performance information, but presentations are not standardised. Learning how to find and interpret a particular aircraft's performance information should be part of a pilot's familiarisation with the aeroplane. 4.15.14.4 Relevant nomenclature. There are few standard 'V speeds' or suchlike performance notations formally applicable to small aeroplanes. Notwithstanding, a number of notations are frequently used to describe small aeroplane performance parameters. *A list of notations in common usage for large and small aircraft are shown at Annex A Table 4. 4.15.15 Critical operations 4.15.15.1 Land and hold short operations (LAHSO). [Not applicable to ultralight operations] 4.15.15.2 One-way operations. One-way operations are little used in Australia except in some hill-country agricultural operations, usually fertiliser dropping. If the only available landing strip faces into a hill, landings have to take place toward the hill and take-offs made away from the high ground. A problem with many one-way landing strips is that rising ground precludes a go-around beyond some point short of the target threshold. Don't try to use a one-way strip unless you are highly experienced or formally trained, and have recent practice in landing in minimum distance. If a pilot intends to operate off one-way strips, logbook evidence of skill training by an experienced agricultural instructor would be a wise precaution against insurance problems and general liability. Many one-way strips have a steep slope, and even experienced pilots may have difficulty with perspective on final - a normal approach angle is likely to appear too steep, causing the pilot to descend to establish an abnormally shallow approach angle with a high approach speed. If the landing area backs on to high ground the pilot may find him or herself committed to a landing above target threshold speeds and either try for a go-around and fail to outclimb the gradient, or have to proceed with a high-speed landing that results in over-running the (usually short) landing strip. Another major problem for one-way operations is the possibility of adverse winds. Tail winds on a minimum length strip are a recipe for disaster, and turbulent winds in hilly areas can cause severe handling problems even if they are generally in the optimum direction. Appendix A: Dimensional and other guidelines for aircraft landing areas (ALAs) 1. Use of landing areas by large aeroplanes 1.1 For non commercial operations the pilot in command of an aeroplane must ensure that the aeroplane only takes off or lands at aerodromes which have physical characteristics, visual aids, facilities and obstacle limitation surfaces that are adequate for the safe operation of the aeroplane, taking into account whether the operation is to be conducted by day or by night, the weather minima to be used and requirements relating to the aeroplane's performance. 2. Recommended minimum physical characteristics of landing areas and water alighting areas 2.1 Runway Width. For other than agricultural operations, a minimum width of 15 metres is recommended, although experienced pilots can operate aeroplanes with a MTOW less than 2000kg on runways as narrow as 10 metres provided there is little or no cross-wind. If the pilot cannot comfortably straddle the mainwheels across the centreline of a sealed runway for the entire ground-roll, a take-off or landing on a strip less than 20 metre wide should not be attempted. Inexperienced pilots should be very cautious about using a narrow strip, especially if the edges of the strip are rough, soft, or otherwise likely to slew or damage the aeroplane. In addition, each aeroplane type has different handling characteristics that need to be taken into consideration. *For agricultural operations, operating on runways less than 10 metres is not recommended. 2.2 Runway length for landing. A runway length at least equal to that specified in the aeroplane's flight manual (or approved alternative) for the prevailing conditions is generally the minimum acceptable. If an unfactored landing distance (the DLD) is shown in the AFM, the pilot should call for at least 15% additional landing distance. Qualified agricultural pilots may conduct day operations off runways equal to 75% of the runway requirements specified in the aeroplane's flight manual for the prevailing conditions, provided that (at least) the remaining 25% of the nominal runway length is available as clearway, and given that the aeroplane approaches to land over the clearway and takes off toward the clearway. Night agricultural operations should be conducted off runways at least 50% longer than those required for day operations, preferably with additional clearway because obstructions will be unlit. 2.3 Longitudinal Slope. The longitudinal slope between the runway ends should not exceed 2%, except that 2.86% is considered safe on part of the runway so long as the change of slope is gradual. In agricultural operations, runway slope should not exceed 12.5% for day and 2% for night operations. *Where overall slope exceeds 2% the runway should be used only for one-way operations, with landing uphill and take-off downhill. 2.4 Transverse Slope. The transverse slope between the extreme edges of the runway strip should not exceed 2.5% or 12.5% upward slope over the fly-over area. For agricultural day operations, the transverse slope should not be more than 3% over the runway and 5% over the runway strip. 2.5 Wind. The effects of wind cannot be ignored because it dramatically affects the distance required for a landing or take-off. A windsock is the preferred method of determining wind, and is a must if the aerodrome is used frequently. In other cases, consider having a person provide a smoke signal (fire or smoke flare) clear of the approach path near the approach end of the runway. 2.6 Other Physical Characteristics. Both ends of a runway should have approach and take-off areas clear of objects above a 5% slope for day and a 3.3% slope for night operations. If an object or terrain projects above the surveyed angle it must be sufficiently far away to allow an aeroplane taking off to turn before it is reached, and to permit a safe turn onto finals. [Other recommended landing area physical characteristics are shown on the full document] 2.7 Float plane alighting areas. For water operations, a minimum water channel width of 60 metres for day and 90 metres for night operations is recommended. The depth of water over the whole water channel should not be less than 0.3 metres below the hull or floats of the stationary aeroplane when loaded to maximum take-off weight. An additional area, as shown in the following diagrams, provides a protective buffer for the water channel but need not consist of water. Where the additional area consists of water then it should be clear of moving objects, or vessels under way. The centre line of a water channel may be curved, provided that the approach and take-off areas are calculated from the anticipated point of touchdown or lift-off. 3. Conversion table Landing area gradients and splays expressed as a percentage, in accordance with ICAO practice, may be converted into ratios or angles using the following table: Percentage Ratios Degrees 1 1:100 0°34? 2 1:50 1°09? 2.5 1:40 1°26? 2.86 1:35 1°38? 3 1:33.3 1°43? 3.33 1:30 1°55? 5 1:20 2°52? 12.5 1:8 7°08? 20 1:5 11°18? 4. Marking of landing areas 4.1 Where extended operations are expected to be conducted at a landing area, the owner/operator is encouraged to provide markings similar to those found at government and licensed aerodromes. If markings are provided, they should follow the colours and specifications set out in AIP AD. 4.2 Where runway markers are provided which are not flush with the surface, they should be constructed of a material that is not likely to damage an aeroplane. 5. Lighting for night operations [Not applicable to Ultralight Aviation] 6. Other factors that should be considered prior to using a landing area 6.1 A pilot should not use a landing area unless the aeroplane can be kept clear of all persons, animals, vehicles or other obstructions. 6.2 Geographic Location. A landing area should not be located: under an instrument approach area or in such a position that it presents a hazard to aircraft conducting a published instrument approach; within any area where the density of aircraft movements creates a hazard; or where take-off or landing over buildings, noise sensitive livestock or populated areas causes a nuisance or hazard. 6.3 If a proposed landing area is located near a city, town or populous area or any other area where noise or other environmental considerations make aeroplane operations undesirable, the availability of such a landing area may be affected by the provisions of relevant environmental legislation. 7. Measuring an aerodrome 7.1 Distances. The length of a potential runway can be established in a number of ways. Always measure twice, preferably by different methods and round measurements down to ensure that the results are conservative. Common methods of measuring are: pacing: all individuals have their own stride length which can be established by averaging, with about 0.75 metre being a common walking stride that might be used to determine the length of a runway, and 1 metre being a long, deliberate stride that might be used to find the width of a runway; motor: the odometer of a calmly driven vehicle or motorcycle can be used to measure the length of a runway and many clearways; measuring wheel: the best method, with wheels commonly available and used for a variety of land measuring tasks. 7.2 Slope. If the slope is seen as significant it can be measured by farm or surveyor's inclinometer, or the pilot may take altimeter readings at both ends of the runway, convert the altimeter reading to metres and divide the altitude difference by strip length to get slope. For example, if the altimeter reading at each end of a 800m runway differs by 55 ft (16m) the ratio is established at 1:50 by dividing runway length with the height difference (800/16). This equates to a slope of 2% (refer to the conversion table para.3) 8. Surface testing of a landing area 8.1 Rough surfaces. The presence of holes, cracks and ruts will degrade aeroplane performance and handling and increase the possibility of structural damage. Driving a stiffly sprung vehicle along the runway at a speed of at least 75 kph can test the smoothness of a runway. If this does not cause significant discomfort to the occupants of the vehicle, the surface can be considered satisfactory. 8.2 Soft, wet surfaces. A test vehicle should be driven in a zig-zag pattern at a speed not exceeding 15 kph along the full length and width of the runway. Particular attention should be paid to suspect areas with up to three passes over suspect ground. If the vehicle's tyre marks exceed a depth of 25mm the surface is not suitable for any aeroplane which can be reasonably represented by the test vehicle. As a rule, an aeroplane with small wheels or high tyre pressures will require harder runways. If recent heavy rain or other waterlogging has occurred, the ground should be tested with a crowbar in several places along the runway to ensure that a dry surface crust does not conceal a wet base. 9. Factors and notations 9.1 After establishing the aeroplane's runway requirements in the prevailing density altitude and wind conditions by consulting the AFM it is recommended that the unfactored (DLD) runway length required by the AFM be multiplied by a safety factor related to MTOW of the aeroplane. The standard factors shown in Table 1 are recommended for private operations in small aeroplanes: Table 1: Standard factors for take-off: for all MTOWs 1.15 or 115% Standard factors for landing: up to 2000kg MTOW 1.15 or 115% 9.3 After factoring the DLD in accordance with Table 1 the pilot should apply further factors in accordance with any guidance given in the AFM. If the AFM is not helpful, consider applying any of the allowances shown in Tables 2 and 3 below that are relevant to the flight. Table 2: Contingency take-off allowances - approximate increase in take-off distance to 50 ft Circumstance Factor Multiple per 10% increase in aeroplane weight 20% 1.2 an increase of 1000 ft in airfield altitude 10% 1.1 an increase of 10C in ambient temperature 10% 1.1 dry grass* up to 20 cm (on firm soil) 20% 1.2 wet grass** up to 20 cm (on firm soil) 30% 1.3 2% uphill slope* 10% 1.1 a tailwind component = 10% of lift-off speed 20% 1.2 soft ground or snow* 25%+ 1.25 + (These effects are additive) * Effects are variable and possibly unpredictable. Expect a ground distance increase, but airborne distance remains the same ** If wet grass is on soft ground the effect on rolling resistance is cumulative, so both elements must be considered. Table 3: Contingency landing allowances - approximate increase in landing distance from 50 ft Circumstance Factor Multiple a 10% increase in aeroplane weight 10% 1.1 an increase of 1000 ft in airfield altitude 5% 1.05 an increase of 10C in ambient temperature 5% 1.05 dry grass* - up to 20 cm (on firm soil) 20%+ 1.2 wet grass* - up to 20 cm (on firm soil) 30%+ 1.3 short and dense or very green grass* 60% 1.6 2% downhill slope 10% 1.1 tailwind component, per 10% of landing speed** 20% 1.2 light snow or surface muddiness 25%+ 1.25+ 20-50mm standing water* 50%+ 1.5+ (These effects are additive) * Effects are variable and possibly unpredictable. While rolling resistance is increased, reduced braking effectiveness has the greater effect. Airborne distance remains the same but expect an increase in ground distance. ** In factoring the effect of wind, reduce estimated headwind by 50% and assume that a tailwind is 50% greater than the estimate. Table 4: Standard notations (the 'V-speeds') The following informal list of notations are typical of those used in depicting performance and handling values for various aircraft (most relate to large aircraft): Vf - design flap speed Vfe - maximum flap extended speed Vh - maximum speed in level flight with maximum continuous power Vne - never-exceed speed Vno - maximum structural cruising speed Vle - maximum landing gear extended speed Vlo - maximum landing gear operating speed Vs - stalling speed, or the minimum steady flight speed at which the aeroplane is controllable Vs15 - (example) stalling speed with 15 deg flap Vso - stalling speed or minimum steady flight speed in the landing configuration Vs1 - stalling speed or minimum steady flight speed in a specific configuration Vs1g - stalling speed for a force equalling 1g Vmu - minimum unstick speed Vr - rotation speed Vlof - maximum lift-off (unstick) speed Vtoss - take-off safety speed, being a speed at which adequate control is available in the event of a sudden and complete failure of the critical engine during climb after take-off Vx - speed for best angle of climb Vy - speed for best rate of climb Vfr - flap retraction safety speed Vfc - final climb speed (take-off) Va - design manoeuvring speed Vb - design speed for maximum gust intensity Vc - design cruise speed Vd - design diving speed Vdf/Mdf - demonstrated diving speed Vmcl - minimum control speed for landing approach, all engines operating Vat - target threshold speed Vmt - minimum threshold speed Vtmax - maximum speed at the landing threshold Vat1 - target threshold speed, one engine out Vtd - touch-down speed Vp - aquaplaning speed STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
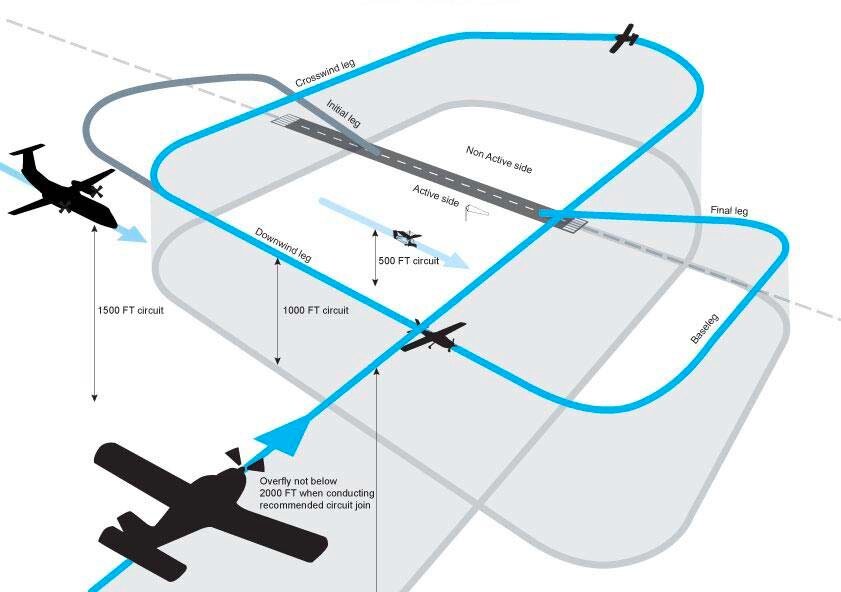
Please note this is an html version of a draft CASA Advisory Circular AC 91-220(0) initially circulated September 2001; all the CASR 91 series regulations referred to are still not enabled, thus this document has not progessed beyond draft status and furthermore, at July 2014, the CASR 91 series regulations are not applicable to RA-Aus aircraft operations. However, the Civil Aviation Safety Authority have produced two civil aviation advisory publications applicable to RA-Aus aircraft operations that recommend particular procedures and provide guidance on a code of conduct to allow greater flexibility for pilots when flying at, or in the vicinity of, non-controlled aerodromes. These Civil Aviation Advisory Publications (available on this website) are: CAAP 166-1 'Operations in the vicinity of non-controlled aerodromes' (August 2014) and CAAP 166-2 'Pilots responsibility for collision avoidance in the vicinity of non-controlled aerodromes using 'see and avoid' (December 2013). 4.14.1 REFERENCES CASR 91.185 Basic rule "see and avoid" CASR 91.190 Operating near other aircraft CASR 91.195 Giving right of way CASR 91.200 Who has right of way CASR 91.205 How to give right of way CASR 91.210 How to overtake in flight CASR 91.215 Right of way rules aircraft on the ground or water CASR 91.220 Operating on or in vicinity of non-controlled aerodromes CASR 91.225 Safety during take-off and landing 4.14.2 PURPOSE This Advisory Circular (AC) provides information to enhance the safety of flight at aerodromes and landing places which do not have an aerodrome traffic control (tower) service in operation. It is intended to give an overview of pilot responsibilities and highlight principles applicable to all pilots who operate at non-controlled aerodromes. 4.14.3 STATUS OF THIS AC This AC is the first that has been issued on this subject. Advisory Circulars are intended to provide recommendations and guidance to illustrate a means but not necessarily the only means of complying with the Regulations, or to explain certain regulatory requirements by providing interpretative and explanatory material. Where an AC is referred to in a `Note' below the regulation, the AC remains as guidance material. ACs should always be read in conjunction with the referenced regulations. 4.14.4 DEFINITION Non-controlled aerodrome means an aerodrome where there is no aerodrome control facility in operation at the time of a particular take-off or landing. 4.14.5 INTRODUCTION 1. CASR 91.220 states the minimum legal requirements for operation of an aircraft at an aerodrome which does not have an aerodrome traffic control service. The regulation requires all pilots to conduct their operations in accordance with standard procedures. The objective is to ensure that each pilot in the vicinity of an aerodrome is aware of any other traffic at the aerodrome, knows the position and intentions of other pilots and can participate in an orderly flow of traffic. 2. Safe operations have been conducted at non-controlled aerodromes for the best part of 100 years, but the basic requirements have always been the same. Non-controlled aerodrome operations work well up to moderate levels of traffic if pilots know the characteristics of the aerodrome, inform themselves well, say what they are doing, keep a good look out and use standard procedures. 3. CASR 91.220 imposes a series of common-sense obligations on pilots, the aim of which is to ensure that any hazard associated with non-controlled operations is reduced to the minimum, consistent with the way operations at non-controlled aerodromes are actually conducted by sound pilots. 4. ATC may be placed into operation at an aerodrome without a tower for special purposes such as an air display, disaster relief or other significant event. Times of operation of a tower facility are promulgated in ERSA, and imposition of tower-type aerodrome control at other places will be notified by NOTAM. 4.14.6 OPERATIONS IN VMC 1. Factors. The principal factors or elements relating to operations in VMC are: The type of operation, i.e., agricultural, pilot training, air transport etc; Type of aircraft; Wind speed and direction; Number of runways; Obstructions and topography in the vicinity of the aerodrome; Built up areas and local noise sensitivity; Number of aircraft; Other activities, i.e., parachuting, glider flying, flight training; Whether all aircraft are radio-equipped; and Proximity of controlled airspace and low-level operations; Non-communicating traffic; and Non-compliant traffic. 2. Operational needs and manoeuvres.There can be varied operational needs and manoeuvres conducted at a non-controlled aerodrome: Skilled pilots will often want to make smaller circuits than pilots under training or with low recency; Larger air transport aircraft are expensive to run, and minutes saved make straight-in approaches an attractive proposition; Helicopters are not restricted to normal circuit patterns and generally operate to stay clear of fix-wing circuit patterns; Pilots doing actual or practice instrument approaches will often make straight-in or abbreviated approaches to a landing or to a missed approach point on an instrument runway, or will elect to join the circuit from overhead a navigation aid via the most convenient turn to the runway in use; Agricultural pilots conducting local deliveries may prefer to do a contra or a low-level circuit, or make straight-in approaches on a cross runway (expect any legitimate manoeuvre that will speed up delivery rates); Parachuting and glider tug aircraft may make steep descents into the circuit area; Ultralight pilots generally prefer to make low, small circuits, and to overfly terrain with potential for a safe forced landing; Gliders require winching or towing, often use parallel runways and/or contra circuits, and are committed to land from the time they enter the circuit; and Trainee pilots require relatively large circuits, don't have reserve capacity to cope with unusual manoeuvres by other aircraft , and can easily be forced to abandon their preferred flight path by other aircraft, including those on normal manoeuvres. 3. Safety rules permitting, the pilots of each type of aircraft will want to fly the circuit pattern most suited to the aircraft and the type of operation. Pilots have to give and take relevant information and exercise tolerance and consideration if varied circuit flight paths and experience levels are to be accommodated safely. 4. Wind, and pattern conflicts. Wind direction is generally more critical to smaller aircraft, hence the common provision of a small secondary runway. If a strong wind favours a short runway the circuit pattern may be complicated because small aircraft will use the short runway while larger aircraft may be forced to use a longer, out-of-wind runway. Light winds can make for a difficult traffic situation because pilots are not provided with a cue to use a particular runway, and will prefer to use the runway which is most suited to their operation. Where wind direction is not available from other sources, incoming aircraft may have to overfly the aerodrome to see a windsock, and may enter the traffic pattern in conflict with preceding aircraft. A difficult situation can arise when an aircraft is established on final leg in conflict with another aircraft taking off in the opposite direction. 5. Obstructions, topography, local noise sensitivity and adjacent CTA. Topography and obstructions (transmitting towers, smokestacks and so forth) may restrict circling in some parts of the circuit area, and built-up areas, hospitals, noise sensitive livestock or the like may require modification of normal traffic patterns or dictate close or wide circuits. Adjacent CTA must be avoided unless clearance to enter is obtained. Pilots must make themselves aware of any pattern variations peculiar to an aerodrome prior to operating at that aerodrome. 6. Number of aircraft, activities and communications. The greater the number of aircraft and the more varied the activities the harder it is for pilots to keep track of other traffic. Each pilot must be on the lookout for no-radio aircraft, ultralight aircraft, helicopters, aircraft on crosswind training, aircraft on straight-in approaches and aircraft operating contrary to the recommended circuit direction. Ready communication with other aircraft is vital, but if the traffic level is too high for self-arranged separation each pilot may have to resort to "unalerted see and avoid" techniques. In this situation each pilot should self-announce and try to keep track of other traffic by listening to other broadcasts of aircraft type, position and intention, simultaneously looking out for unannounced traffic. If there is too much traffic for a satisfactory level of safety, comply with the recommended circuit direction, announce your position and intentions, adopt alerted see-and-avoid practices and either land or vacate the circuit as soon as possible. 7. Gathering information. Before operating at any aerodrome, listen out, if possible, on the relevant CTAF or UNICOM frequency. Establish that the correct frequency is selected and use UNICOM, ATIS, AWS and/or other traffic to establish the pressure and wind direction, traffic numbers, traffic type and the runway in use. Maintain a good lookout while using radio to arrange self-separation; bearing in mind that excessive RT will decrease safety. Announcements made shortly before committing to particular manoeuvres such as entering a runway, taking off, entering downwind, or turning base give other pilots at the aerodrome a chance to adjust or arrange separation. 8. Circuit protocols. All pilots should develop the following habits: Carry radio, and use it appropriately; Make turns in the direction recommended for the aerodrome unless there is good reason to do otherwise, bearing in mind that there may be a left-hand pattern when landing in one direction and a right-hand pattern when landing in the opposite direction; Comply with CASR 91.220 (2) if making a turn contrary to the normal direction for the runway; Observe the right-of way rules (CASR 91.195, 91.200, 91.205, 91.210 and 91.215 ); Turn at a safe height and speed after take-off, (ideally, not less than 500ft AGL); Aim to line up above 500ft AGL for landing, and at least 1000m before the threshold; (g) where it is reasonable to do so, follow the approximate flight path of preceding aircraft (beware wake turbulence - never approach below the flight path of any large aircraft). If unable to follow, maintain your position in the sequence and broadcast your intentions if this will require an unusual shape or size to the circuit; If possible follow the convention that most fixed-wing aircraft fly the circuit at 1000ft AGL, helicopters at 800ft AGL and jet aircraft at 1500ft AGL; If overflying to review the windsock and signal square, do so at 1500ft AGL, (bearing in mind the possibility of jet or instrument practise aircraft at that height). Be proactive, do not remain silent because other aircraft are broadcasting. Let them know you are there. In 2010 CASA produced two advisory publications to support CTAF procedures and provide guidance on a code of conduct to allow greater flexibility for pilots when flying at, or in the vicinity of, non-towered aerodromes. These Civil Aviation Advisory Publications (available on this website) are: CAAP 166-1 'Operations in the vicinity of non-towered (non-controlled) aerodromes' and CAAP 166-2 'Pilots responsibility in collision avoidance in the vicinity of non-towered (non-controlled) aerodromes by 'see and avoid'. Note that the 'ultralight' term used in the CAAPs when recommending a 500 feet circuit height, refers only to those RA-Aus aircraft which have a normal cruising speed below 55 knots, or thereabouts. There are some variations in the advice given in the CAAPs and in this document. The CAAPs are the superior publications. CASA has produced an online interactive learning tool titled 'Operations at, or in the vicinity of, non-controlled aerodromes' which is now available at CASA online learning. 4.14.7 OPERATIONS IN IMC OR MARGINAL CONDITIONS 1. IMC. If the weather is below VMC all aircraft must carry radio and proceed on the basis of a professional level of broadcasting, although the possibility of a pilot operating full broadcast but on the wrong frequency remains. Visual circuit practice should not be done in weather below VMC, even if the ceiling is above the circling minima. In reduced visibility it is especially important that pilots display anti-collision lights and navigation lights in compliance with CASR 91.590. 2. Marginal conditions.There is a possibility that the pilot of an IFR aircraft may assume there will be no VMC traffic, and there a possibility that a VFR pilot may be airborne in conditions too demanding for his or her skills. A saving grace is that traffic numbers are usually fairly low, but there is a need to know what other IFR and VFR aircraft (the latter possibly without radio) are doing and to remain alert. Full use of radio, sight and lights is needed. A pilot should avoid doing repetitive circuits in marginal conditions, and should not fly as PIC if his or her skill levels are not enough to be comfortable in the prevailing conditions. 4.14.8 STRAIGHT-IN APPROACHES 1. In an endeavour to align expectations and lookout with what often happens in real life regardless of rules, the regulation for operations at non-controlled aerodromes does not limit straight-in approaches to certain classes of aircraft. Pilots must be on the lookout for aircraft on straight-in approaches, and any pilot who does a straight-in approach must exercise sound airmanship and observe the relevant rules 2. CASR 91.200 requires that, in the event of conflict between a powered aircraft on base leg and a similar aircraft on finals, the lower of the two aeroplanes has the right of way, subject to the courtesy expressed in the rule. 4.14.9 CIRCUIT DIRECTION The regulations permit turns contrary to the recommended circuit direction subject to supplementary safety procedures. Left-hand circuits should be performed unless right-hand circuits are recommended for the particular runway, but the pilot may use a contrary direction if it is safe to do so (CASR 91.220 (2)). In assessing the safety of a contrary turn the pilot should take into account, among other things, the prevailing visibility, the probable expectations of other pilots, and the possibility of a missed broadcast. 4.14.10 PARACHUTING AND GLIDING 1. Parachuting. Parachuting operations are conducted at many non-controlled aerodromes used by a variety of traffic. Protocols developed by CASA and parachuting organisations are expressed in the AIP. Some of the main elements are: Parachutists are not authorised to drop through cloud, but are not subject to the rules of VMC and may drop through gaps in cloud. The dropping pilot must broadcast intentions to drop 2 minutes prior to the planned exit, and must not drop if there is evidence of conflicting traffic. All aircraft except balloons must give way to descending parachutists. Parachutists will not exit within 15 minutes of the ETA of a scheduled passenger transport flight unless the drop pilot can be sure that all parachutists will have landed before the passenger aircraft has entered the circling area. Similarly, parachutists will not be dropped until a departing scheduled passenger aircraft is clear of the circling area. Parachutists' pilots will listen out on both the CTAF and area frequency, and will give an additional call 4 minutes prior to the planned exit. Parachutists will avoid conflicting with traffic on the live side of a licensed aerodrome (assuming a live side is defined by aerodrome traffic), nor will they intentionally land on any runway, taxiway or apron. Parachutists will not be dropped if another aircraft is conducting an instrument approach, or is expected to commence an instrument approach within 5 minutes after the planned drop. If the drop zone is on the aerodrome, the drop aircraft will usually proceed to a drop point upwind from the aerodrome, the distance being proportional to the wind strength and the release height. The exit point can be up to 4 miles upwind, which may at first appear to be clear of the circuit area. 2. Gliding operations.Gliding operations are also conducted at many non-controlled aerodromes used by a variety of traffic. Gliders may also overfly or land at aerodromes where no gliding operation is established. Again, protocols developed by CASA and the gliding movement are expressed in the AIP. Some of the main elements of this are: Gliders may be launched by aerotow, ground-based winch or car tow, or may be self-launching. Aerotow and wire launches may involve releases up to 4000 feet AGL. Overflying an active wire-launching site below 2000 feet AGL is not advisable. Pilots must be aware that if a launch cable breaks during a launch it may lie across a runway until cleared. Tug aeroplanes, winches and tow-cars will normally be radio-equipped. Gliders must monitor and broadcast on the CTAF if there is a scheduled service at the aerodrome. Elsewhere they must monitor the CTAF if they are fitted with radio capable of using the appropriate frequency. At locations where contra-circuits are notified in AIP ERSA there is no dead side to the circuit and all operations below 1500 feet AGL should remain on their own side of the runway. Traffic should join circuit on either an upwind leg over the runway, or a downwind leg. Any glider which thermals within 2nm/below 1500 feet AGL of the downwind end of the runway in use must be fitted with VHF and must monitor the CTAF, and are not permitted to interfere with other circuit traffic. Powered aircraft must give way to gliders, as gliders are committed to landing once established in the circuit, and may need to return to land if a launch is aborted or if sinking air is encountered after launch. Gliders, more so than powered aircraft, may need to vary their circuit patterns and fly a non-standard pattern during landing manoeuvres. 4.14.11 FURTHER READING Refer to the AIP or the VFR Flight Guide for further advice about circuit operations, particularly operations at aerodromes and landing places that have special characteristics or which support parachuting, gliding, military, aerobatics or various training operations. AIP ERSA contains the details of specific aerodromes and landing places. AIP AD contains technical information about aerodrome construction, marking and facilities. AIP NOTAMs are used to provide notice of events and changes. AIP Supplements and Aeronautical Information Circulars (AIC) are used respectively to convey details about special operations and procedure updates prior to their appearance in the AIP proper. 4.14.12 SUMMARY The need for sound airmanship is at its greatest at a busy non-controlled aerodrome, where all pilots must obtain and use all relevant information, observe the rules, use radio and lights where possible, maintain the best of lookouts, and practice patience and courtesy. Assistant Director Aviation Safety Standards STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
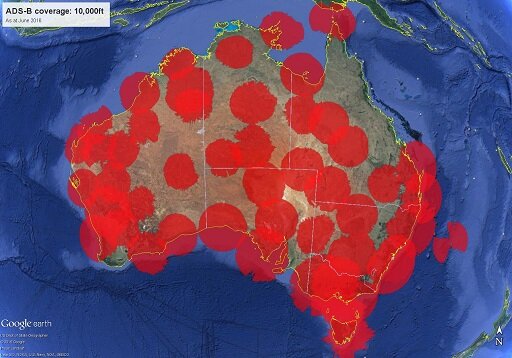
4.13.1 ADS-B navigation and surveillance technology system The concept The concept for the full ADS-B system is that all, or most, airborne aircraft operating in Class A, B, C and E airspace and Class G above 10 000 feet, automatically and continually (i.e. once or twice per second) squitter (i.e. broadcast automatically) several digital data packets which together contain the aircraft's ICAO 24-bit Aircraft Address code (the unique airframe identification assigned by CASA), flight identification (aircraft call sign), GNSS-derived position – latitude and longitude plus the integrity/accuracy of that position, its three-dimensional velocity; i.e. rate of climb/descent, direction in azimuth and speed. Pressure (or Mode C) altitude is provided by an altitude encoding device. The data packets broadcast from aircraft are received by Airservices Australia [AsA] ground stations, which feed the data to air traffic management [ATM] systems, providing more precise tracking than primary or secondary surveillance radars. The broadcast ADS-B packets are also received by all aircraft equipped with an ADS-B data receiver that are within range. The derived data provides a real-time cockpit display of traffic information, similar to the ground ATC systems except that the traffic is shown in relation to the receiving aircraft's intended track. The Mode S transponder, currently used for response to AsA's secondary surveillance radar network and as the standard air-air datalink in Traffic Collision Avoidance Systems [TCAS], is also used in ADS-B — with some enhancements. ADS-B broadcasts are via a ModeS1090 MHz Extended Squitter [1090ES] transponder link. The term 'extended squitter' refers to the additional [112-bit] ADS-B data packet, which is part of the enhanced Mode S transponder data link standards for ADS-B. However ADS-B, even in full operation, will not alter the VFR pilot's responsibility to 'see and avoid' other aircraft; ADS-B is seen as an aid to visual acquisition for VFR operations. Airborne avionics To achieve the full Airservices Australia ADS-B system concept, the onboard ADS-B avionics for general aviation and recreational aviation aircraft would have to include several functions or modes: data transmission. This provides data broadcast capability so that positional data, provided by a GNSS system plus an altitude encoding altimeter or a pressure altitude blind encoder, is continually broadcast. This is known as 'ADS-BOUT' capability and all aircraft ADS-B units must have this minimum function. The accuracy of the broadcast data is dependent on the positional/navigational data from the GNSS receiver which, in turn, is dependent on the availability of signals and the capability of the GNSS. A high-performance TSO* GNSS receiver is part of the system. *Note: a 'TSO' or technical standard order is a 'minimum performance standard issued by the FAA (CASA issues ATSOs) for specified materials, parts, processes, and appliances used on civil aircraft' data reception. This refers to the capability to receive all data packets broadcast by all ADS-B OUT units within an appropriate range data processing. This entails using the received data to provide a real-time plot of own and other aircrafts' tracks, speeds and altitudes; it is also known as a 'cockpit display of traffic information' [CDTI]. Data reception plus CDTI is known as 'ADS-B IN' capability, providing a pilot's own airborne surveillance and traffic alerting system — much the same as air traffic controllers may be viewing on their displays but completely independent of ATC. There are no regulatory proposals requiring use of ADS-B IN by any sector of aviation but it is the function that could benefit VFR recreational pilots, through enhanced situation awareness information. ADS-B implementation in the USA The United States Federal Aviation Administration [FAA] has decided that ADS-B transmission/data links in the USA will be via the Mode S 1090 MHz Extended Squitter [1090ES] surveillance link for aircraft that may operate above FL 180. For aircraft that only operate below FL 180 FAA has specified a Universal Access Transceiver [UAT] surveillance link using 978 MHz rather than use the 1090ES; mainly in order to reduce congestion on 1090 MHz. UAT is a bi-directional data link system developed in the USA, specifically for ADS-B operation, so that aircraft with a UAT transceiver can also receive the freely available Flight Information Service data broadcasts [FIS-B] from the FAA-maintained 978 MHz uplink network, in addition to an ADS-B traffic information service broadcast TIS-B]. FIS-B includes METARs, TAFs, SPECI, winds and temperatures aloft, pilot reports [PIREPs], restricted area status and NOTAMs. In Australia similar products are available in flight from Airservices NAIPS Internet Service via mobile broadband, see EFB software suppliers. The FIS-B facility also provides an animated NEXRAD weather radar service — similar to the Australian BoM weather radar network. UAT is not a transponder so UAT equipped aircraft also need a Mode S transponder for the secondary surveillance radars. Garmin released their portable GDL 39 ADS-B 1090 MHz/978 Mhz receiver and GPS receiver in June 2012, it can connect via Bluetooth to an iPad or iPhone to display the traffic information on a moving map. See the user guide. This particular device is built for the United State's ADS-B dual frequency environment and has no application in Australia. The current (November 2012) selling price in the US is $800. 4.13.2 The Australian ADS-B implementation program AsA has opted for 1090ES for all aircraft, except those operating under the VFR in Class D airspace or in Class G below 10 000 feet. CASA amended CAO 20-18 December 20, 2012 to reflect the following ADS-B OUT implementation time table: 9B Directions relating to carriage and use of automatic dependent surveillance — broadcast equipment 9B.8 On and after 12 December 2013, any aircraft that is operated at or above FL290 must carry serviceable ADS-B transmitting equipment that complies with an approved equipment configuration by meeting the conditions for approval set out in Appendix XI. 9B.9 An aircraft: (a) that is first registered on or after 6 February 2014; and (b) that is operated under the IFR; must carry serviceable ADS-B transmitting equipment that complies with an approved equipment configuration by meeting the conditions for approval set out in Appendix XI. 9B.10 On and after 2 February 2017, an aircraft: (a) that is first registered before 6 February 2014; and (b) that is operated under the IFR; must carry serviceable ADS-B transmitting equipment that complies with an approved equipment configuration by meeting the conditions for approval set out in Appendix XI. 9B.11 On and after 4 February 2016, an aircraft that is operated under the IFR in airspace: (a) that is Class A, B, C or E; and (b) that is within the arc of a circle that starts 500 NM true north from Perth aerodrome and finishes 500 NM true east from Perth aerodrome; must carry serviceable ADS-B transmitting equipment that complies with an approved equipment configuration by meeting the conditions for approval set out in Appendix XI. None of the above ADS-B OUT legislation affects aircraft registered with a Recreational Aviation Administration Organisation as no RAAO aircraft may operate under the IFR or will operate in class A or above FL 285 except perhaps an officially sanctioned altitude attempt. However, the following subsection of CAO 20-18 will apply from 6 February 2014 to any newly registered RA-Aus aircraft operating under the VFR that chooses to operate in any controlled airspace other than Class D. Such aircraft must be fitted with a serviceable Mode S transponder that meets the Australian standards for 1090 ES transponder equipment and for ADS-B OUT capability. This may create problems with imported aircraft. 9E Carriage of Mode S transponder equipment 9E.1 This subsection applies to an aircraft engaged in private, aerial work, charter or RPT operations. 9E.2 Subject to paragraph 9E.3, an aircraft: (a) that is: (i) first registered on or after 6 February 2014; or (ii) modified by having its transponder installation replaced on or after 6 February 2014; and (b) that is operated: (i) in Class A, B, C or E airspace; or (ii) above 10 000 feet amsl in Class G airspace; must carry a serviceable Mode S transponder that meets the standards: (c) for Mode S transponder equipment — in subsection 9C; and (d) for ADS-B transmission — in a clause or clauses of Appendix XI as follows: (i) clauses 2 and 5 of Part B; or (ii) clause 7 of Part C; or (iii) clause 8 of Part C. Note: The requirement is for aircraft to be fitted with a Mode S transponder with ADS-B OUT capability. That does not mean that ADS-B OUT transmission is also required under this paragraph. It means that, with the later connection of compatible GNSS position source equipment, ADS-B OUT can be transmitted as well as Mode S SSR responses. Note: Paragraph 9E.2 does not apply to an aircraft operating in Class E airspace or above 10 000 feet in Class G airspace, if the aircraft does not have an engine or sufficient engine-driven electrical power generation capacity to power a Mode S transponder. Otherwise, Australian sport and recreational aircraft that always operate under the visual flight rules below 10 000 feet and outside Class A, B, C and E airspace (the Class D areas are excluded) will not be affected by the ADS-B surveillance and separation service implementation, at least not before 2020. 4.13.3 Airservices Australia's ADS-B system The upper airspace program AsA states that ADS-B "is an air traffic surveillance technology that enables aircraft to be accurately tracked by air traffic controllers and other pilots without the need for conventional radar." To date AsA has deployed 58 ADS-B ground stations at 29 sites across Australia which, combined with SSR, provide ATC surveillance capability over the entire continent above FL290 (29 000 feet ISA). These first 29 locations established an Australian ADS-B network for ground-based air traffic management; i.e. an ADS-B OUT system. These stations, each with a range up to 200 nm, are co-located at existing VHF communication relay sites and linked to surveillance displays at ATC centres, which allows Airservices Australia to provide an SSR-like traffic separation service across the current non-radar airways above FL290. Of course these same ground stations also have the capacity for air traffic management at altitudes below 10 000 feet — but reduced range, being line-of-sight dependent. Currently in Australia the main Mode S transponder function is to allow aircraft equipped with Traffic Alert and Collision Avoidance Systems to 'talk' directly with each other, thereby enabling mutual resolution of potential traffic conflicts. Mode S can also provide faster, more accurate ATC surveillance, provided the ground radars are of the fast single pulse interrogation type such as those Mode S Terminal Area Radar equipment with solid-state primary surveillance radar and Mode A/C and S capable SSR systems at Coolangatta, Melbourne, Adelaide, Sydney, Cairns, Brisbane, Canberra, Darwin and Perth. The ADS-B OUT function is accomplished by upgrading an aircraft's existing Mode S transponder to 1090ES, and linking the GNSS system and the transponder. The upper air space program doesn't affect recreational aviation. The lower airspace program "A major, longer term program designed to make ADS-B the primary means of ground to air and air to air surveillance in Australian enroute airspace. Includes installation of additional ADS-B ground stations to provide air traffic surveillance in airspace currently covered by enroute radar facilities. Intended to lead to the eventual decommissioning of a number of radar sites." Initially an accelerated introduction of ADS-B surveillance into lower airspace was planned, but in the last quarter of 2008 it was agreed that a more gradual transition to satellite-based systems, harmonised with the North American and European transition plans, would be wiser. Airservices Australia is "proceeding with the replacement of its enroute radars and navaids as necessary to ensure the integrity of Australia's air traffic control system." (CASA's Notice of Final Rule Making – Transition to Satellite Technology for Navigation and Surveillance). "The timing and scope of future steps will be progressed through normal regulatory processes and will take into account ... outcomes of the Government's Aviation Policy Green Paper consultation." The Australian land area is about 7.5 million km² and the airspace included from ground level to 10 000 feet agl is about 21 million km³. Probably no more than 10% of the 15 000 registered aircraft are airborne at any time. So it is not surprising that the Australian history of recreational day VFR aircraft 'mid-airs' or 'near-misses' appears to be confined to the circuit area, to aircraft flying formation or to gliders sharing a lift source. Certainly a CDTI traffic display will alert a pilot in the vicinity of an airfield to the direction to look to avoid collision with another aircraft but the likelihood of collision with the ground in a stall/spin incident would seem to be increased if the VFR pilot's eyes remain in the cockpit checking a CDTI traffic display. CASA have published a 28 page ADS-B booklet dated November 12, 2012. There is also an 18 page Frequently Asked Questions pdf document. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
The Global Navigation Satellite System (GNSS) technology, combined with a current, accurate and approved aeronautical database, normally provide excellent position-fixing capability — and continuing 'heading-to-steer' capability, when associated with a stored flight plan. GNSS is classified as a supplemental-means VFR navigation system. However, contrary to good sense, some sport and recreational pilots (and others) do rely on GNSS receivers — plus electronic on-screen position tracking (e.g. a moving map display with own aircraft position centred) — as a primary-means navigation system. Electronic flight planning software has been available for many years but the concept of the sport and recreational aviation 'electronic flight bag' (EFB) is introduced when a tablet computer with inbuilt or external GNSS reception is used for flight planning plus storage of — and inflight reference to — documents such as the following: aircraft flight manual/pilot's operating handbook; ERSA and the AIP book; ARFORs and NOTAMs; and georeferenced Airservices Australia digital aeronautical charts. The EFB is perceived as an aid to situational awareness and is not a CASA approved navigation system. So, electronic flight planning and electronic VFR situational awareness aids are becoming the norm for many recreational pilots, not least because of the availability of: powerful, reasonably priced, reliable, general purpose, portable touchscreen tablet computers, with inbuilt and/or external GNSS connectability, though perhaps not so easy to operate in normal flight conditions in very light aircraft — recognised in aviation as a 'portable electronic device' [PED] smartphones and very fast broadband 3G/4G-LTE cellular mobile telephony networks expanding around Australia plus the availability of WiFi area networks and Bluetooth personal area device interconnection and data transfer, all facilitating surface and inflight access to SIGMETs, BoM weather radar, lightning trackers and other information aids to situational awareness. But the cellular mobile communication services class licence does not authorise the use of any mobile communication device in an airborne aircraft unless in an airliner equipped with a 'pico cell' unit operating under a public telecommunications service licence high quality operating systems and inexpensive iOS/Android/Windows application software packages readily available to all via the internet and the NAIPS Internet Service multi-function, computerised, subscription-free, aeronautical information system provided by Airservices Australia and the Australian Bureau of Meteorology. The current situation enables any reasonably computer adept person to put together a system of software, GNSS aviation receivers, general purpose (rather than aviation-oriented) hardware and navigation databases tailored to their particular aviation needs. All accomplished in accordance with the civil aviation advisory publication CAAP 233-1(1) and at rather low cost — if well researched and done carefully. CAAP 233-1(1) 'provides information and guidance in the use of portable Electronic Flight Bags as a replacement* for paper in the flight compartment'. *Though it is still prudent to carry back-up paper charts. 4.12.1 Navigation system performance criteria There are four parameters for assessing the performance of a navigation system: integrity, accuracy, availability/vulnerability and continuity of service. Integrity refers to the trustworthiness of the device, i.e. user assurance that the data being provided by the device/s meets specified standards and that the system will alert the user when it is not meeting those specified standards. For example, any GNSS system that fails to immediately and adequately inform the pilot when it enters 'dead reckoning' mode certainly does not meet the integrity standard. If a particular system is demonstrated to satisfy all four parameters for a flight phase then it may be classified as a sole-means navigation system — for that phase and thus require no back-up navigation system. When operating under the day visual flight rules, en route navigation by map reading and visual reference to the ground satisfies all four parameters and is the only sole-means system available to RA-Aus aircraft. If a system meets the integrity and accuracy requirements all the time, but falls short on availability/vulnerability or continuity of service, it may be approved as a primary-means navigation system for a flight phase, if specified procedures are employed. Day VFR navigation does not use primary-means systems, only the sole-means system plus supplemental-means systems as required. A supplemental-means navigation system may only be used in conjunction with a sole-means navigation system, but it must meet the integrity and accuracy requirements. Pilots operating under the VFR may use GNSS to supplement map reading and other visual reference en route navigation techniques. Any GNSS receiver may be used but if it is an installed receiver (i.e. not portable) it must be fitted in accordance with CAAP 35-1 or AC21-36; see AIP GEN 1.5 section 8.5.4. GNSS is only officially regarded as a primary-means night VFR navigation if the GPS/Glonass receiver system accords with the FAA's Technical Standard Order [TSO] C129 or TSO C145/6 series, or has other CASA approval. The GPS/GLONASS receiver may supply position data to a portable electronic device as part of a supplemental-means navigation system. For more information concerning the use of GNSS in VFR navigation see AIP ENR 1.1 paragraphs 19.2 and 19.5. Note the wording of sections 19.2.1e and 19.5.1d together with the latter's link to AIP GEN 1.5 section 8. Also see the CASA document 'Instructions — use of GNSS' that came into effect 1 November 2012. 4.12.2 Digitised aeronautical charts VFR charts available Airservices Australia's WAC, VNC, VTC, ERC-L and PCA charts are also available in geoTIFF* digital format redistributed by a few Australian flight planning software producers. These organisations have entered a Standard Data Licence Agreement with Airservices Australia [AsA] enabling the inclusion of AsA map packs as part of their software package; such agreements limit the usable life of each chart within the application. AsA will not sell digital charts direct to the general public and since Maptrax Australia withdrew from that market sector there are no retailers of AsA VFR charts [at March 2013] except for those included in an EFB package for iPad systems. *Note: geoTIFF = geographic tagged image file format with the necessary georeferencing data embedded as metadata. Considering that most Australian controlled airspace below 8500 feet amsl is within 100 nm or so of the coast-line then, outside that coastal fringe, there is little VNC/VTC coverage and the 1:1 million scale of the WACs is a rather small scale for light aircraft pilotage — the sectional charts that are the standard United States VFR navigation chart are 1:500 000. The NATMAP 250K series are 1:250 000 transverse Mercator projection charts (with an optional latitude/longitude graticule) providing a good, larger-scale navigation solution for much of Australia, even though they contain no aeronautical data. As with a paper map, digitisation and the necessary software allows the user to mark up the chart with any information considered pertinent, even allowing permanent storage of several versions of the one chart, and the NATMAP 250K series are valid for several years. Airservices Australia's Visual Terminal Charts are based on the NATMAP 250K series with a latitude/longitude graticule. The digitised NATMAP 250K series may be purchased from Geoscience Australia. The 513 maps of the series are available on DVD, in .ecw format, for about $100 which is less than 3% of the cost of the paper series and well worth having as home reference material — even if you don't use them in flight. Raster and vector maps A raster map or image (such as that from a digital camera or a scanner) is a matrix or grid of rows and columns, each cell being a picture element (pixel) with a discrete colour value — a bit-mapped image — a BMP, TIFF, JPEG, GIF or PNG file. When a paper map is scanned to produce a geoTIFF raster image file latitude/longitude control points have to be identified and stored as metadata (i.e. georeferenced) together with other essential information such as the map projection, coordinate system, datums, pixel cell scale (e.g. metres per pixel) and latitude/longitude of the first pixel (i.e. the top, left hand corner cell). PDF files may be either raster or vector files. A vector map is a series of mathematically defined points (e.g. airfields, peak elevations), lines (e.g. controlled airspace boundaries, rivers, railways, roads), and polygons (e.g. shapes – towns, lakes, PRD areas) stored as a data file; GPS devices draw their vector maps by plotting the data read from their aeronautical database files. Such maps are usually made up in information layers that allow the display to be de-cluttered by the user as required. Vector graphics are 'rasterised' for display on digital screens. 4.12.3 Airservices Australia's integrated aeronautical information package Aeronautical Information Service [AIS] Some parts of AsA's integrated aeronautical information package [IAIP] may be freely downloaded from the AIS site provided you agree to the terms and conditions of the copyright notice. The .pdf format publications of interest to VFR pilots are the aeronautical information publication [AIP] often referred to as the AIP book, ERSA, the AIP supplements [SUPP] and aeronautical information circulars [AIC]. The documents are in pdf format. The Designated Airspace Handbook [DAH] is also included, it contains 272 pages of tables detailing, for example, the precise location of controlled airspace boundaries either straight line point-to-point or point-to-point arcs of a circle with the centre point location and radius stated, VFR and IFR waypoints — the latter where latitude/longitude may be expressed to 100th of a second [about 30 cm], and much other material. Navigational information datasets In manual flight planning, basic navigational information is obtained from printed documents — ERSA, AOPA airfield directory, ERC-L, VNC, VTC and other reference charts — that contain details concerning airfields, navigation aids, air route intersections, special use airspace, airspace boundaries, magnetic variation and communications frequencies; obviously location latitude, longitude and elevation are particularly important needs. The digital version of such print information is the aeronautical navigation database, see the standard datasets that Airservices Australia markets for creation of such databases. There is a world standard — ARINC 424 — for the format of data intended for navigation system databases. Each data record is 128 bytes (128 characters) in length and made up of primary records and continuation records. The position of the data fields within each type of record is fixed, for example in a waypoint primary record latitude occupies byte positions 33-41, longitude 42-51 and local magnetic variation 75-79. There are three 128 byte continuation records for a waypoint. The database records are transferred as text, comma-separated values [.csv] files perhaps. Obviously it is most important that the data originator of the database material — AsA — has a quality assurance system that guarantees the viability of the data. The data for airfields that are not contained in the standard AsA database might be provided in a supplementary database by the software supplier. The need for absolute assurance that location coordinates and other critical data have been correctly ascertained and entered by the supplier, cannot be over-emphasised. Aeronautical briefing information — the NAIPS Internet Service Other parts of the IAIP — NOTAMs and pre-flight information [PIB] are downloadable from the NAIPS Internet Service [NIS], 'a multi-function, computerised, aeronautical information system. It processes and stores meteorological and NOTAM information as well as enabling the provision of briefing products and services to pilots and the Australian Air Traffic Control platform'. NIS is accessed through the internet with any web browser or access is integrated within flight planning software or within an IPhone app. The Bureau of Meteorology provides all the weather products to the NIS. You must register with AsA before you can access the NIS. For further information see 'Airservices Australia's online meteorological briefing and NOTAM system'. 4.12.4 The electronic flight bag The Australian regulatory status The electronic flight bag [EFB] document reader concept has been utilised, to some extent, for many years by some of the world's airlines, but the burgeoning world-wide public acceptance of tablet computers — led by the Apple iPad — has prompted the ICAO and national airworthiness authorities to expand the regulations and enhance developments directed toward a paperless flight deck/cockpit. An EFB may incorporate a flight planning tool to facilitate the use of the data/documents stored in the EFB, both pre-flight, flight and post-flight. In 2007 the United States FAA released the document AC 91-78 'Use of Class 1 or Class 2 EFB'. That advisory circular is still current (November 2012) and applicable to Part 91 VFR preflight, flight, and post flight operations in the USA. In June 2012 the FAA published AC 120-76B 'Guidelines for the Certification, Airworthiness, and Operational Use of Electronic Flight Bags'. AC 120-76B is directed at the airline transport industry but also intended as a guide for US Part 91 aircraft (mostly general aviation). In November 2012 the CASA released a 'notice of final rule making' including an advisory publication CAAP 233-1(0). (The AWB 00-017 issue 2 of May 2010 was cancelled at that time.) The CAAP defines the EFB as: 'A portable Information System for flight deck crew members which allows storing, updating, delivering, displaying and/or computing digital data to support flight operations or duties.' The CAAP provides general guidance for private pilots and states 'The EFB, with GPS functionality, may be used for situational awareness only. It is not an approved navigation system and cannot be used as the primary means of navigation.' In CAAP 233-1(0) the recommended minimum display screen size was A5 (210 × 148 mm and 257 mm diagonally). The A5 paper-based dimension ratios of 1.41:1 don't equate with the common display screen dimension ratios, e.g, 1024 × 768 pixels is 1.33:1 so, at 197 × 148 mm, the iPad screen is as close as a 1024 × 768 pixel display can get to CASA's A5 recommendation. The iPad Mini dimensions are about 162 × 122 mm and 201 mm diagonally; its display area is about 64% of A5 area. However CASA reviewed their recommendation and issued CAAP 233-1(1) dated 24 August 2013 now recommending a minimum display screen size of 200 mm diagonally across the active viewing area, i.e. the iPad Mini. The iPhone display is probably too small for satisfactory map reading. CASA recommends that a tablet computer should be dedicated to the EFB/flight planning/flight monitoring functions, however it is up to the pilot-in-command of a light aircraft to ensure that a tablet has sufficient/ample capacity for other functions without any chance of affecting the inflight EFB function. CAR 233 requires pilots to carry the latest editions of the aeronautical maps, charts and other aeronautical information and instructions published in AIP or by holders of an 'instrument of approval' . The CASA has the responsibility to regulate the provision of aeronautical information services thus CASA, not AsA, is the approval authority under CAR 233 (1) (h) and 1A; of course AsA is a CASA approved document supplier and their documents do not need additional approval if they have been stored in an EFB in essentially the same form as the original AsA document. At September 2013, CASA has issued written acceptance of quality assurance capabilities for appropriate redistribution of AsA digital VFR/IFR charts and other database material, as part of an EFB package, to only two Australian companies – OzRunways and AvSoft. Jeppesen and Lufthansa System's Lido have an instrument of approval for IFR charts. A notice of proposed rule making — NPRM 0901AS — for CASR Part 175 'Aeronautical information services' was published in 2009 (associated with AsA's intention to change from AIS to aeronautical information management [AIM]) but no notice of final rule making has yet been issued. The proposed 'certificates of authorisation' for persons to act as data service providers will specify requirements to demonstrate that the aeronautical data and information they publish (that pilots are permitted to use as an alternative to the AIP) is equivalent to the aeronautical data published in the AIP and on aeronautical charts, and the service provider's systems and procedures do not introduce errors. In November 2012 an amendment to CAO 82.0 was published adding the requirements to be met for the use of an EFB, by the pilot in command of an aircraft operated under an Air Operator's Certificate, as a means of complying, or partially complying, with CAR 233 (1) (h). (Private pilots may use their own pilot in command authority to approve use of an EFB, bearing in mind the guidance material in CAAP 233-1(0).) The following are extracts from CAO 82.0 Appendix 9 summarising definitions which are likely to also appear in future rules applicable to sport and recreational aircraft: Electronic flight bag, or EFB, means the portable electronic device of an EFB system that satisfies all of the following requirements: (a) it is not an instrument, equipment or navigation computer to which CAR 207 [Requirements according to operations on which Australian aircraft used], CAR 232A [Operational procedures in relation to computers] or CAO 20.18 [Aircraft equipment - Basic operational requirements] apply; (b) it provides, as a minimum, data storage, search, computational and display capabilities; (c) it uses a screen which displays data in a size and form that is at least as easily read and used as it would be in a paper document for which the EFB would be a substitute; (d) it is used primarily by the flight crew for the purpose of accessing and using data relevant to the operation of the aircraft EFB system means the hardware, the operating system, the loaded software and any antennae, connections and power sources, used for the operation of an EFB Class 1 EFB means an EFB that is portable but not mounted (on the aircraft) Class 2 EFB means an EFB that is portable and mounted (on the aircraft) Note: Class 1 and Class 2 EFBs are portable electronic devices [PEDs] and limited to functionality level 1 and 2 software. Functionality level 1 means that the EFB: (i) is used to view the aeronautical maps, charts, and other aeronautical information and instructions mentioned in CAR 233 (1) (h) but without the functionality to change any of that data; and (ii) may have a flight planning tool to facilitate the use of the data mentioned in subparagraph (i); and (iii) may be 1 or more of the following: (A) held in the hand; (B) mounted on an approved mount; (C) attached to a stand-alone kneeboard secured to a flight crew member; (D) connected to aircraft power for battery re-charging; (E) connected to an installed antenna intended for use with the EFB for situational awareness but not navigation; and (iv) unless secured in accordance with sub-subparagraph (iii) (B) or (C) must be stowed: (A) during take-off and landing; and (B) during an instrument approach; and (C) when the aircraft is flying at a height less than 1 000 feet above the terrain; and (D) in turbulent conditions; and (v) has no data connectivity with the avionics systems of the aircraft; and (vi) may have wireless or other connectivity to receive or transmit information for EFB administrative control processes only Functionality level 2 means that the EFB: (i) must have the functionality of functionality level 1; and (ii) subject to subclause 1.4, has 1 or more software applications that use algorithms requiring manual input to satisfy operational requirements; and (iii) has no data connectivity with the avionics systems of the aircraft; and (iv) may have wireless or other connectivity to receive or transmit information for EFB administrative control processes only Note: examples of software applications that use algorithms requiring manual input to satisfy operational requirements include weight and balance calculations, or performance calculations required by the aircraft's approved flight manual, e.g. density altitude and take-off distance required. EFB software suppliers As mentioned above there are a few Australian producers of flight planning software who have entered a Standard Data Licence Agreement with Airservices Australia enabling the inclusion of the AsA map packs as part of their software package. Two of those flight planning software producers market the concept of a tablet computer/mobile broadband hardware system combined with EFB + flight planning + GNSS + flight monitoring software. At November 2012 the software from both producers is only Apple iOS compatible and intended for the iPad, but it can be installed in an iPhone for ground use — iPhone hardware does not meet the CASA's expectations for flight use. Note: it is the pilots legal requirement to carry the current maps and charts for the sector to be flown, that have been approved by CASA. At September 2013 two EFB products have been approved by CASA for VFR pilot use [see above] as an alternative to the AIP paper publications, so other EFB products cannot be used as an inflight substitute for the paper charts sourced from Airservices' AIS. Thus until an instrument of approval has been received by the relevant data service provider, AsA's paper charts must be available in flight; another electronic device cannot be nominated as a back-up system. With two data service providers holding a CASA instrument of approval for the digital WACs and VNCs private VFR pilots are able to use an acceptable tablet computer, rather than paper charts, as the primary means of in-flight documentation. Even so, although an EFB is a paper replacement system, it is prudent to carry back-up paper charts. During 2012-2013 the CASA flight operations inspectors were surveying iPad and flight planning software usage when conducting ramp checks. EFB suppliers sell their VFR software product on a remarkably low cost annual subscription basis — at November 2012 around $75 p.a. The subscription includes the complete AsA VFR digital chart pack for Australia and the updates of charts and other aeronautical data in accordance with AsA's standard update cycle; it also includes software updates/expansions. Those data service suppliers might alter the AsA product; for example the 43 WAC charts have overlapping seams and the EFB supplier might 'stitch' all the individual charts together to produce one very large seamless mosaic. Locality names, or parts of names, may disappear from the seamless mosaic. Such activity, being an alteration of the AsA material, may be prohibited within a CASA approval instrument. Data service providers approved under CAR 233 (1) (h) must also ensure that all database material supplied cannot be modified by the user. The freely available Aeronautical Information Publication plus updates is also included in the package — the EFB supplier may add a search facility for ERSA and the AIP book. Mobile broadband service provider's charges are, of course, an additional cost to be considered. Although there may be a GPS engine included in the hardware it is recommended that an external GNSS aviation receiver engine be linked to the hardware. There are packaged GNSS engines available which output the navdata, via a Bluetooth connection, to an iPad, iPhone, Android or other display device. The cost for aviation types is $75 to $150. For example the Garmin GLO for aviation costs about $150 and receives position date from GLONASS and GPS satellites (thus 48 satellite potential) with an update rate of 10× per second. Weight is 60 grams and USB connection also available. Note: from 2 February 2017 all aircraft operating under the instrument flight rules must carry ADS-B OUT equipment. It is probable that many of those aircraft will also install ADS-B IN. It is then likely that a tablet type computer, linked to the ADS-B receiver, could be used for the cockpit display of traffic information. The mobile broadband connection allows inflight connection to BoM weather radar, internet lightning trackers, regular checking of the NAIPS Internet Service for changed information relative to the flight plan (SIGMETs and SPECI for example) and to overlay that information graphically on the moving map display. Note: the use of a cellular mobile voice or data communication device in an aircraft — that is not equipped with a picocell base-station — is not in accordance with the class licence that legalises personal transmissions from a mobile telecommunications device; see further information about the Radiocommunications (Cellular Mobile Telecommunications Devices) Class Licence 2002. The EFB suppliers' products are: AvPlan — from AvSoft. OzRunways EFB — from OzRunways. It is suggested their manuals be downloaded for full information. 4.12.5 Electronic VFR flight planning Basic needs Good electronic VFR flight planning should only differ from the manual flight planning steps outlined in sections 3.4 to 3.6, in that digitised topographical charts and graphical flight planning software — rather than paper, protractor, E6-B etc — are used to plan, and finally plot, the route. The graphical flight planning software downloads weather and notams from Airservices Australia's NAIPS Internet Service; provides tracks, distances, magnetic headings, times, fuel burn etc and stores the final flight plan in digital format and, if required, uploads a flight notification with sartime to NIS. When airborne there is only a slight difference in pilotage where the navigator — while still reading from map to ground — is primarily relying on a cursor on a digitised moving AsA topographical chart to display current position and then confirming it with the ground. The significant difference in airborne sport and recreational aviation navigation is in using the flight plan plus GNSS reception to provide in-flight data, warnings and corrections necessary to arrive at the planned destination safely, avoiding PRD areas where necessary and without infringeing controlled airspace. That indicates the vital importance of working with a complete, current and absolutely accurate aeronautical database. Proper use of VHF radiocommunication frequencies is an adjunct to normal VFR navigation procedures. In flight the software should facilitate any necessary changes to the plan and recalculate all relevant data. For VFR operations outside controlled airspace, electronic planning and navigation should follow much the same manual procedures described in the preceding modules of the navigation tutorials: accessing airfield and airspace information planning the waypoints/landing points/alternates for a safe route — using PCA, WAC, VNC, VTC and ERC-L charts — taking controlled and PRD airspace into account accessing all applicable TAFs, ARFORs, METARS, NOTAMs, surface charts, BoM radar images and AWIS doing the E-6B calculations and producing a preliminary flight plan determining beginning/end of daylight assessing fuel needs establishing the VHF communication frequencies applicable to each section of the flight filing the flight plan with the NAIPS Internet Service or emailing a flight note to a responsible person facilitating the weight and balance checks calculating density altitude and take-off/landing distance required facilitating aircraft checklist run-through monitoring flight by checking from digital map display to ground making the necessary en route flight path adjustments and revising the flight plan timing, fuel needs etc. In addition — as a flow-on from the glass primary flight displays and multi-function displays of larger contemporary IFR aircraft — the availability of relatively inexpensive non-certified electronic flight instrument systems [EFIS] is increasing. Such non-certified systems can be installed legally in RA-Aus amateur-built category aircraft — although there will be a regulatory problem if a non-certified EFIS is providing altitude encoding to a transponder. These flight instrument systems are microchip-based (including micro electro-mechanical gyroscope devices and acceleration sensors) and, in the future, are likely to provide increasing data transfer to or from other avionics within electronic communications, navigation and surveillance technology. VFR flight planning software packages There are several Australian flight planning software packages currently available that have a solid history of development and provide similar VFR application Command Flight Planner from Command Software is a Windows product. IFR oriented but there is a facility to overlay the flight plan on a Google Earth image. The route can be exported to a GPS via a .gpx (GPS exchange format) file. An excellent screencast provides a demonstration of the flight planning procedure, for an IFR flight, but the procedure for a VFR flight is similar. Purchase price is about $400; the annual fee for software updates plus updates of AsA aerodrome, airway, and airspace data in line with ERSA issue dates is less than $100. Flight Planner 3000 from Champagne PC Services is a Windows product. Software has database waypoint route planning with selectable display — but does not include digitised topographical charts, so it may not provide increased VFR situational awareness. The route can be exported to a GPS via a .gpx file. Purchase price is about $400; the annual fee for updates of AsA aerodrome, airway, and airspace data is less than $100. AirNav VFR — from Sentient Software. A Windows system, AirNav VFR comes bundled with one of five VFR map packs containing Airservices Australia's charts, at a cost from about $120. There is an optional discounted subscription for the biannual chart reissues. Each chart pack contains all WACs, VNCs, VTCs and ERC-Ls relevant to the area; for example the south-eastern Australian pack contains 8 WACs, 3 VNCs, 9 VTCs and 4 ERC-Ls. The software prevents access to the stored VNCs, ERC-Ls and VTCs two weeks after the official chart expiry date, but the access to WACs is not time-limited. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.11.1 Tracking to an NDB A useful ADF application in visual navigation is to locate a particular NDB and then track — or home — directly to it. The ADF receiver is tuned to the NDB frequency, and the audio volume turned up, so that the NDB can be identified as soon as the aircraft comes within range. The ADF needle indicates the bearing to the NDB. The wind correction angle necessary to maintain that track is then ascertained by bracketing, a technique that bears some similarity to the double track error method. The term 'bracketing' is derived from the artillery technique for ranging the target by deliberately placing initial rounds behind and in front of it. Note: this sequence is best performed if the heading being flown is positioned on the ADF card at TDC. The diagrams in the left column below indicate the readings with those settings. PROCEDURE Needle position Compass heading Event sequence 060° Position A. When receiving the NDB signal turn the aircraft so that the head of the ADF needle is pointing to TDC, then check the heading from the compass. That heading is the track required to home directly to the NDB; for our example, 060° magnetic. Rotate the ADF compass card to set 060° at TDC and the needle head will also indicate 060°. Remember that all heading changes should be logged. 060° Position B. As the flight progresses, holding the 060° heading, the crosswind causes the aircraft to drift to the south of the required track and the ADF needle has moved left about 5° to 055°. 030° Position C. We now have to make a first rough cut at the track error — it is best to initially overestimate so let's choose 15° and, applying the double track error technique, we turn left 30° onto an intercept heading of 030° magnetic. Positioning the 030° heading at TDC, the head of the needle will still initially indicate 055° but will move towards 060° as we close with the required track. 045° Position D. When the needle reaches 060°, the 060° track to the NDB has been regained. Now halve the intercept angle (i.e. subtract the track error) and turn right onto an initial wind correction heading of 045° magnetic; i.e. the estimated track error was 15°, we turned left 30° onto the intercept heading of 030° and now, having regained the required track, we turn right 15° onto a wind correction heading of 045°. Now rotate the card to the 045° heading and the needle remains at the 060° bearing. 045° Position E. If the 15° WCA is correct then the ADF needle will remain at the 015° position whilst the 045° heading is maintained. However, it is most likely that we have overcorrected. The aircraft will drift north of track, shown by the needle moving clockwise a few degrees from the 015° position — so we now have to refine the wind correction angle. 055° Position F. We might guess that we have overestimated the WCA by about 5° so, applying the double track error technique, we turn right 10° onto an intercept heading of 055° magnetic. Positioning the 055° heading at TDC, the head of the needle will still initially indicate something greater than 060°, say 063°, but will move towards 060° as we close with the required track. 050° Position G. When the needle reaches 060°, the 060° track to the NDB has been regained. Now halve the intercept angle and turn 5° left onto a wind correction heading of 050° magnetic, rotate the card to the 050° heading and, if we've estimated correctly, the needle will remain at the 060° bearing, maintaining a 10° WCA, while we continue along the required 060° track to the NDB. 4.11.2 Tracking from an NDB Another useful application for the ADF in visual navigation is in determining track error when departing from an airfield equipped with an NDB or when overflying an NDB. For example: the flight plan calls for a departure — from overhead an NDB — on a track of 240° magnetic. Any necessary wind correction is to be assessed after departure, using the ADF, with the track recovery and heading correction to be made by a slightly modified double track error method. (The modification is that rather than timing the intercept leg to estimate track recovery we will use the ADF needle to indicate when we are back over the required track.) In this ADF application the ADF card may be used with the 0° position set at TDC or your personal preference may be to set the 240° heading at TDC. The diagrams and the text below indicate the procedure and the readings with 0° positioned at TDC, but the additional text in italics is the procedure when rotating the card to the new heading for every change. Hopefully you will be able to see that the latter method is easier to handle. Note that when tracking away from an NDB we use the tail of the ADF needle, rather than the head, as the indicator. PROCEDURE Needle position Compass heading Event sequence 240° Departing from overhead the NDB to track 240° magnetic. The magnetic compass heading is 240° (i.e. no wind correction provision) and the tail of the ADF needle swings to the 0° position. With the 240° magnetic heading set at TDC the position of the needle relative to TDC is exactly the same as in the diagram but, on the background card, the needle tail indicates the 240° heading. 240° Position B. As the flight progresses holding the 240° heading, the crosswind causes the aircraft to drift to the south of the required track. The tail of the ADF needle has moved about 15° to 345° and is in the left half of the card. Thus the opening angle, or track error, is 15° and the tail of the needle represents the track made good, which is 15° to the left of the required track. With the 240° magnetic heading set at TDC the tail of the needle will indicate the track made good, 225° or an opening angle, or track error, of 15°. 270° Position C. Use the double track error method to intercept the required track. The aircraft is turned 30° (2 × 15) onto a heading of 270° magnetic. The ADF needle tail initially moves 30° to 315° then commences to reverse direction as the 270° heading is maintained and the aircraft is closing the 240° track out. The aircraft is turned 30° (2 × 15) onto a heading of 270° magnetic, and 270° magnetic is now set at TDC. The tail of the needle will then initially still indicate 225° but will move towards 240° as you close with the required track. 270° Position D. When the needle has moved through a 15° arc and is back to the 30° left position (330°), on a heading of 270°, the 240° track out from the NDB has been regained. With the 270° magnetic heading set at TDC, the 240° track out from the NDB has been regained when the tail of the needle reaches 240°. 255° Position E. Subtract the track error (15°) and turn left onto the new heading of 255°, which will then maintain the necessary 15° wind correction angle. The ADF needle moves 15° clockwise and the aircraft should hold the required track – if the heading is maintained and the needle kept at the 345° position. Subtract the track error (15°) and turn left onto the new heading of 255°, which will then maintain the necessary 15° wind correction angle. Set the 255° magnetic heading at TDC — the tail of the needle now indicates 255°. After flying this heading for a while you may find that you still have some drift, which is indicated by movement of the needle. In this case a small heading correction is usually enough compensation. 4.11.3 Running fix/distance from NDB Whenever your track will pass abeam an NDB, it is quite easy to obtain a running fix using the 1-in-60 rule and a little mental arithmetic, providing you have a reasonable idea of your ground speed. The technique is illustrated in the diagram. Procedure 1. Tune and identify the NDB, set your heading at TDC and watch the ADF needle. The NDB is directly abeam when it moves to 90° either side of TDC, position A in the diagram. 2. Note the time and continue flying your heading, for example 040° magnetic. 3. When the needle has moved a sufficient amount to get a good reading (position B on the diagram), note the time and the bearing from the NDB, indicated by the tail of the needle. Let's say the needle has moved 10°, the elapsed time is 8 minutes, the bearing from the NDB is 110° magnetic and you reckon your ground speed at 70 knots. 4. Now we calculate the distance we are along that bearing using the 1-in-60 rule: i.e the distance (nm) from the NDB = elapsed time (mins) × ground speed (kn) degrees traversed = 8 × 70/10 = 56 nm. The aircraft's position at time B is then 110°/56 nm from the NDB. The real difficulty now is to measure and plot that position on the navigation chart (not forgetting to convert the bearing to degrees true) so perhaps get the passenger to do it while you fly the aircraft. It is always a good idea to get your passenger involved in the flight. If you are wondering what happened to the '60' in the 1-in-60 application the answer is that it is negated by the usage of minutes in one factor and nautical miles per hour in another. In the diagram, the dashed red line outlines the right angle triangle on which the calculation is based — the distance from the NDB to position B forms the hypotenuse. 4.11.4 ADF simulation If your browser is Java-enabled then I suggest a visit to FergWorld and try out the ADF trainer applet. Drag the aircraft symbol to position your aircraft then set the aircraft's heading on the directional gyro and read off the bearing to/from the NDB. Note the ADF card is non-rotatable. Try the quiz. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.10.1 Global navigation satellite systems [GNSS] Future development GNSS is the generic term covering satellite-based, three-dimensional position fixing, timing and navigation systems. The first such — and currently dominant — system is NAVSTAR or GPS, initially developed by the United States Department of Defense for position fix coordination of the inertial navigation systems [INS] on board military aircraft and cruise missiles. GPS has since become freely available — as a valuable primary and supplemental navigation aid — to civilian aircraft of all types and all nations, with the compliments of the U.S. government. The Russian GLONASS GNSS system now has 24 satellites in orbit and providing world coverage. Some GPS receivers can utilise both GLONASS and GPS satellites singly or jointly. The European Union's Galileo GNSS system is undergoing trials with several satellites in orbit and is scheduled for full operation capability by 1920. China's national satellite system is being expanded into 'Compass', a 35 satellite GNSS system. Massive growth is expected in the application of the satellite navigation systems, with aviation being a very small part of the total market. The European GNSS Agency 2012 world market estimate is $48 billion and the 2020 market forecast is $128 billion. The split-up by market sector for cumulated revenues from GNSS devices for the period 2010 to 2020 is: Road: personal navigation devices and in-vehicle devices – 54% Location based services: smart phones etc – 43.7% Aviation – 1% Agriculture – 1% Land and hydrographic surveying – 0.6% Commercial maritime – 0.1% It is estimated that 80% of all applications would be satisfied by a one standard deviation accuracy of one metre. (One standard deviation refers to a statistically normal distribution where 68% of all measurements will be within that accuracy.) The European Space Agency's new [2011] Navipedia website contains a large store of information regarding GNSS in general and Galileo in particular. For a more generalised GNSS overview download the Navipedia Galileo booklet. The Global Positioning System GPS or the NAVigation Satellite Timing And Ranging [NAVSTAR] system consists of a minimum 24 satellites (of which usually three are operating spares) orbiting Earth at an altitude around 19 000 km, with each unit taking about 12 hours to complete one orbit. The NAVSTAR orbits are arranged in six planes with three or four satellites in each plane. This configuration ensures that a minimum of four satellites would be in view from most locations on Earth at any time. Each NAVSTAR unit is solar powered and equipped with atomic clocks for extremely accurate time measurement. The satellites have an operational life of around 10 years, so there is a continuing replacement program, which also allows phased introduction of new technology and expanded facilities. Early launch of replacements plus satellite trials often provides more than 30 units in orbit. Initial Operating Capability was established in 1993 with 24 operational NAVSTAR units in orbit. NAVSTAR satellites continuously broadcast information on very low power at two UHF L band frequencies. The civilian standard positioning service [SPS] 'coarse/acquisition' ranging code at 1575.42 MHz (the L1-C/A code) and an encrypted precise positioning service code (the PPS code) at 1227.60 MHz. The L1-C/A code is freely available to all while the PPS code is only available to authorised users — chiefly military. The basic L1-C/A code is designed to provide a latitude/longitude position-fixing accuracy within 300 metres 99% of the time and within 100 metres laterally and 140 metres vertically 95% of the time; but probably better than 30 metre lateral accuracy is achievable most of the time without augmentation. Some manufacturers quote accuracies of 3 metres, or less. At present it is far more accurate than necessary for flight under the VFR though perhaps the higher accuracies might be valuable if using georeferenced taxiway diagrams at a major airport. All civilian GPS receivers are SPS units receiving the L1 code, however a new, easier to track civilian signal [L2C i.e. Civilian] has been added at 1227.60 MHz to provide some redundancy and improve accuracy for dual-frequency receivers. The accuracy obtained is said to be as good as that obtained with the military PPS code. Each satellite continually transmits three sets of information contained in the L1 code navigation message: its own ID, current date and time ephemeris* data that the receiver uses to calculate the precise position of that satellite at the start of the navigation message and which includes current orbital position data and predicted orbital positions for the next few hours plus its 'health' status almanac data providing future orbital position and time information for all the currently operational GPS satellites in the constellation. The almanac data helps the receiver determine which other satellite ephemeris data to use. The almanac data is valid for 30 days or more. *Note: in astronomy the ephemeris term describes a table of predicted positions and is derived from the Greek word for diary. In essence the aviation GPS receivers use the information, emanating from each satellite in view, to measure the time lapse of a received radio signal, calculate the distance to each satellite's position and then establish the receiver's three-dimensional position by trilateration — a form of triangulation — of the distances from a minimum of three satellites. But simultaneous range calculations from four satellites are necessary to correct for the clock error in the GPS receiver. Although the receiver would normally calculate an altitude, external input of an aircraft's altitude — baro-aiding — can provide a further range measurement — that from the centre of the Earth, thus simulating an additional satellite; but see 'The WGS84 ellipsoid and the geoid-ellipsoid separation'. Aircraft positions are calculated by the receiver in terms of latitude, longitude and GPS altitude. The receiver chips contain mathematical models of the Earth. The most accurate, and commonly used for aviation purposes, is the World Geodetic System 1984 [WGS84] which is the lateral datum for WACs and — in Australia — VNCs, VTCs, aerodrome reference points and VOR sites. The Australian Height Datum is the vertical datum for Australian charts. See 'Defining the shape of the Earth – ellipsoids and geoids'. Note: GLONASS uses a different coordinate system to WGS84 which might result in a 20 metre or so difference in position relative to the GPS calculation. For an outline of the Global Positioning System visit Navigation Programs - Global Positioning System in the Federal Aviation Administration's website. Navigation system performance criteria There are four parameters for assessing the performance of a navigation system: availability/vulnerability, accuracy, integrity (i.e. trustworthiness) and continuity of service. Availability/vulnerability: the basic GPS civilian service is available nearly 100% of the time. Integrity refers to the trustworthiness of the device, i.e. the information provided is of the required standards for the particular application and the user is alerted when it is not. If a particular system is demonstrated to satisfy all four parameters for a flight phase then it may be classified as a sole-means navigation system — for that phase. When operating under the day visual flight rules, only navigation by visual reference to the ground satisfies all four parameters. A supplemental-means navigation system may be used in conjunction with a sole-means navigation system. Pilots operating under the VFR may use GNSS to supplement map reading and other visual reference navigation techniques. Any GPS receiver may be used but if it is an installed (i.e. not readily demountable) receiver it must be fitted in accordance with CAAP 35-1 or AC21-36; see AIP GEN 1.5 section 8.5.4. GNSS is only officially regarded as a primary-means night VFR navigation if the GPS receiver system accords with the FAA's Technical Standard Order [TSO] C129 or TSO C145/6 series, or has other CASA approval. If a system navigation meets the integrity and accuracy requirements all the time, but falls short on availability/vulnerability or continuity of service, it may be approved as a primary-means navigation system for a flight phase, if specified procedures are employed. Day VFR navigation does not use primary-means systems, only the visual reference system plus supplemental-means systems as required. For more information concerning the use of GNSS in VFR navigation see AIP ENR 1.1 paragraphs 19.2 and 19.5. Note the wording of sections 19.2.1e and 19.5.1d together with the latter's link to AIP GEN 1.5 section 8. Also read CAAP 179-1(1) section 5 'Navigation using Global Navigation Satellite Systems'. 4.10.2 GPS receivers and augmentation systems Portable and panel mountable/demountable GPS receivers Manufacture of GPS receivers for all applications is a multi-billion dollar industry supplying, just in the avionics field, a wide range from inexpensive handhelds — not much more advanced than the 1995 Magellan SkyBlazer first-generation receiver illustrated — to very expensive aviation panel mounts with integrity monitoring, ground-based position correction capability and colour moving-map position and terrain awareness warning systems [TAWS] displays. Inbuilt GPS reception capabilility has been added to smartphones and other portable consumer electronic devices, — perhaps in the form of a Trimble chip set — but such GPS capability may not be suitable for aviation use. Some dual receivers have GPS and GLONASS reception capability. The simplest handheld satnav receivers always contain a re-writeable user's database to store a reasonable number of planned flight routes and perhaps a thousand user-defined waypoints (icon, name, latitude and longitude). The actual track date from previous flights can also be stored. Aviation portables will also provide a recognised standard aviation navigation database, possibly compiled by Jeppesen (the Pacific Basin) and containing: location/elevation coordinates and other information for all aerodromes referenced in ERSA; plus VORs, NDBs and intersections shown on ERCs; plus controlled and 'special use' (PRD areas) airspace. Those location references may also be used as waypoints when defining routes. Those simplest receivers provide elementary 'moving map' graphics that display the aircraft's position and the relative position of all the waypoints and aviation-related detail within a user-defined range. The diagram or 'map' can be configured to remove unnecessary items from the display and thus present a less cluttered image. Display diagonal dimensions are typically 4.3 or 7 inches for the portable units. Purchase cost for the simpler aviation 4.3 inch receivers may be $500 - $750 while the cost of those in the middle of the portable range may be $1200 to $1750. The illustrated AvMap EKP IV receiver is in the middle of the portable range. It first appeared around 2006 and is representative of a 7 inch colour 800 × 480 pixel LCD receiver with split screen capability. The image shows two of the navigation screens — moving map on the left and horizontal situation indicator [HSI] on the right. A third screen is a navigation and location screen with a course deviation indicator [CDI] display. A compact flash memory card contains Jeppesen NavData plus land cartography. The amount of cartographic detail displayed can be varied. Internal RAM can store up to 1000 user-defined waypoints and up to 15 flight plans. The unit uses an external antenna and needs external power supply — battery life is limited and may be regarded as an emergency supply. An NMEA 0183 serial input/output data port is included. There are a limited number of portable/panel mountable aviation GPS receiver manufacturers: Bendix King, Garmin and AvMap are probably the best known in Australia. Calculating altitude 'GPS altitude' is calculated as the elevation above the WGS84 ellipsoid, which differs from the Australian height datum geoid [AHD]. Aviation GPS receivers navigation data should include tables/algorithms (based on latitude/longitude grids of varying cell sizes) of the geoid-ellipsoid separation values to allow the altitude above the AHD (rather than the WGS84 ellipsoid) to be displayed. Non-aviation receivers generally display only GPS altitude. In Australia the geoid-ellipsoid separation is quite significant, varying between minus 110 feet and plus 220 feet. At Cooktown, north Queensland, the airfield elevation is 26 feet (7.9m) and the AHD is 65.2m above the WGS84 ellipsoid (the N value) so the ellipsoidal height at the airfield would be 73.1 metres and a GPS receiver that doesn't adjust for the geoid-ellipsoid separation would indicate an airfield elevation of 240 feet; i.e. altitude is overstated by 214 feet. See 'Altitude and Q-code definitions'. The GLONASS system uses a different ellipsoid so the geoid-ellipsoid separation would differ from the WGS84 separation. Configuring displays Aviation GNSS receivers offer a variety of screen displays with user-configurable content that varies between models. The most useful displays for supplementary-means purposes are a moving topographical map screen, a horizontal situation indicator screen and an alphanumeric navigation screen. Some handhelds might provide just a basic cartographic which may be a monochromatic or colour representation of a few significant line or point features (highways, railroads, coastlines) on which aviation-related detail (airfields, PRD areas and controlled airspace boundaries) is overlaid. This is generally sufficient for VFR navigational use, but there are more expensive handhelds on the market which provide a topographic, colour moving map display — possibly with terrain clearance indication — but note these may not be WACs, VNCs or VTCs and such map displays for Australia are likely to have some detail deficiencies. Also the screen area is too small to show normal map detail. GNSS terrain clearance warning is not particularly useful for VFR navigation; Mark 1 eyeball, which also takes in the cloud base, is superior. The basic moving map, which is the preferred navigation mode, is usually configured to show an aeroplane image at the lower centre of the screen representing the aircraft's position in relation to the flight-planned track between current waypoints, airfields and controlled airspace, etc. The display can be configured as 'north up' or 'track up'. The 'track made good' will also be displayed, together with bearing and distance to the next waypoint. The display can normally be zoomed in or out and thus represent an area ranging from a few square miles to thousands of square miles. The alphanumeric display might show track made good, ground speed, distance and bearing to the next waypoint, ETE to the next waypoint plus a course deviation indication similar to that of a VOR omni bearing indicator. The division dots on a GNSS CDI are not spaced at two-degree intervals, but generally indicate distance off track — with the interval between dots being user-scalable from maybe 0.25 nm up to 5 nm. Some devices may change scale automatically as the waypoint is neared. The bar indicates where the required track is in relation to the aircraft; e.g. if the interval is set at one nm and the bar is located three divisions to the right of centre then the required track is 3 nm to the right. Stand-alone GPS/GNSS engines There are packaged GNSS engines available which output the navdata, via a Bluetooth connection, to an iPad, iPhone, Android or other display device. The cost for aviation types is $75 to $150. For example the Garmin GLO for aviation costs about $150 and receives position date from GLONASS and GPS satellites (thus 48 satellite potential) with an update rate of 10× per second. Weight is 60 grams, USB connection also available. 4.10.3 Performance standards for installed receivers in IFR aircraft Unlike aircraft operating under the day Visual Flight Rules, aircraft operating under the Instrument Flight Rules must use a GNSS system that meets the minimum performance standards of a Technical Standard Order (TSO) issued by the United States Federal Aviation Administration (FAA). The manufacturer of the system must hold a TSO authorisation issued by the FAA aircraft certification office; such receivers are then generally acknowledged as being 'TSO compliant' or "TSO'd". Note: there are no certification standards for the hand-held GPS devices used in sport and recreational aviation; i.e. they are non-TSO'd. There is little to gain by installing a more expensive TSO'd device in a RA-Aus aircraft. The TSO'd receivers are not necessarily more accurate than a non-TSO'd receiver. Panel-mounted GNSS receivers are certified to comply with TSO C129 which is a 'supplemental means' standard for IFR en route navigation that includes Receiver Autonomous Integrity Monitoring (RAIM). RAIM is an aircraft-based GNSS augmentation system (ABAS) that identifies any satellite that is not meeting specified standards and alerts the pilot. Portables only have the ability to inform the user when navigation has ceased entirely; they don't warn when a significant degradation in accuracy (the precision of the position solution) is occurring. TSO C145 and C145a add a fault detection and satellite exclusion system (FDE) plus capability to use an American satellite-based augmentation system (SBAS) known as the Wide Area Augmentation System (WAAS); though WAAS is not available in Australia. TSO C146 and C146a includes SBAS and is a standalone system with the same status as ADF and VOR, i.e. a primary means of navigation. No satellite-based augmentation system is yet planned for Australia though the Japanese SBAS, known as MSAS, has one of its six ground reference stations in Canberra. Ground Based Augmentation Systems (GBAS) are local systems installed at airports that transmit data to aircraft during precision approach and landings (GNSS Landing Systems or GLS) and will eventually replace Instrument Landing Systems (ILS). One GLS system is fully functioning at Sydney airport (2012) but handling only GLS receiver equipped Qantas Airbus A380 and Boeing 737-800 approach and autoland operations. See CASA 156/12. In aviation a 'sole means' precision IFR navigation system has to meet certain standards with respect to accuracy, integrity, availability and continuity of service. GPS by itself cannot meet those standards. However, the ICAO nations are developing a sole means global navigation satellite system based on GPS and Galileo augmented with ground- and space-based correction (or Differential GPS) systems, airborne avionics plus digital data link communications and surveillance between aircraft and ground stations. (See ADS-B surveillance technology.) GNSS will eventually make obsolete all VORs, NDBs and other ground-based systems* and — as manufacturers are prepared to develop low-cost light aircraft avionics — it may have considerable spin-off benefits to recreational fliers. *But not a self-contained onboard system like an Inertial Navigation System. GPS is used to supply position-fixing data to INS which, while its electronic DR is very accurate, still has a gyro drift inaccuracy up to one nautical mile per hour. For further information see CAAP 179A-1 Navigation using GNSS [2006], also see the CASA booklet Overview of GNSS [2006] and take particular note of the human factors section. Note: a robotic antenna calibration facility installed at Geoscience Australia and used to calibrate the 200 GNSS antennae forming part of the Australian Geophysical Observing System (AGOS) is expected to increase their satellite positioning precision to less than one millimetre. 4.10.4 GNSS VFR applications Establishing a flight plan The primary use of GNSS in sport and recreational aviation is in en route navigation — monitoring flight progress against the established flight plan and providing the heading corrections necessary to maintain the required track. This requires entering the planned route into the GNSS database, activating that GNSS route on take-off and making the necessary adjustments, as indicated by the GNSS, to maintain track. When used in the moving map navigation mode, the GNSS display exactly complements the en route navigation techniques expounded in section 8.3. The following is a basic illustration of GPS practice, for example let's take our planned flight from Oxford to Tottenham: Completed flight plan Segment Altitude Distance Track [mag] Heading [mag] Ground speed ETI Comms Oxford – Warraway Mountain 3500 74 083° 079° 67 66 ML 124.9 Warraway – junction 3500 52 050° 050° 65 48 ML 124.9 Junction – Tottenham 3500 33 029° 031° 65 30 ML 123.9 QNH: 1027 Last light: 1755 hrs AEST Fuel margin: 40 mins Entering the flight plan route Let's assume our GPS receiver contains a standard aviation database, in which case the only waypoint already existing would be Tottenham (YTOT) and the others would have to be entered into the users database thus: Oxford S33° 02.5' E144° 35.1' Warraway S33° 06' E146° 02.5' Junction S32° 40.6' E146° 57' The route would be given an identification and simply entered into the route database as: Route ID: Oxford – Warraway – Junction – YTOT The processor will calculate and provide an alphanumeric display of the required track and the distance for each leg, which must then be verified with the flight plan data. If they don't agree the cause must be found and corrected. The database contains the isogonals for the region so the required tracks can be displayed as magnetic or true. Note that the map screen will also include details from the standard aviation database; in this case the airfields at Ivanhoe (YIVO), Lake Cargelligo (YLCG) and Condobolin (YCDO). Monitoring progress Once airborne, and the receiver has locked on to the required number of satellites, the planned route is pilot-activated. The navigation computer within the receiver recalculates the aircraft's position at set intervals of one or two seconds, or less, keeps a history log of the track made good, and continually recalculates groundspeed and distance off track. The moving map display at left shows the situation at our position fix at Trida enroute from Oxford. The indication is that we have drifted left of track, the bearing to the first waypoint at Warraway is 085° magnetic and the distance to run is 52.4 nm. The track made good was 077° magnetic and the ground speed since activating the route is 55 knots. In this screen the system acts exactly as if there is an NDB at Warraway and the GPS receiver homes to it by indicating the bearing. However, if you just fly that bearing, without any heading adjustment for the crosswind component, the bearing will keep changing due to the drift and, like the ADF, you will eventually arrive at Warraway — but the track followed will be curved and the magnetic heading flown will be changing consistently. Thus to maintain a constant heading, and the direct track, you still have to calculate and apply the wind correction angle. The GPS doesn't directly calculate the heading to fly, either to regain the planned track or fly direct to Warraway. You don't need to estimate the track error from your chart the GPS shows the track made good as 077°, the track required was 083° thus the track error is 6°. You can then apply the double track error technique to regain and hold the original track. You will know you have regained track when the GPS indicates that the bearing to the waypoint is 083° so you then make the necessary heading adjustment to maintain track. If you continue to adjust your heading so that track made good — not your heading — matches the bearing you will theoretically continue tracking directly to the waypoint. Alternatively if you prefer to fly directly to the waypoint, rather than first regaining the original track, a quick mental calculation of track error/closing angle will provide the wind correction angle to accomplish that. e.g. track required = 083° track made good = 077° thus opening angle = 6°. Track required = 083°, bearing to waypoint = 085° thus closing angle = 2° Opening + closing angles = 8° WCA to track direct to the waypoint. In fact that calculation is simplified by using the difference between the track made good and the bearing; i.e. bearing = 085°, track made good = 077° thus WCA = 8°. Some handhelds may do the calculation for you in providing a TURN display, which is just that difference between the track made good and the bearing. The alphanumeric display at left, for the same flight situation, shows the same bearing, distance, track made good and ground speed information. In addition the CDI indicates that the required track is about 3 nm to the right of the present position, the estimated time en route to reach Warraway is another 55 minutes and the actual distance off track or the cross-track error [XTE] is estimated at 3.15 nm. The type of navigation using this display is very much the same as tracking in on a selected VOR radial and obviously to maintain a constant heading you still have to calculate and apply the wind correction angle. Handheld aviation GPS receivers normally provide an E6-B page where, if you enter the heading being flown and the true airspeed, the receiver will estimate the 'winds aloft' from the TMG and ground speed, and then calculate the heading to fly. Due to the limited key board it is not so easy to input data during flight in a light aircraft , so it is much easier to just use the variant of the track error/closing angle calculation outlined above. Remember that during flight under the Visual Flight Rules you are required to navigate by map reading and visual reference to the ground, not the GPS display. That display should only be a fractional portion of your continuing scanning pattern. The VFR rules (ERSA ENR 1.1 para 19.2) state 'the pilot must positively fix the aircraft's position by visual reference to features shown on topographical charts at intervals not exceeding 30 minutes.' Emergency search feature Aviation GPS receivers all provide an emergency search key, possibly labelled 'GOTO/NRST'. Pressing this key once will bring up a screen displaying the 10 nearest airfields extracted from the database together with the distance and bearing to each. Highlighting one of these airfields and then pressing the 'GOTO/NRST' key again will bring up the alphanumeric navigation screen to 'go to' that airfield. However, and this is a big however, the GPS indicates the direct route to the selected airfield not the safe route nor the route that avoids controlled airspace or restricted areas — though the device should warn when the aircraft is approaching such areas. The GPS does not take into account the type of terrain or the height of terrain — the GPS indicated route might be over 'tiger country' or straight through a mountain. The 'GO TO' function is for emergency use; you must not use it as a substitute for proper route planning. 4.10.5 GNSS constraints Antenna placement. The capability of a handheld receiver is greatly reduced if the receiver antenna is sited where it is shadowed by the airframe or is within one metre of a VHF antenna. An externally mounted antenna usually provides the best reception. Interference. Ensure that (1) the mounting/placement of the GPS unit, and associated cables, within the cockpit can cause no interference with the magnetic compass; and (2) other equipment within the cockpit (including mobile phones) can't interfere with the GPS receiver. Satellite signal quality [SQ]. The SQ number is an indication of signal to noise ratio for each satellite in view: 0–1 is useless, 2–3 is undesirable and 7–9 is good. The SQ may be indicated as an unnumbered bar chart but the scale usually reflects the 0–9 range. Horizontal dilution of precision [HDOP]. Some handhelds may show the HDOP value reflecting the relative geometric positioning of the satellites in view. Low HDOP (less than 02) is best, high HDOP (greater than 06) is not so good for accuracy. Ease of use. The keypads of aviation handhelds are not designed for entering data whilst flying a light aircraft, thus it is very difficult to change route details whilst airborne. Some GPS receivers now on the market, purporting to be aviation receivers, seem to have been designed for the much larger road vehicle market. Improper use of the 'go to' function. There is always the temptation to use the 'go to' function as a replacement for proper flight planning. Further reading Airservices Australia's document 'Safety_Net: using GPS as a VFR navigation tool' concerning avoiding airspace infringements while using GNSS. An excellent online book written by John Bell of Orlando, Florida titled 'A practical guide to using GPS in the cockpit'. The book is in pdf format (4 MB), and also contains links to additional material. It was published in 2006 (developed from an earlier publication) and updated November 2007. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.9.1 Non-directional beacon In Australia the non-directional beacons [NDBs] are the oldest established and, though technically obsolescent, still the most common radio navigation aid. The beacons are usually located at or near an airfield (they were originally called homing beacons), although a very few are still sited to mark waypoints along air routes. The reason they are called 'non-directional' is that the aural radio ranges they originally replaced had directional antennas. The NDBs transmit an omni-directional carrier signal in the low frequency band between 190 and 535 kHz. Their effective range is primarily dependent on the operating power. Most inland NDBs have a transmitter power between 100 and 500 watts providing a range, during daylight hours, usually between 40–100 nm but tending toward the lesser figure. The rated coverage of each NDB is shown in the ERSA entry for the airfield or waypoint. Low-power NDBs, known as 'locators' with a range of 30 nm or less, are sited around major airports and are associated with their instrument landing systems [ILS]. There are also high-power (2–3 kW) NDBs sited near the coast to provide guidance for overwater routes, their over water range being much greater than their inland range. Identification The carrier wave is transmitted on a specific frequency, but a two- or three-letter Morse code signal is continually superimposed on the carrier for NDB identification. The frequency and identification for each beacon is given in ERSA and shown on VNCs, ERC-Ls and VTCs. Some NDBs may provide an intermittent 'voice-over' facility for airfield information. If you want to practise, the morse code dots and dashes are rendered phonetically as 'dits' and 'dahs'. If a Bureau of Meteorology automatic weather station [AWS] is located at an aerodrome that also has an NDB then an aerodrome weather information service [AWIS] may be available at that station. The NDB installation includes voice modulation on the carrier to broadcast the actual, or recent, weather conditions. For further information consult AIP GEN 3.5 para 7.4. AM broadcast stations The companion airborne system which makes use of the NDBs — known as automatic direction finding [ADF] equipment — can also receive transmissions in the 520 to 1611 kHz AM broadcast band. The power output of the broadcast stations is usually considerably greater than NDBs — typically 2, 5, 10 or 50 kilowatts — so their signal can be received at a greater distance. The transmission frequency, power and latitude/longitude of broadcast station transmitters are shown in the NAV/COMM section of ERSA. Broadcast stations must be used with caution because of identification problems. There are long intervals between station identification calls and even then the transmitter to which you are tuned may be relaying programs from another station. The information contained in ERSA may not be up to date. 4.9.2 ADF equipment The ADF, or radio compass, equipment consists of an antenna system, a receiver/control box system and a panel-mounted indicator instrument. The antenna system comprises a loop antenna and a sense antenna which, depending on the age of any particular unit, may be completely separate or combined into one unit. The ADF receiver includes the frequency selector (probably 190 to 1799 kHz) and usually some test capability. The loop antenna nowadays may be a fixed square ferrite core with two perpendicular windings and may be coupled with a goniometer — (a device for measuring angles, with a great number of scientific applications) — in the receiver. Such a system automatically ascertains the direction of the transmitter relative to the longitudinal axis of the aircraft. Hence the reason for the term "Automatic" DF because in earlier days the loop antenna was a physical loop (mounted on top of, or beneath, the fuselage and often enclosed in an egg-shaped fairing) which, simply put, had to be manually rotated by the operator to find the direction of the transmitter, which was read off a scale. At that time, and later, the sense antenna was a wire from the top of the tail fin to a fuselage connection. The output from the receiver is fed to the panel-mounted instrument, which is a needle indicating the direction to the NDB, or broadcast station, as an angle relative to the aircraft's longitudinal axis. Behind the needle is a circular card marked off in 5-degree azimuth divisions from 0° to 355°, with a mark at the top dead centre [TDC] indicating the aircraft's nose. Depending on the age of the instrument, that card may be fixed — in which case 0° is always at TDC — or, more commonly, manually rotatable by turning a heading knob on the instrument. If the card is rotated so that the aircraft's current magnetic heading is situated at TDC then the head of the needle indicates the magnetic track to the transmitter and the tail of the needle indicates the reciprocal bearing — the aircraft's magnetic bearing from the station. When using the ADF indicator it should be normal practice to adjust the card whenever the aircraft's heading is changed. The illustration shows the ADF instrument with the heading knob [HDG] rotated so that the aircraft's heading of 350° magnetic is at TDC; the needle head indicates the track to the NDB is 155° magnetic while the bearing from the transmitter is 335°. However, whether the card is fixed or rotatable, the head of the needle should always point directly to the transmitter and the angle (the number of degrees) between TDC and the head of the needle is always the angle between the fore and aft axis, and the direction of the transmitter. In the illustration that angle is 10 + 155 = 165°. Heavier aircraft are usually fitted with a more complex and very expensive form of ADF called a Radio Magnetic Indicator [RMI], which incorporates, or is slaved to, a directional gyro. It may also have a two-needle display, the second needle being tuned to another navigation aid which, of course, makes position fixing remarkably easy. 4.9.3 ADF applications There are several applications for the ADF in light aircraft cross-country VMC navigation — remembering the Visual Flight Rules require that the pilot must be able to navigate by reference to the ground, and position fixes must be taken at least every 30 minutes. The applications briefly described below will be detailed in the 'Using the ADF' module. Position fixes. If two (three is best) transmitters are in range then the bearing from each can be ascertained, the lines of position roughly plotted on the chart (after converting to true bearings) and the aircraft position will be close to the intersection point. In most of Australia, to have two NDBs in range at the same time is not so common and three would be most unlikely, so the most likely position-fixing use is to combine a surface line feature with an NDB bearing. Running fix / distance from NDB. The 1-in-60 rule can be applied when the aircraft is within range of a transmitter by turning the aircraft so that the station is abeam and then measuring the degrees traversed against time. This is a form of running fix in that two bearings are taken, at an interval, from one source and the aircraft's position is the distance along the second LOP from the NDB. For example: Distance (nm) to NDB = elapsed time (mins) × ground speed (kn) / degrees traversed Homing and tracking to or from an NDB. If there is no crosswind component then tracking toward an NDB is quite simple — just keep the head of the ADF needle at TDC and you will arrive overhead; the track over the ground will be straight and the magnetic heading constant. However, if there is a crosswind component and you just endeavour to keep the head of the ADF needle at TDC, you will eventually arrive but, due to the drift, the track followed will be curved and the magnetic heading will need to be consistently changing. This is called homing, and you will arrive at the NDB on an into-wind heading. Thus tracking, or flying directly towards, or from, an NDB is exactly the same as tracking from A to B — you have to calculate a wind correction angle. Passing overhead an NDB is signified by a "cone of silence" (if the 'ident' volume has been turned up beforehand) and the needle then swings to the reciprocal bearing. Using the ADF probably appears to be fairly simple, which it is, but there will be difficulties — for the uninitiated in perceiving, from the position of the needle, the headings to fly when attempting to intercept and then track along a particular magnetic bearing to or from the ground station. As in all navigation you should always maintain an awareness of the aircraft's position in terms of being north, south, east or west of the NDB and, when initiating a turn, think in the same terms; e.g. a left turn will take you further east. 4.9.4 NDB/ADF errors Electrical interference. Radio waves are emitted by the aircraft alternator in the frequency band of the ADF. An alternator suppressor is fitted to contain those emissions but this component does not have a long life and it is wise to test the ADF for correct operation during pre-flight checks. The test is made by selecting a transmitter — which must be a reasonable distance away, say 30 nm – then watch the ADF needle during the engine run-up. If the needle moves as rpm increases there is electrical interference and probably the alternator suppressor should be replaced. Magnetos may also interfere with the ADF. Thunderstorms emit electrical energy in the NDB band and will deflect the ADF needle towards the storm. Twilight/night effect. Radio waves arriving at a receiver come directly from the transmitter — the ground wave — and indirectly as a wave reflected from the ionosphere — the sky wave. The sky wave is affected by the daily changes in the ionosphere; read the ionisation layers section in the Aviation Meteorology Guide. Twilight effect is minimal on transmissions at frequencies below 350 kHz. Terrain and coastal effects. In mountainous areas NDB signals may be reflected by the terrain, which can cause the bearing indications to fluctuate. Some NDBs located in conditions where mountain effect is troublesome transmit at the higher frequency of 1655 kHz. Ground waves are refracted when passing across coast lines at low angles and this will affect the indicated bearing for an aircraft tracking to seaward and following the shore line. Attitude effects. The indicated bearing will not be accurate while the aircraft is banked. 4.9.5 VHF Omni Range In Australia the VHF omni-directional radio ranges [VORs] operate in the Very High Frequency aviation navigation [NAV] band between 112.1 and 117.9 MHz. As VHF transmissions are line-of-sight, the ground-to-air range depends on the elevation of the beacon site, the height of the aircraft and the power output. The VOR beacons are usually located at airfields but as they serve to define designated air routes (airways) some are located away from airfields, often on high ground. A simplified concept of the ground beacon is that it simultaneously transmits two signals, a constant omni-directional signal called the reference phase and a directional signal which rotates through 360°, during a 0.03 second system cycle, and consistently varies in phase through each rotation. The two signals are only exactly in phase once during each rotation — when the directional signal is aligned to magnetic north. Imagine a wheel with 360 spokes, at one degree azimuth spacing, with the VOR beacon being the hub. The spokes are numbered clockwise from one to 360 and each spoke or radial represents a magnetic bearing from the VOR beacon. The airborne navigation circuitry measures the phase angle difference between the directional signal phase received and the reference signal phase, and interprets that as the angular, or 'radial', indication currently being received. Radials are identified by magnetic bearing — e.g. the 30° radial — and thus form the basis for VOR and designated air route navigation. Essentially the system indicates a line of position from the selected VOR, on which the aircraft is located at any time. The beacon also transmits a Morse code aural identification signal at about 10 second intervals. The airborne system utilising the VOR beacon transmissions usually consists of an antenna (probably a V-type dipole mounted horizontally on the fin or fuselage, but could be the more expensive 'blade' or 'towel rail' types), a conventional VHF receiver (if combined with the VHF communications transceiver it is then called a NAV/COMM unit), navigation circuitry and the separate panel-mounted navigation indicator or 'omni bearing indicator' [OBI]. Some hand-held aviation COMMS transceivers can also receive the NAV band VOR transmissions and appear to have some navigation circuitry but, from all reports, their VOR navigation capability, if it exists at all, is limited. A basic OBI , like the above Bendix-King model, has a manually operated radial or 'omni bearing' selector [OBS] that rotates an azimuth ring marked from 0° to 355°. The OBS selected radial is indicated by the arrow at top dead centre and the reciprocal bearing is indicated by the bottom arrow. The other features of a basic OBI are the TO–FROM indicators, a deviation bar, a deviation indicator needle and a NAV/OFF alarm flag. The TO–FROM logic The TO–FROM indications on the OBI are dependent on the aircraft's position relative to a notional ground baseline, formed perpendicular to the selected radial and passing through the beacon site. Unlike the NDB the indication is completely independent of the aircraft's heading. The navigation circuitry compares the difference between the radial being received and the radial selected. If the aircraft is located anywhere within range on the radial side of the baseline, the 'FROM' indication will be displayed on the OBI and, if located within range on the reciprocal side, the 'TO' indication will be displayed. For example if the 030° radial is selected on the OBI, the ground baseline is established between 300° and 120°. If the radial received indicates the aircraft is anywhere in the blue shaded area of the diagram and no matter whether it is headed towards or away from the VOR, or in any direction whatsoever, the OBI will display 'FROM'. Similarly if it is in the yellow area the OBI will display 'TO' no matter which direction the aircraft is headed. There are two areas of ambiguity – near bearings at right angles to the radial (e.g. shown at 120° and 300°) – where the OBI will give fluctuating indications, or display the 'OFF' flag. The course deviation indicator The deviation bar and the deviation indicator needle together form the course deviation indicator [CDI]. If the needle is over the centre point the aircraft is then located at some position along the selected radial — or its reciprocal. The five division marks or dots either side of the centre point are spaced at two-degree intervals, thus if the needle is over the third mark, left or right of centre, the aircraft is positioned at a radial six degrees in azimuth from the selected radial, or its reciprocal. (Actually the aircraft is at the centre mark and the needle indicates the position of the selected radial). Full travel of the needle from the centre to either side represents 10° or more of azimuth. The ambiguity of whether the OBS selection is the radial or the reciprocal is determined by the TO/FROM indication; in the diagram at left 030 must be the radial, as the aircraft is in the FROM area. When the aircraft passes overhead the beacon, the needle will swing from side to side, the alarm flag may temporarily indicate that navigation is 'OFF' and the TO/FROM indication will reverse. A difficulty for a non-IFR trained pilot using the VOR is a lack of perception of which way to turn the aircraft to fly to a selected radial, using the CDI indications. However, for VFR purposes, this is easily ascertained if the pilot follows two simple rules: 1. To track FROM a VOR select the radial required and ensure FROM is indicated. 2. To track TO a VOR rotate the OBS until the CDI is centred and TO is indicated. In both cases as wind effect drifts the aircraft off track the deviation indicator needle will move to one side and that movement indicates the direction to turn to regain track. i.e. turn towards the needle. 4.9.6 VOR applications Like the NDB/ADF there are several applications for the VOR in light aircraft cross-country VMC navigation. The applications are briefly described below. Homing and tracking to a VOR. Even with a crosswind component, tracking toward a VOR is quite simple — rotate the OBS until the CDI is centred and TO is indicated, turn onto that magnetic heading and then just keep the CDI centred and you will track more or less directly to the VOR. Tracking from a VOR. Rotate the OBS to the required track (radial), ensure FROM is indicated, turn onto that magnetic heading and just keep the CDI centred and you will maintain the track. Position fixes. If two VORs are in range then the bearing from each can be ascertained, roughly plotted on the chart (after converting to true bearings) and the aircraft position will be close to the intersection point of the LOPs. Alternatively a VOR bearing and a NDB bearing can be used, or a VOR bearing and a line feature on the chart — the latter technique being the most frequently used. Running fix/distance from VOR. The 1-in-60 rule can be applied when the aircraft is within range of a transmitter by turning the aircraft so that the station is abeam and then measuring the degrees traversed against time, as in the NDB running fix application above. The advantage with the VOR is that the CDI needle indicates the degrees traversed. As in the NDB application, the position fix is the distance along the second radial from the beacon. VOR simulator If your browser is Java enabled then I suggest a visit to www.fergworld/training/ and try out the single VOR trainer applet. Drag the aircraft symbol to position your aircraft, set the aircraft's heading on the directional gyro, rotate the OBS to a desired radial and check the CDI and flag. Then try to intercept the radial and track to or from the VOR, by changing heading and dragging the symbol along the flight path.Try the quiz. 4.9.7 VOR errors Standard VOR systems are more accurate than NDB/ADF but are still subject to errors at the ground station, bending distortion of signals caused by terrain effect and avionics errors. The aggregation of all errors is very unlikely to exceed 5°. Though very thin on the ground in the outback areas of Australia, NDB and VOR can be very useful, provided the aircraft is within range, but not the best value for money. That distinction now belongs to another, and more advanced, supplementary navigation tool — the Global Positioning System. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.8.1 Setting compass heading Before we reach the set-course point of our flight we have to determine the compass deviation known to occur at that magnetic heading — if the deviation has been measured and if there is a compass correction card displayed in the aircraft. Such a card might look like this, although it is more likely to be expressed in 30° rather than the 45° intervals shown: Compass correction card Heading magnetic 045° 090° 135° 180° 225° 270° 315° 360° Compass correction +2° +5° +2° 0 -2° -5° -2° 0 To find the heading to set on the compass just add the deviation value to, or subtract it from the magnetic heading. For example, our heading on the flight from Oxford to Warraway Mountain is 079° magnetic. Interpolating between the values shown for 045° and 090°, the correction for 079° is +4°. Thus the compass heading to steer is 083°, the magnetic heading will then be 079° and the true heading will be 090°. 4.8.2 Monitoring and recording flight progress Position fixing methods Monitoring involves checking from clock to map to ground — anticipating what should be in view a few minutes ahead — plus intermittent position fixing to establish the track made good, and estimating the track error and the actual ground speed. The essential navigation instruments are just the compass and the clock — to provide direction and ground speed. Deviations from the track required occur because: the pilot is not maintaining the planned heading or has set the wrong heading; e.g. the heading for a different leg. unrecognised compass error (deviation) causes the heading flown not to be the planned magnetic heading. There should be no extraneous ferrous materials (e.g. the rings on a binder) near the compass, or larger items anywhere in the cockpit. the wind velocity is substantially different from that used for the flight plan or it was applied incorrectly during flight planning the required track direction was incorrectly measured — or converted to magnetic — during flight plan preparation. In eastern Australia, where magnetic variation reaches 13°, reversed application of variation when calculating the magnetic heading can result in a very large heading error. There are basically two methods of fixing the aircraft's position. The first and the most common in light aircraft navigation is by identifying a landmark close to the aircraft. The second is by identifying (or establishing) two or more lines of position [LOP] such that their point of intersection provides the position fix. A line of position is a line drawn, or an existing line feature, on the chart indicating that the aircraft's position is somewhere along it. Note that there should be a reasonable angular difference, maybe more than 30°, between two LOPs in order to derive a useful position fix. A LOP might be: a clearly identifiable physical identity such as a long road, railway or river that the aircraft is about to fly across — but without any supporting indication of exactly where the crossing is being made a visual bearing from an identifiable landmark. Or an instrument bearing from a radio beacon, which we will discuss in the 'Supplementary navigation techniques' module a transit. When two identifiable landmarks are seen to be in line with each other the aircraft must be somewhere on the extended sight line. It is a good practice to identify suitable landmark pairs in the flight planning stage, and mark on the chart the transit line between them, extending it across the track required — thus pre-marking the LOP. It is not always possible to derive two LOPs, more or less simultaneously, to get a position fix at their intersection. However, if a second LOP can be derived within, say, 10 or 15 minutes of the first then a running fix can be ascertained. This is done by estimating the distance flown along the track during the lapsed time and then shifting the initial LOP that distance. The intersection of the shifted line and the second LOP is the position fix. (There is another form of running fix involving two bearings from the same object but this is really a technique applicable to radio navigation aids and we will look at it in the 'Supplementary navigation techniques' module.) Recording progress An accurate running log of LOPs or position fixes, segment start and finish times, magnetic headings, and fuel consumption must be maintained — it is very difficult to remember times and headings flown — and the log need only be a blank note pad. For example the first note made is the engine start-up time and the second is the set heading time. The minutes elapsed between those times is then noted on the fuel log 'Airfield departure' line in the 'Actual' column. All events logged should be preceded by the time and all the magnetic headings flown must be logged, even when that heading is the planned heading. As each route segment is commenced, log the ETA for the next waypoint or major landmark, which will be the current time plus the flight plan ETI (or an adjusted ETI) for that segment. As each route segment is completed, the elapsed time is transferred to the fuel log. 4.8.3 Track error adjustments The track plot below shows the first leg of our planned flight between Oxford and Tottenham. The green lines are the 10° drift lines and the pink marks along the track are the 10 nm distance marks. In this example the first three marks are distance from Oxford, the last three are distance from Warraway Mountain, and the longer mark is the track midpoint. Instead of distance marks some navigators favour time marks at, say, 10-minute intervals. However time marks really don't correlate that well with charts — time notations should be shown in the log. As it is remarkably easy to set off in the wrong direction — reversed application of variation for instance — it is advisable to note a landmark as a means of verifying that, at the set-course point, you really are setting off along the required track. The sun's position provides a gross indication of heading, which will at least confirm that you are not flying the reciprocal course. Starting off in the wrong direction, without realisation, really makes progress monitoring difficult when you are flying over relatively featureless terrain. In addition, a position fix must be acquired within 15 to 20 minutes of the set heading time. At any time after departure, when the aircraft's position has been pinpointed and found to be off track, heading adjustments will be necessary. Initially this is to regain the required track and then to maintain it; or, alternatively, for a new heading to track directly to the next turning point. There are several methods for calculating and applying heading adjustments — a pencil and paper will be handy. Double track error method This is the recommended method if the position fix shows the aircraft to be less than halfway along the leg — hence the reason for marking that midpoint on the chart. The procedure is as follows: 1. Using the diverging 10° drift lines estimate the track error — the difference in degrees between the track required and the track made good. The track error is also referred to as the opening angle or sometimes as the drift angle. (The term 'drift angle' in this context is deprecated, as it normally refers to the angular difference between the heading flown and the track made good.) For example let's say, on our Oxford to Warraway Mountain segment, we find ourselves crossing the railway line at Trida and estimate the track error as 6° north of required track; i.e. the track made good is 077° magnetic. We log the time and note 24 minutes have lapsed since departing the set-course point. 2. Double the track error and add or subtract that value from the planned heading to arrive at the heading to regain track or the intercept. If the drift was to the right of track the new heading must be to the left of the original heading and, conversely, if drift was to the left the new heading must be to the right of the original. The time we must remain on this new heading, until intercepting the required track, is roughly equivalent to the time flown on the original heading. Although we have used the terms 'left' and 'right' you will find it more helpful, when considering position, to think in terms of 'north', 'south', south-west' etc. For example track error is 6° north (left) and original heading 079° magnetic, thus the heading to regain track is 079 plus 12 = 091° magnetic (096° compass) and we fly that for 24 minutes — the same time as that flown on the original heading. 3. After it is visually evident that the required track has been reached, or the required time has passed, subtract the track error and turn onto the new heading to maintain the required track and log the event. For example the track error was 6° and the heading to regain track is 091° magnetic, thus the heading to maintain track is 091 minus 6 = 085° magnetic (090° compass) and we fly that until either a new position fix is obtained or we reach the waypoint. Theoretically this method doesn't work if the position fix is past the halfway point, because the point at which the required track is finally regained would be past the waypoint. Track error/closing angle method The recommended method if the position fix shows the aircraft to be more than halfway along the leg, or if you choose to fly directly to the waypoint at any time, is as follows: 1. Using the diverging 10° drift lines estimate the track error or opening angle — the difference in degrees between the track required and the track made good. Then using the converging drift lines estimate the direct track to the waypoint, and the angle between that track (the new required track) and the original required track. This is usually called the closing angle. For example, let's say on our Oxford to Warraway Mountain segment we fix our position as one mile south of the Dundooboo Ridge with an estimated track error of 7° north of required track and the closing angle to the waypoint is about 9°. 2. Add the track error and closing angle, and apply the value as a correction to the original heading. If the drift was to the left of the required track the new heading will be to the right and vice versa. For example, 7 plus 9 is 16°, drift was to the left of the original heading 079° magnetic, thus the heading — to track directly to the waypoint — is 095° magnetic (100° compass). Flight direct to landmark A third method might be employed if after getting a position fix a landmark known to be on, or close to, the required track is positively identified. • Having pinpointed your position use the diverging 10° drift lines to estimate the track error, then fly directly to the identified on-track landmark. To maintain the required track it will be necessary to turn onto a new heading when overhead the landmark. The new heading will of course be the original heading plus/minus the track error. Utilising the 1-in-60 rule The 1-in-60 rule of thumb can be used to determine track error, given distance travelled and distance off track. It replaces the use of drift lines but the latter is much the easier method to use in flight because the angle is easier to estimate than the on-chart distance off track, and the mental arithmetic is easier. However, just to keep you informed, here is the 1-in-60 method for track error calculation. 1. Having pinpointed the aircraft's position, estimate the distance off track and the distance travelled along the leg. The track error = the distance off track [DO] divided by the distance travelled [DT] × 60; i.e. the track error = DO/DT × 60. Or conversely the distance off track [DO] = track error/60 × DT For example, let's revert to our Oxford to Warraway Mountain segment where, after 24 minutes flight, we pinpoint our position at Trida. Trida is about 3 nm north of required track and 22 nm distant from the departure point. Thus 3/22 × 60 = 8° track error. The track error we estimated using the drift lines was 6°, but that's basic navigation for you. 2. To regain the required track double the track error and when the required track is reached, or the time has elapsed, subtract half the error and take up the new heading. In this aspect it's the same technique as the double track error method. 3. Or to track direct to the next waypoint calculate the closing angle — which will equal the distance off track [DO] divided by the distance to go [DTG] × 60; i.e. closing angle = DO/DTG × 60. For example, Trida is 52 nm distant from Warraway Mountain. Thus 3/52 × 60 = 3° closing angle. The new heading is the original heading plus track error plus closing angle = 079 + 8 +3 = 090° magnetic — as in the track error/closing angle method. Each time the aircraft's position is pinpointed and the heading is adjusted, a re-calculation of the ground speed and ETI for the segment should be made on the running log. The 1-in-60 rule has use in this aspect of navigation, see 'Re-assessing wind velocity'. Diversions — 30° and 60° dog-legs There is another navigational adjustment technique occasionally mentioned as a standard method of diversion around hazards or no-fly areas such as towns. As no such diversions would be necessary if the flight plan is properly prepared, such techniques should be of practical use only when something of interest is spotted off track and you divert for a little sight-seeing. The method is as follows: • When you judge the point of interest is about 30° off your current heading, alter course 30° towards the target. Note the time when the target is reached and then turn 60° in the reverse direction and fly that heading for the same time as the first part of the dog-leg, then revert to the original heading. The time lost during the two legs (which has to be added to the ETI for the segment) is one third of the time flown on the first (or either) leg and, of course, you have to add to the ETI any time spent circling over the target. • The 60°dog-leg is much the same except that you alter course when the target is 60° off the original heading, the alteration to return is 120° and the time lost flying the dog-leg is the time spent on the first (or either) leg. For example, if we were about halfway along our track from Oxford to Warraway Mountain, we thought it a good idea to have a look around the Warranary Hill. Thus we turn 60° left from our original heading of 079° magnetic to 019° magnetic, fly that for, say, six minutes then turn right 120° to 139° magnetic and fly that heading for six minutes. Then turn left 60° back on to our original heading of 079° and add six minutes to our ETI and/or ETA. 4.8.4 Recalculating ETI/ETA and fuel consumption Checking ground speed Being off-track, because of a variation in wind, is much more likely to be noticed quickly than if on track but adversely affected by a stronger than expected headwind, or tailwind. Whenever a position fix is obtained, whether it is on-track or off-track, it is advisable to calculate the ground speed achieved and to re-estimate the ETI for the leg, ETA at the next waypoint and at the destination. Ground speed [GS] in knots is distance travelled [DT] / elapsed time in minutes [ET] × 60; i.e. GS = DT/ET × 60. For example, reverting to our Oxford to Warraway Mountain segment where, after 24 minutes flight, we pinpoint our position at Trida, 22 nm distant from the departure point and about 52 nm from the next turning point. Ground speed = DT/ET × 60 = 22/24 × 60 = 55 knots. Recalculating ETI Time in minutes to the next checkpoint, turning point or destination = distance to go [DTG] / GS × 60; i.e. time = DTG/GS × 60. e.g. Time to the next turning point = DTG/GS × 60 = 52/55 × 60 = 57 minutes. There is a slightly simpler means to estimate the time to the next turning point. A ground speed of 60 knots is one nm per minute so at 60 knots the distance of 52 nm will be covered in 52 minutes. However, our ground speed of 55 knots is about 10% less so the time will be 10% greater than 52 = 57 minutes. Our ground speed at 55 knots is nearly 20% less than the flight plan figure of 67 knots and our ETI for the first leg is now 57 + 24 = 81 minutes; some 15 minutes — or more than 20% — greater than the flight plan figure of 66 minutes. This decreased performance is most likely caused by an easterly wind speed much greater than the forecast. The summed flight plan en route time was 144 minutes and 20% of this is about 30 minutes — which makes a significant inroad into our 40-minute above-reserve fuel margin. We will have to closely monitor progress, as we are getting near to the point of considering diversion to an alternate airfield. Re-assessing wind velocity The headwind component of the wind speed must be our true airspeed (75 knots) minus our ground speed (55 knots); i.e. 20 knots. However, the crosswind component is difficult to estimate because we don't know what caused the drift away from the track required. It could be unadjusted compass deviation, poor heading holding or changed wind velocity — or a combination of all three. However, let's assume we found ourselves over Trida only because the wind varied substantially from that assumed in the flight plan. If so, what is the crosswind component? To measure the crosswind component we need to measure the drift angle — the angular difference between the heading flown and the track made good [TMG]. For example, at Trida TMG was estimated as 6° left of the track required (083° magnetic) thus 077° magnetic and the heading flown was 079° magnetic. The drift angle is then 2° left, rather than the expected 4° right. Using the 1-in-60 rule, the crosswind component in knots = the drift angle/60 × TAS in knots. For our Trida position fix, the crosswind component is thus 2/60 × 75 = 2.5 knots. We can now estimate the wind direction by first ascertaining the ratio of crosswind to headwind, which in this case is 2.5/20, and then, using the 1-in-60 rule, calculate the wind angle relative to the aircraft's heading. The equation is: wind angle = crosswind/headwind × 60 = 2.5/20 × 60 = 7°. As the drift recorded was to the left, the wind must be coming from 7° to the right of heading, the heading being flown was 079° magnetic thus the wind direction is 086° magnetic or 097° true and its speed must be very close to 20 knots. Summary of track angle definitions Track error — the angular difference between the track required and the track made good. You may come across the term cross track error [XTE] which refers to the distance off track. Opening angle — another term for angular track error. Drift angle — the angular difference between the heading flown and the track made good. Closing angle — the angular difference between the direct track to the waypoint and the original required track, measured at the waypoint. Wind angle — the angular difference between the heading flown and the estimated wind direction; i.e. the wind relative to the aircraft rather than the ground. Checking fuel consumption Fuel flow indications are monitored for abnormalities as part of the continuing in-flight instrument scan. A calculation of consumption rate should be made at half-hourly or hourly intervals to check for any significant variation from the hourly consumption rate used in the flight plan. Fuel consumption should always be measured in terms of time not distance. 4.8.5 Diverting to an alternate airfield During flight the pilot should always be aware of the general direction of the planned alternate airfields so that, should a diversion be deemed necessary, the aircraft can then — after verifying current position — be headed in the general direction of the selected alternate without unnecessary delay. The mental calculations required to refine the heading, estimate distance, ETA and fuel requirement are then done without wasting time and fuel. For example, let's say that we reach our turning point at Warraway Mountain 85 minutes after the set heading point; i.e. the actual time interval [ATI] is 19 minutes, or nearly 30%, greater than our ETI of 66 minutes. Under these circumstances we could expect that the total en route time would also increase by 25% to 30%, even though the next two legs are swinging out of wind by 30° or more. This adds around 40 minutes to total time and reduces the planned fuel margin above reserve to zero so a diversion to Condobolin is warranted. The bearing is about due east so we would note the time and alter heading slightly to 079° magnetic. Looking at the chart the distance from Warraway Mountain to Condobolin is easily estimated (knowing that the distance between the meridians on the grid of this WAC is 25 nm) at about 55 nm and the bearing is not quite due east, say 088° true. Our ground speed will not alter from that established between Oxford and Warraway Mountain where we covered the distance of 74 nm in 85 minutes — 74/85 × 60 = 52 knots. The ETI from Warraway Mountain to Condobolin will be 55/52 × 60 = 63 minutes and our fuel log will look something like this: Fuel calculation and fuel log Cruise fuel flow: 16 litres/hr Usable fuel loaded: 64 litres Endurance: 240 mins Estimate Actual Airfield departure: 10 mins 12 Climb penalty: 6 mins 6 En route: Oxford – Warraway: 66 mins 85 Warraway – junction Cond'n: 48 63 mins Junction – Tottenham: 30 mins Estimated time en route: 144 148 mins Airfield arrival: 10 mins Fixed reserve: 30 mins Total fuel required: 200 206 mins Fuel margin (endurance –total required) 40 34 mins 4.8.6 Line-of-sight distance and landmarks Knowledge and use of landmarks is an essential part of light aircraft pilotage, thus on cross-country flights it is useful to know at what distance any landmark, particularly those distinguished by height and shape, might be discernible. The rule-of-thumb is, given unlimited visibility and an eagle eye, the maximum optical line-of-sight (LOS) distance, in nautical miles, is equal to the square root of the observer's height in feet. More precisely, it is 1.06 times the square root of the height. By the way, VHF transmissions are also LOS. Theoretical LOS distance to horizon Observer height (feet) Maximum LOS distance (nm) 10 3.2 100 10 1000 32 10 000 100 The theoretical distance in nautical miles at which a landmark may be seen is near enough to the sum of the square root of the height of the top of the landmark (in feet) and the square root of the observer's height. Theoretically then a pilot flying at 10 000 feet might first see the highest point of an island, with an elevation of 1000 feet, from 132 nm away (100 + 32). However, in cross-country flight, the only landmarks readily discernible at long distance are hills or mountains, particularly sentinel types. For example, in south-eastern Australia, Mt Ulandra, Mt Major and The Rock. Even then, for firm identification, you may need to have the top few hundred feet in view and be less than the maximum distance from the landmark. Haze, residual dust and smoke greatly reduce visibility, particularly below inversions and in the friction layer. When doing the calculation for LOS distance the basic elevation of the general intervening terrain must be deducted from the elevation of the landmark — and from the observer's altitude. In the table below, The Rock has an elevation of 1800 feet and the intervening terrain elevation is 800 feet, so the top 500 feet of the sentinel begins 500 feet above the general terrain. The third column of the table shows the LOS distance from observer height above the terrain, the fourth column shows the LOS distance from a point 500 feet below the summit and the last column — the sum of columns three and four — shows the maximum distance at which all the top 500 feet might be seen, above the horizon, by an observer at a recommended light aircraft hemispherical cruising level. LOS distance to sentinel landmarks Observer altitude Observer height above terrain Observer LOS distance The Rock top 500 ft LOS distance Distance at which The Rock visible feet feet nm nm nm 1500 700 27 23 50 2500 1700 42 23 65 3500 2700 52 23 75 4500 3700 61 23 84 An eagle-eyed observer flying at 4500 feet, on a remarkably clear day, could see The Rock, well above the horizon, from as far as Young, Griffith, Deniliquin or Benalla. If you were heading for Yabba North, at 3500 feet, you could see Mt Major (nine miles south of Yabba) from Culcairn, Jerilderie or Bendigo. Estimating the square root: mental calculation is easier if you ignore the two least significant digits of the height, then estimate the square root of the remaining one or two digits and multiply by 10. For example; height 3000 feet, ignore 00, the square root of 30 is between 5 and 6 — say 5.5 and multiply by 10 = 55 nm LOS distance. Another example; height 700 feet, ignore 00, the square root of 7 is between 2 and 3 — say 2.5, multiply by 10 = 25 nm LOS distance. 4.8.7 Procedure when lost There are occasions during a cross-country flight when the pilot is uncertain about the aircraft's position, particularly when there are considerable distances between verifiable landmarks and a near-track landmark has not come into view. If proper flight planning and checking procedures are followed, and actual versus planned flight progress is continually monitored and recorded, then probably the only way to become really lost — in fine weather and reasonable visibility — is if an en route heading adjustment is incorrectly calculated or implemented, or if a turning point is overflown without noticing. There are a few rules that must be followed if thought to be lost or caught in a difficult situation; 1. Fly the aeroplane! You must not concentrate all attention on the navigation problem — keep the normal scan going otherwise you can readily lose control of the aircraft. 2. If the ETA at the next waypoint has not yet, or only recently, lapsed then hold the heading — resist the temptation to start wandering about searching for landmarks. 3. However, if the ETA at the waypoint has long passed then choose a landmark below the aircraft, log the time and then orbit the landmark while you carry out a quick recheck of the running log and previous mental DR, and start the procedure detailed in the next paragraph. But don't forget rule 1: "Fly the aeroplane!". There is no point in wasting fuel while doing this so reduce power and airspeed to the best endurance setting for a safe flight speed. If no obvious error is found that will provide the basis for a position estimate then proceed with rule 4. 4. Check the time elapsed since the last position fix and estimate the distance covered in that time. On the chart draw a line of position [LOP] across the track (the original or an intercept) at the estimated distance from the last fix. The line should extend about 1 nm either side of track, for each 5 minutes flown since the fix; i.e. if it is 30 minutes since your last positive fix then the line will extend roughly 6 nm either side. Then draw a rough circle with the LOP as the diameter (see diagram below) — your most probable position [MPP] is somewhere within that circle of uncertainty. Find the most prominent features on the map within the circle and then try to locate them on the ground. The 1 nm per 5 minutes is based on ground speeds around 50 or 60 knots; if ground speeds are around 100 knots then make it 2 nm per 5 minutes. 'Most probable' means maybe an 80% chance. 5. If below 3000 feet agl then climb a little, cloud base permitting. The theoretical line-of-sight distance at 4000 feet agl is 65 nm all round. This provides sufficient coverage to pick up all the major landmarks — near and middle distance — which aren't concealed by terrain or atmospheric conditions. If climbing takes you above an inversion layer you may find surface visibility is better just below the inversion. Remember that on a bright day, scattered cloud shadows may make some landmarks difficult to pick up even if relatively close. Reduce power to best endurance. 6."Read from ground to map!" Normally in flight, the navigator should be continually identifying features on the map and waiting for the next one to come up on track, within an estimated time. When uncertain of position, the procedure is reversed — look for two or more large features on the ground and then identify features on the chart that are in the same juxtaposition. Prominent line features are best although, quite often, a spot feature is easily identified — for example the names of grazing or farming properties are shown on the charts and their owners, particularly those with an airstrip, often paint the name on a roof, in large letters. If you see a prominent line feature, then fly along it until you can derive a fix from an intersect or a verifiable landmark. 7. If necessary "assess the wind!" Whilst over the orbiting landmark turn onto a quadrantal heading, e.g. north, and fly that heading for one minute then turn 90°, e.g. west, and fly that for one minute. Systematically scan the surrounds for an identifiable landmark, starting with the area closest to the aircraft then moving out to the middle distance. Repeat for two more anticlockwise turns and after 4 minutes have elapsed you should arrive back near the starting point. If you have held to the headings and the timing, then the ground distance and direction of the arrival point from the orbiting landmark should provide a reasonable estimate of the wind velocity; e.g. if the arrival point is about 1 nm north-west then the wind speed must be 15 knots from the south-east. Of course if you are a poor judge of ground distance (which applies to many/most of us) then the indicated wind speed is not calculable but at least you know the direction and have a gross indication of the speed. 8."Start an expanding square search!" Starting over the orbiting landmark turn onto a quadrantal heading, e.g. north, and fly that heading for 2 minutes then turn 90°, e.g. west, and fly that for 2 minutes. Log the times and headings. Systematically scan the surrounds starting with the area closest to the aircraft then moving out to the middle distance. Repeat for two more legs but fly these for 3 minutes each. The next 2 legs are flown for 4 minutes each and so the expanding pattern is repeated, extending each pair by one minute, until a position is pinpointed or you are well outside the circle of uncertainty and a precautionary landing might be a wise action. Do not fly around in increasing circles, always fly planned (and logged) headings and durations. 9. "Don't stay up too late!" Be prepared to make a precautionary landing well before the fuel content reaches the 30-minute reserve figure and well before oncoming twilight reduces visibility at ground level. You need to ensure that a precautionary landing isn't downgraded to a forced landing because of fuel exhaustion. Try to select a suitable site near a house. Remember after you have landed you still have to secure the aircraft, protect it from stock (cattle licking the skin do a lot of damage) and perhaps get some help — very difficult in the bush and near impossible in the dark! A 'precautionary' landing is an emergency landing under power at a prepared landing ground or some other suitable, but unprepared, site. If you have read your insurance policy carefully you may find that damages claims are limited if you make a precautionary landing at a 'non-prepared landing zone'. There are many circumstances where a precautionary landing is a wise move. Among them are: occupant illness or a frightened passenger deteriorating weather oncoming darkness fuel reaching reserve level lost and you decide to obtain help on the ground engine running rough (although this might be considered a forced landing.) The technique for precautionary landings at other than a prepared landing ground is essentially the same as that for short field landings except that additional low-level passes should be made to check the hazards, taking particular care in locating and avoiding wires. Map out the landing/run-out path and also determine the escape route in the event of an aborted landing. And lastly: 10."Communicate!" Share the problem. See the next module in the "Coping with Emergencies Guide' — Safety and emergency communication procedures. 4.8.8 Dangers of flight into cloud or when lacking visual references Vestibular system illusions When walking, a person's prime sense of orientation is provided by visual references. When vision is severely degraded, the vestibular system in the inner ears — which senses motion and gravity (thus roll, pitch and yaw) — generally allows us to keep our balance when walking without using visual references. However, the vestibular system is not designed for high speed or angular motion, and cannot be used as an in-flight back-up system; i.e. you cannot close your eyes and continue to fly straight and level. Motion of the fluid within the ears' semicircular canals is affected by inertia and will feed quite erroneous prompts to the brain, resulting in various types and levels of vertigo. For example, without the external visual references of clear sky, terrain or a horizon, forward deceleration tends to give a pitching-down sensation whilst forward acceleration gives a pitching-up sensation. Once settled into a constant-rate turn, the sensation is of not turning at all; but when the turn is halted, the sensation is then of turning in the opposite direction. In addition, the vestibular system will not detect slow rates of bank, so that if the aircraft is banking at the rate of one or two degrees per second the vestibular system will not send any prompts to the brain — it will consider the aircraft is still flying straight and level, while any associated speed changes may provide contrary sensations. For example, if the aircraft is slowly banking and accelerating in a descending turn, the sensation may well be one of pitching-up. Such sensations disorient the pilot. See the effect spiral instability may have on an aircraft and pilot in cloud. Spatial disorientation Aircraft accidents caused by spatial disorientation are usually fatal and occur when VFR flight is continued in adverse visibility conditions — cloud, fog, smoke, haze, showers, oncoming darkness and combinations thereof. Pilots who have not been trained to fly solely by visual reference to the indicators in an 'instrument flight' panel/display will soon find themselves experiencing spatial disorientation should they inadvertently or deliberately enter instrument meteorological conditions [IMC] where the external visual references — by which they normally orient themselves in visual meteorological conditions — are lost. The same applies to any atmospheric condition where the visual references — horizon (principally), terrain and clear sky — are lost or just significantly reduced; see white-out/flat light for example. Thus, a non-instrument rated pilot would be unable to maintain controlled flight in cloud, or maybe even in conditions where the horizon disappears, and even an instrument-rated pilot cannot fly in cloud without the minimum IFR instrumentation. Nor can an instrument-rated pilot in an IFR aircraft fly where the aircraft can't out-climb rising terrain, whether it is concealed or not. In addition many horrific accidents have occurred when an IFR pilot has descended below the area 'lowest safe altitude' in IMC and impacted the terrain; such events are classified as controlled flight into terrain. Note: even a pilot who is well experienced in flying in IMC may occasionally experience a phenomenon called 'the leans'. This might occur when the IFR instrumented aircraft has been inadvertently allowed to slowly bank a few degrees and the pilot then makes a quick correction to level the wings. The vestibular system doesn't register the slow initial bank but does register the wing levelling as an opposite direction bank (away from a wings level attitude) and the pilot's brain produces a leaning sensation while also perceiving from the instrument readings that the aircraft is flying straight and level. The reaction — which can persist for quite a while — may be for the pilot to lean sideways in her/his seat so that everything feels right! 4.8.9 Pressing on in deteriorating conditions Most fatal excursions into IMC by light aircraft seem to occur when the pilot freely elects to find a path through or over high terrain beneath an overlaying cloud cover, in order to maintain a perceived time schedule, but without ensuring that there is a clear way out or back. For example a Jabiru pilot, who held a current RA-Aus CFI approval and had accumulated some 10 000 flight hours experience mostly in commercial general aviation, elected to cross the Great Dividing Range east to west in the northern NSW region after being frustrated from doing so the previous day by low cloud. The aircraft crashed on track, first impacting trees and then the rising terrain of a rainforest covered ridge, approximately 200 feet below the top of the ridge. The aircraft made its initial impact with wings level, travelling on a westerly heading consistent with its track. The aircraft initially contacted a tree some 80 feet agl shearing off the starboard wing then continuing another 100 metres where the port wing was removed by another tree. The fuselage continued for another 100 metres where the engine still running at high rpm was buried a half-metre in the forest floor. "The accident was consistent with the operation of an aircraft in marginal visibility, close below the cloudbase where the pilot inadvertently enters IMC conditions possibly due to a slight lowering of the overcast. In this situation one option is to lower the nose attitude slightly, establishing a small rate of descent with the expectation of regaining VMC within two or three seconds. This scenario would account for the slightly nose-low attitude at the point of contact with the first tree and for the marginally greater than cruise airspeed indication at the time of impact." For another example of 'pressing on' read this fatal accident report. Apart from accepting that you will not be able to cope with adverse weather conditions encountered at low levels, and thus positively resisting that urge to press on or get home (which urge seems to become quite strong once you have passed the half-way point), the following rules can save your life. "Stay in the clear!" Watch what is developing around you — including behind you. Don't fly towards worsening weather — if you have to change course, fly towards better conditions/terrain. In conditions where the METARs indicate little spread between temperature and dewpoint — or the air just feels cooler and damp, perhaps a bit drizzly — watch out for mist, fog, fractus or scud suddenly forming. This is particularly in valleys, across ridge-line saddles or on wooded slopes, and more so in the late afternoon which, when combined with a compunction to get there before dark, can lead to disaster. Also the gaps in a layer of broken cloud — in front, behind, above or below — may start to close in at any time and perhaps very rapidly. When any doubt exists, make a 180° turn or divert towards better conditions. The accident reports cite too many instances of light aircraft 'controlled flight into terrain' or 'continued VFR flight in adverse weather' because of increasing cloud cover, a lowering cloud base or reducing visibility; probably because the pilot thought 'I'll just go a little bit further and see what the conditions look like there'. "Be wary of lowering cloud and rising ground!" If you can't see a gap between the horizon and the overlaying cloud base, be absolutely sure you can proceed and be very careful that: (a) you are not gradually climbing and losing airspeed; and (b) that you don't get into a position where poor visibility precludes making a 180° turn without entering cloud. "Be wary of valleys!" If you can't see the tops of surrounding hills because of cloud, don't fly into a valley unless: (a) you can clearly see the exit and the horizon is quite clear and well defined at that end; and (b) the valley is clearly wide enough to do a 'U' turn at any time. In addition, possible turbulent downflows over the windward slopes warrant some precautions when turning. "Be wary of concealed CB and squall lines!" When flying below low and mid-level unbroken or broken cloud layers ,cumulonimbus and squall line development may be concealed from view and you may suddenly encounter extreme turbulence, wind shear and very heavy rain with consequent loss of VMC in the worst possible conditions. Don't get caught on top! If caught above what appears to be an extensive cloud layer it is generally wise to turn 180° and climb for a better line-of-sight distance while returning to clearer sky. But remember the wind velocity changes with height, so what may be a favourable wind at low level may be unfavourable at height. "Be wary of sucker holes!" If caught out above a cloud layer be extremely wary of descending through a hole in the layer. Such holes tend to suck you in but — if the hole starts to fill or proves not to be wide enough to conduct a safe slow-speed descending turn — disorientation may spit you out the bottom. Also you have to be sure that, having descended through the hole, the height of the cloud base, terrain and visibility will allow safe onward passage in VMC under the cloud cover, or at least the option of a safe precautionary landing. Once descending in a sucker hole it may well prove impossible to climb out of it — without entering cloud — if you change your mind. There are several articles, contained in the online version of CASA's magazine Flight Safety Australia, which are recommended reading. See the section titled Micrometeorological event effects and VFR incursions into IMC in our index to those magazine articles, be sure to read "178 seconds to live". Also read 'Wind shear and turbulence' in the 'Decreasing your exposure to risk' modules. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.7.1 What is airmanship? The definition of airmanship is somewhat indistinct. With the introduction of computerised control systems, the application of airmanship is certainly more broadly based and complex now than 50 years ago. Some might say it involves pilot proficiency, flight discipline, aircraft system and airworthiness knowledge, and skill in resource management, plus being fully cognisant of every situation and exercising excellent judgement. A few years ago someone did say — in relation to the management of airline transport aircraft — airmanship is "the ability to act wisely in the conduct of flight operations under difficult conditions". If that is valid then the three-pilot flight-deck crew of Air France Flight 447, with 20 000 flight hours experience, failed their crucial airmanship test on June 1, 2009. The author's definition is reasonably applicable to sport and recreational aviation: Good airmanship is that indefinable something, perhaps just a state of mind, that separates the superior airman/airwoman from the average. It is not particularly a measure of skill or technique, nor is it just common sense (i.e. the normal understanding and judgement we should all have). Rather, it is a measure of a person's accumulated learning — their knowledge and awareness of the aircraft and its flight environment, and of their own capabilities and behavioural characteristics; combined with good judgement, wise decision-making and attention to detail in the application of that learning; plus a high sense of self-discipline. Airmanship is the cornerstone of pilot competency. Competency has been defined as the combination of knowledge, skills and attitude required to perform a task well or to operate an aircraft safely — in all foreseeable situations. For example, here is an extract from an RA-Aus incident report: "The aircraft, with instructor and student on board, was returning to the airfield when a pitch-down occurred. Not known to them the elevator control horn assembly had failed. Control stick and trim inputs failed to correct the situation, but a reduction in power did have some influence, though not enough to regain level flight. A satisfactory flight condition was achieved by the pilots pushing their bodies back as far as possible and hanging their arms rearward. A successful landing at the airfield was accomplished." A flight operation, even in the most basic low-momentum ultralight, is a complex interaction of pilot, machine, maintenance, practical physics, airspace structures, traffic, weather, planning and risk. When every flight is undertaken, it is not only the aircraft that should be airworthy; the total environment — flight planning, airframe, engine, avionics, atmospheric conditions, pilot condition and aircraft handling — should allow for the safe, successful conclusion of each operation. It is the perception — founded on the acquired underpinning knowledge — of the state of that overall flight environment and its potential threats that provides the basis for good airmanship and safe, efficient, error-free flight. Insufficient perception and insufficient self-discipline create a pilot at risk. The bulk of sport and recreational aviation is undertaken by 'amateur' pilots (using the original meaning of the term; i.e. a lover of a particular activity or pastime), but such pilots must still approach aviation with the continuing diligence of a professional. Less experienced pilots must acquire levels of airmanship consistent with their progress along the aviation learning curve. Ensuring engine and/or airframe airworthiness prior to flight is a prime component of airmanship. Owner-pilots are totally responsible for the continuing maintenance of their aircraft, be it a hang glider or a high performance aeroplane. However — for the person accepting an aircraft they do not own/operate — airworthiness, unfortunately, is a matter of faith in the operator, and in the accuracy and completeness of the aircraft's maintenance record. Daily inspections and pre-flight checks cannot assure airworthiness — the pilot does not know what is hidden under the skin or within the engine. Just as the term 'seamanship' implies a full appreciation of surface wave action and sea movement, so 'airmanship' implies a full appreciation of atmospheric waves, eddies, thermal activity and turbulence. 4.7.2 Risk management Most sport and recreational pilots accumulate only a small number of hours each year; about two-thirds of powered aircraft fly less than 60 hours. Perhaps such annual hours is enough to maintain physical flying skills learned at the ab initio flight school — if the pilot has established a program for self-maintenance of that level of proficiency — but maybe not enough to maintain a high level of cognitive skills; for example, situation awareness, judgement and action formulation. In addition, having completed flight theory studies sufficient to pass the basic aeronautical knowledge test and achieve the RAAO's Pilot Certificate, it seems that many, perhaps most, pilots leave it at that — so failing to expand their knowledge by further in-depth studies of flight dynamics and the application of the acquired knowledge; possibly because it involves sometimes difficult detail rather than the broad-brush approach of the flight school. Or, perhaps, assuming that the necessary knowledge will be acquired through subsequent flight experience, also assuming (I guess) that they will survive every learning experience in a condition to continue flying. However, many pilots are just continually repeating the same flight experience — each year is the same as the last — so all they accumulate is a repetition of one year's experience. They have no program of deliberately advancing knowledge and skills, nor have they really absorbed the safety basics that should have been drummed into them over the years — never turn back following EFATO; always maintain a safe airspeed; if the engine has been misbehaving never take off until the problem is identified and fixed; if the engine goes sick in flight don't try to make it back to base, land ASAP; don't continue into marginal conditions — turn back; and so on. So a safety problem exists with some pilots. Many are just not ensuring that they accumulate adequate post-certificate knowledge and skills. In short, they never really learn much about flight dynamics and the atmosphere (and some of their accumulated beliefs are dangerously false); they lack other pertinent knowledge; and worse, they are just not listening or hearing. Be assured that every pilot needs to know more. The sound pilot must understand how the environmental parts relate and interact with each other, and judge the likely consequences of any action, deliberate non-action or random event. A systematic approach to continuing improvement in airmanship, plus an ability for self-appraisal, is necessary to achieve that understanding. The Flight Manual or Pilot's Operating Handbook for the powered aircraft model being flown must be fully understood, and the content recollectable when needed in an emergency. Every flight should be conducted correctly and precisely, using procedures appropriate to the airspace class and without taking shortcuts, even if just a couple of circuits and landings are contemplated. To paraphrase Louis Pasteur's 1754 observation: 'Chance favours only those who have prepared'. Pilots should be aware that fatigue, anxiety, emotional state — or flying an aircraft that stretches their skill level or just flying an aircraft they don't like — will affect perception and good judgement. See the "I'M SAFE" checklist. Most studies of aircraft accidents or incidents reveal not a single cause but a series of interrelated events or actions that, being allowed to progress without appropriate intervention from someone, lead to an unplanned termination of the flight. A U.S. Navy pilot once wrote "In aviation you very rarely get your head bitten off by a tiger — you usually get nibbled to death by ducks." However, U.S. Navy pilots are well-trained, well-informed, self-disciplined individuals who do not expose themselves to those situations where eventually the tiger WILL bite your head off. Many years ago, the gliding community demonstrated that there were two main cyclic periods (for them) where people were accident prone. This was about the 100-hour mark, where pilots were beginning to think they were immortal, and about 200–250 hours when they were sure they were; being survivors of the incidents of the first period. Dr Rob Lee, the then Director of the Australian Bureau of Air Safety Investigation, wrote in 1998: "Over 40 years of investigation of General Aviation accidents by BASI and its predecessors clearly shows that while the immediate circumstances of each accident may well be unique, the underlying factors are always drawn from the same disturbingly familiar cluster — pre-flight preparation and planning, decision making, perception, judgement, fuel management and handling skills". A study of the factors contributing to fatal general aviation accidents in Australia for the ten years 1991–2000 showed that inadequate flight planning was a factor in 38% of the accidents, aircraft handling errors in 30%, and fuel starvation or exhaustion in 10%. 4.7.3 Situation awareness (The Australian Civil Aviation Safety Authority's 2009 publication 'Safety behaviours - work book for pilots' © CASA includes airmanship and situation awareness text from this page.) Being situationally aware means to be fully cognisant of the big picture at all times, by continually collecting and judging information from sources inside and outside the cockpit. In flight, a pilot has to be thinking several minutes ahead of the aircraft, not several seconds behind it — to perceive what's going on and be able to impose sound judgement on every change, from a minor distraction to a major in-flight emergency. Stress may build rapidly in an emergency situation and the pilot will tend to unconsciously focus on a very few aspects of the situation, without noticing that other aspects are degrading — airspeed or attitude for example. Good handling of any unusual situation — particularly the first major emergency — provides a basis for confidence in abilities. Poor handling of an emergency will undermine confidence. (Note: I have used the term 'situation awareness' throughout the various guides rather than the more commonly seen 'situational awareness'. This is to accord with the official documents CAAP 5.59-1(0) , CAAP 5.81-1(0) and the CASA day VFR syllabus – aeroplanes (PPL and CPL). CAAPs provide recommendations and guidance to illustrate a method, or several methods by which legislative requirements may be met. ... JB) There is much written on the ways to improve situation awareness but it boils down to a few basics: Assimilate an adequate knowledge base. To enable appropriate judgements and manage threats — or your errors — you must have sufficient underpinning knowledge of all relative aspects of flight, of human limitations and of the aircraft you are flying. Plan well in advance with a properly researched weather forecast and flight plan. Pre-flight planning may start days before a flight. Even local flying should be preceded by looking at a met forecast the evening before — to compare against the conditions you find and how the sky really looks. You must know the aircraft's take-off and landing capability in the existing or expected environment. Continually monitor flight progress against that plan, re-evaluating where necessary and implementing alternatives as soon as the need becomes apparent. Develop and use a scanning technique that takes in engine instrument indications, flight instrument indications, aircraft heading, flight path (60° left, ahead, 60° right, above, below), time, map and ground. Develop a scanning pattern that covers everything without becoming superficial but also allows time to be allocated to individual scan segments according to your perceived needs. For scanning techniques read 'Eye on the sky' in the September – October 2003 issue of Flight Safety Australia. For a research report on the limitations of the VFR unalerted 'see-and-avoid' principle read this 1991 ATSB report. For a description of the pilot's role in collision avoidance read the FAA advisory circular AC90-48C. Project ahead and rehearse your actions — for example: "The next checkpoint will be in sight in …" "If the next checkpoint doesn't appear as scheduled I will … " "If the cloud is not as high as it appears or there is more of it than there appears I will …" "If an aircraft appears on a straight-in approach I will …" "If the engine packs up soon after lift-off I will …" "If the engine packs up above 200 feet I will …" Avoid locking on to a problem, a task — or, for instance, your intended landing point — for too long, don't keep your head in the office, keep the scan going, be aware of the relative position and movement of other traffic, hold the heading and fly the aircraft at a safe airspeed appropriate to current atmospheric conditions and your height above the surface and obstructions. When operating at or in the vicinity of airfields, use a radio transceiver to communicate your position and intentions to other aircraft. Listen out for those key words that indicate other aircrafts' positions and intentions. Be aware that not all aircraft will be radio-equipped and even those that are may not be listening out on the appropriate frequency. Project ahead to plan safe and orderly traffic separation — most light aircraft mid-air collisions and near-misses occur in the vicinity of an airfield. In short — be well informed, plan well in advance, fly to that plan, continually monitor flight progress, use a scanning technique and be aware. Know where all other aircraft are and their intentions, communicate when appropriate, project ahead and, above all, don't be distracted — fly the aircraft and fly it at a safe speed and within your limits and the aircraft's performance limits. 4.7.4 Self-discipline The reason for choosing to ignore the established rules is usually to save time or money, coupled with the belief that they will get away with it because 'It can't happen to me' or 'It'll be okay'. Sometimes, particularly when they flout the laws of physics or aerodynamics, it is either pure bravado or wanton disregard (i.e. plain stupidity), or maybe it is just lack of knowledge. There are — fortunately only a few — rogue pilots in the various aviation communities who believe that the rules, written or otherwise, are stupid or unnecessary, and so determine to flout them. Such people ignore the trail of injury and death, stretching back over most of the 20th century, which formulated the rules and conventions. Each conscious infraction of those rules further dulls good judgement until crunch time finally arrives and, unfortunately, such rogues often take others with them. All pilots have a moral responsibility to inform a passenger, intending to fly with a person known to engage in illegal or doubtful activities (e.g. unauthorised low flying or inappropriate manoeuvres around the airfield), that flight with that person is inadvisable. If a person is known to consistently indulge in illegal or dangerous flight then there is a responsibility to inform an appropriate authority — police, CASA, RA-Aus, HGFA, etc. All pilots must occasionally ask themselves the question: Am I maintaining a fully disciplined approach to all flight and pre-flight procedures? And if not — why? Good airmanship cannot co-exist with poor discipline. A self-evident truth is that a pilot lacking the appropriate self-discipline is an accident in preparation. Discipline overrides panic and reinforces the ability to maintain/regain control of the aircraft when faced with a serious flight situation. 4.7.5 Rules, regulations and common sense Not even the most experienced pilot, flying maximum hours every year, can judge the probability of all likely outcomes in any situation, expected or unexpected, and make the appropriate decisions. For that reason, among others, a system of regulations, rules, conventions, practices and standard procedures exists for recreational and sport aviation — and all other aviation communities — to follow. Once acquainted with them, these rules and procedures, plus commonsense practicality, generally provide an acceptable level of protection. But far too often, pilots and others — all of whom should know better — deliberately choose not to follow them and thus abandon that inherent protection. 4.7.6 Personal operating procedures Standard operating procedures (e.g. joining the circuit, completing a flight note) are not included in the RA-Aus Operations Manual. However, every pilot should develop and follow their own set of personal operating procedures and apply them, where applicable, to each flight operation: e.g. a procedure to be followed if unsure of position on a cross-country flight; or turn-back if you find yourself flying toward rising terrain and a lowering cloud base; or having the self-discipline, when under time or other pressures, to decide whether you should take-off in the first place! If there is doubt about the weather, the wise pilot leaves the sky to the IFR-rated pilot in the IFR-rated aircraft. A non-IFR pilot caught out in instrument meteorological conditions [IMC], or dark night conditions, will be very lucky to survive. The dedicated pilot flies accurately, using approved technique, knowing the performance (i.e. the best rate) airspeeds for the aircraft being flown and consistently maintaining such airspeeds — and the chosen altitudes and headings. She or he will know the minimum safe speeds for various angles of bank when turning in level, climbing and descending flight — and at varying weights and cg positions. The pilot will know the aircraft's glide performance and, during flight, will be continually monitoring the ground for possible safe landing sites should the engine fail. Such pilots will have developed a set of tolerances for personal performance assessment; e.g. airspeed consistently within 5 knots, altitude within 100 feet or heading held within 5°. The dedicated airman or airwoman aims to fly with style, making smooth, timely and balanced transitions when turning, climbing, descending or levelling off so that the flight path flows, rather than being seen as a string of loosely connected manoeuvres. Every landing is a gentle arrival that doesn't strain any part of the aircraft. 4.7.7 Human factors training The term 'pilot error' appears extensively in safety investigation reports but is generally a most unsatisfactory summation of an event and its causal factors. In the 1980s the International Civil Aviation Organization [ICAO], the administrative authority for the world's international air transport system, finally accepted the inevitability of human failure in flight, maintenance and other aviation operations. Consequently, in the late 1980s ICAO introduced 'human factors' [HF] training and assessment requirements for pilots (and others), and circular 227-AN/136 'Training of operational personnel in human factors' was issued. Effective August 2008, RA-Aus introduced human factors training to the flight training syllabus; consequently, from August 2008, all student pilots study HF in their training and, by 31 August 2010, all existing Pilot Certificate holders must complete an RA-Aus HF course or pass the RA-Aus written HF examination, or show other evidence of meeting the required competencies of the RA-Aus Operations Manual, section 3.09. The Australian Civil Aviation Safety Authority [CASA] also decided that, from 1 July 2009, threat and error management will be added to the existing human factor aeronautical knowledge examinations within their day VFR syllabus. The Civil Aviation Advisory Publication CAAP 5.59-1(0) 'Teaching and assessing single-pilot human factors and threat and error management' was published in October 2008 and is recommended reading. CAAP 5.59-1 links human factors with deficiencies in airmanship. The CAAP defines human factors as 'Optimising safe flight operations by enhancing the relationships between people, activities and equipment. This means: achieving the safest outcome for flight operations by the most effective use of people, and what people do when operating in the aviation environment and the equipment they use.' The 2009 CASA safety behaviours publication 'Safety behaviours: Human Factors for Pilots' is available. The pack consists of: Safety behaviours – resource guide for pilots (183 pages plus a CD) Safety behaviours – work-book for pilots (111 pages) Safety behaviours – facilitator's guide (15 pages) Guidance material – extract from CAAP 5.59-1(0) (42 pages) The pack can be purchased from the CASA online store for the cost of postage (one copy per person only). Further reading The online version of CASA's magazine Flight Safety Australia contains some articles relating to airmanship, which are recommended reading. A categorised index of articles of interest to recreational pilots contained in Flight Safety Australia since 1998 is available on this site. The articles are listed within ten categories together with a very short summary of the content. 4.7.8 A CFI's viewpoint The late Tony Hayes, once CFI of Brisbane Valley Leisure Aviation Centre — and the inaugural holder of the RA-Aus Meritorious Service Award — published this airmanship interpretation. "Airmanship — aviation could not exist in a responsible manner without this apparently intangible component. Let us define airmanship exactly so you do know what you are searching for to make your own, and thus achieve personal protection, pride, and protection of others, in your own standards of what you do, or propose to do. The big intangible is our personal attitude to flying — why we do it, how we do it. Do you care to define an individual's personal attitude to both flying and the environment in which that person's flying is conducted? Many things form our attitudes and we need to consider these if we wish to see airmanship as it really is — get a handle on it and make it our own. That is easy enough, but before we start — accept that airmanship is something that grows. It grows on experience whether shaped by training or by personal exposure to what you do. You cannot learn airmanship only from a book or an instructor, you are as much guided there by exposure to those circumstances, encountered with growing experience, which require airmanship. Whether it be flying training or airworthiness training — only the basics can be established. Like the runner in a relay race taking the baton — you have the potential winning element in your hand, it is up to you if you win or not, take on what you have been given, and make it work for both yourself and the others with whom you share the skies. Winning the airmanship race is not simply about staying alive or not bending yourself or aircraft — it is walking off the airfield relaxed, knowing you have not simply performed but have crafted an activity, and being totally aware you have enjoyed the sum of that and owe nothing to anyone. Let us start with a target to shoot for. Airmanship — a definition 'A personal and situational management state required to allow a human being to enter and exit, in safety, an environment which they were not naturally designed to inhabit. This state comes into being immediately a decision is made that an aircraft is going to be flown and continues until you walk away from the completed flight and correctly secured aircraft.' That continuation may require an instinctive willingness to assess, between flights, the lessons that have been stated by the flight just completed. Airmanship is as much a ground-based attitude as it is an air-based one. Airmanship structure We are now going to look at the basics upon which airmanship is formed and therefore can be understood. We have already touched upon one — PERSONAL ATTITUDE — now we must put this in context with the others: KNOWLEDGE — SKILL — CONFIDENCE — RESPONSIBILITY. These four are then applied by personal attitude. The application of airmanship can be defined to three areas: the airworthiness of the aircraft the operation of the aircraft and the environment in which the aircraft operates. We will briefly examine each of these requirements and applications. All four requirements are intimately interconnected with each other and with applications, so cannot be treated entirely as stand-alone subjects. Knowledge • AIRWORTHINESS. You do not have to be a mechanical engineer to be a pilot but you do need to know sufficient about the aircraft structure and systems to enable you to safely pre-flight it and adequately monitor its continued satisfactory operation. The degree of knowledge required will depend upon the complexity of the machine and the range of environments in which the machine is capable of operation. (See the 'home builder' comment below.) As pilots do not have to be engineers, there is therefore a supporting mechanical and engineering system to which the pilot will generally interface, via documentation, which revolve around periodic servicing and in-service defect reports. Understanding this system is part of the knowledge requirement such that you do appreciate whether the aircraft is provisionally serviceable or not — subject to pilot inspection. • OPERATIONS. These are very much the pilot's responsibility and sufficient knowledge must be present for the safe operation of the aircraft within the parameters for which it has been designed. This knowledge must extend adequately from flight principles through to understanding of systems operation. All of this must then interface with the environment within which the aircraft will operate and this in turn requires understanding and application of airspeed limitations, manoeuvres permitted, weather minima (e.g. maximum crosswind limits), etc. • ENVIRONMENT — Meteorology. The forces exerted by the ever-changing atmosphere upon an aircraft are far removed from those weather considerations we have knowledge of when we exist only on the ground. The pilot has to be able to read the sky like an advertisement, interpret current conditions and identify changing conditions along with the rate and degree of change. Decisions so made then have to be balanced with aircraft operational limits and the pilot's personal skill limits — usually this is a forecast being responded to before the situation has moved beyond estimated limitations. — Behaviour controls. In simple terms this is knowing the 'rules of the road' in terms of rules of the air. From simple basics such as 'give way' rules, to airfield marking systems, to airspace restrictions — these are all designed to enable the present huge variety of aircraft to share airspace safely. They must be understood and instinctively applied by the pilot. — Regulation. Partly from lessons learnt the hard way in the past, and partly due to an ever expanding population both in the air and on the ground — the information resource of who does what to whom is bound into regulation. The pilot needs to know this regulation as applicable to his or her operation, respect that others have different parameters they must follow and make allowance accordingly, plus have the regulation available and currently updated to suit the operations being conducted. Skill This is an area determined, at least on the surface, by our ability to perform certain actions and procedures. But you can teach a bird to talk — that does not mean the bird understands what it is doing or can hold a conversation. Skill is underpinned entirely by knowledge and from this skill may be put in context and is capable of organised development based upon growing experience. • AIRWORTHINESS. The degree of skill in this area depends upon the level of airworthiness control you intend to apply. In pilot pre-flight terms, the skill will be certainly underpinned by a healthy element of curiosity — does it actually work and is it likely to stay in place! As we move further into servicing and repair, then hand and machine skills (adequately supported by appropriate knowledge) increase. For both control and convenience, divisions are made as to the degree of work which may be undertaken via various airworthiness maintenance approvals, each requiring higher knowledge and skill levels. • OPERATIONS. As the aircraft you have access to become more complex then so the further you are removed from basic stick and rudder skills to new skills that are mainly founded upon systems operation and changing operating parameters. Those basic skills have to be totally and automatically in place, with sufficient competence of application supported by knowledge, such that the new skills may be safely founded. With this foundation, you may move from a simple aircraft to a slightly more complex one with some confidence and further acquisition of systems and operating parameters — but you should instinctively stop if you are clearly going beyond your existing knowledge and skill base until you have corrected that situation. There is another element to skill and that is currency. None of us, no matter how much we have flown, are any better than our next arrival on the ground. If we are not current (particularly with more complex aircraft, which require confident fluidity in the checks and procedures with their operation) then we could just be rolling the dice on the basis of 'been there, done that — she'll be right'. But even the simplest of aircraft will severely bite the 'out of practice' pilot. How much out of practice is 'out of practice'? The airman instinctively knows. Situational appraisal, how long out of practice, so many other things — all come into play here. As a command pilot, the airman will make a valid decision based on information and assessment, and react accordingly and safely. • ENVIRONMENT. In this situation we are less concerned about the tirades of the weather (although that has an obvious control upon how skill is intended to be employed). In airmanship terms we are more interested in the human environment of peer group pressure, personal needs to achieve a task, or (for some pilots) pressure applied by employers. Too often, a flight becomes driven by emotive pressure and/or need to complete a flight for personal gain (in so many forms). Emotion and personal gain are the two biggest killers yet invented by our race. Every year the figures continue going on the board in terms of deaths and wrecked aircraft — ran out of fuel, weather out of parameters, flew into lowering cloud base and rising ground. It still happens every year! As human beings we are never more vulnerable than when our skill is being questioned or challenged by others — or even ourselves, particularly in situations where by its very nature flying begins being interpreted as some 'personal courage combined with ability' thing. The true airman, with knowledge present and supporting skill in place, is dispassionate and evaluates situations on known and observed circumstances. Too often for some, tomorrow may indeed have been soon enough, but was not! Confidence Confidence can be underpinned by one simple control statement — 'If in doubt, don't'. If there is doubt, then confidence by definition does not exist. If you are not confident then you should not go. Confidence is formed by adequate levels of knowledge and skill. The airman has these in constant balance and sees a flagging of confidence as a natural warning bell — there is yet more work or revision to be done so that confidence is truly there. When those warning bells sound then it does not matter if the doubt concerns whether the aircraft is serviceable, or if you are up to the flying you are undertaking — time to take pause and look for additional abilities. There is also another element to confidence, and that is overconfidence. In this situation, even adequate knowledge and skill is being superseded by an emotive form of confidence. Once with a Pilot's Certificate achieved, the need to satisfy an instructor's discipline may fade, knowledge becomes steadily forgotten as a stimulus to what must be, and skill currency may go the same way. With the demand strictures of flying training now past, near enough may be good enough — forgotten is the need for why those original standards were set. Overconfidence meets its true ground in exhibitionist flying. In this situation the pilot is driven by ego, deliberately in front of an audience (which is mandatory) to show they are more than mortal and can really 'fly'. Unfortunately, the accident records confirm that such people are indeed mortal. Those tend to be the 'headlines' examples — but the run-of-the-mill situations are the greater number of people who bend themselves and/or aircraft — or — the much larger majority who narrowly avoid disaster, and hopefully become airmen as a result of that new demonstration of their inadequacy. Responsibility If confidence cannot exist without knowledge and skill then the exercise of responsibility cannot exist without all three. Here the airmanship pattern may be disrupted and two opposites meet. A totally trained, knowledgeable and skilled pilot, under the influence of irresponsible behaviour, can be as discounted as the worst non trained aerial lout. Ultimately we are human. We are subject to human drives. So maybe there is another definition to airmanship — the self-discipline and wisdom to rise above our human condition and just be practical about what we do and where we do it. Homebuilders Within the ultralight community we have a sector of effort which is, via particularly CAO 95.10, but within overtones of 'amateur built' — an area where airmanship principles themselves may be seen by reflection. In this area, the intending pilot does have to embrace sufficient elements of the designer, engineer and aircraft constructor. The requirements for knowledge and skill are self-evident. Confidence will ultimately be expressed by a preparedness to fly the finished machine. Responsibility will be expressed by understanding that sufficient knowledge and skill was present to build the machine to an airworthy standard, but there is also equal knowledge and skill present in the operations area to ensure that the proving flights are conducted safely, responsibly and with validity. Near enough is never good enough on a new aircraft type. So the ultimate definition of airmanship, when seen in context with allied disciplines, comes down to quality of performance within prevailing circumstances — backed by quality of personal intent. Flying is fun — a pile of wreckage is neither. Between those two extremes is the ultimate expression of airmanship." The following document is an extract from the BVLAC flying training manual written by the late Tony Hayes. (The flying school has since ceased operations.) "For all my exposure to aviation — which extends over my entire life from my birth next to an operational bomber airfield in World War 2 — when I came to pilot training myself I met a term so commonly used yet nowhere could I find actually defined and explained, Airmanship. So I will fix that right now in my own flying training manual. The problem is understood once Airmanship itself is actually understood. It is very real and manifestations of it may be seen at every airfield or places people come to fly aircraft. Yet Airmanship is an intangible, for it is a state of mind, personal convictions and self discipline expressed in our actions and attitudes. It is the prudent operation of a machine, and the management of circumstances surrounding that operation, within an element we were not naturally designed to inhabit. Airmanship appears in every flying area and sets aside the airman from the aircraft driver. It is founded firmly in basic training where mental attitudes to flying are forged, and sometimes in self training where a pilot learns the hard way about what is prudent or not, gets away with it, and elects to make more sensible decisions at the right time, next time. Under growing experience airmanship may grow and blossom into a comfortable protective cloak, resting light upon the shoulders, worn perhaps with pride, but never in vanity, and giving the protection of 2 inch armour plate. The very need for its presence is a reminder that we are privileged to transit from our natural element into another. There may be a high price for such transition if that act is made in scorn or ignorance. But we may go there safely if we acknowledge the limitations of ourselves and our machines, so generating a curious mixture of humility and confidence which is expressed in the very form of airmanship. Airmanship may be performing a proper pre-flight check of an aircraft rather than a casual look-around. It is something as instinctively looking before turning. It is actually doing pre take-off and pre-landing checks — not mouthing the words. It is sensible pre-flight planning — either for a circuit or going over the horizon. It may be as simple as looking at the windsock before hitting the 'loud' lever, or as complex as interpreting a changing weather pattern. It is the essential personal and situational management difference between being up there wishing you were down here, rather than being down here wishing you were up there. But, founded on flawed training, or growing experience driven by a different pride, airmanship may wither into a deadly weed of contempt for those who slavishly obey 'regulation' or are not deemed 'good enough' to sort out situations as they happen. People driven by such views, in their ignorance, inhabit a perilous place of their own making wherein they have become an accident looking for somewhere to happen, and so ensure that it will happen. The non-airman will discount that the 'officious regulation' is (in the main) a book written in the blood of people who found out the hard way and handed down to us methods of avoiding their fate. In discarding that knowledge so is generated the certainty of the same fate, standing in the shadows, waiting. The airman is a person who maintains a valid skill and knowledge currency such that when the unexpected does happen there is ability and composure enough to manage the situation into safety. He or she, is a person with a sense of balance and intelligence enough to heed the lessons of the past, apply them in the present, and so ensure a future to be able to fly again, and again, and again. You will be hearing a great deal more about airmanship in your time with us, and now you know what we are actually talking about." — Tony Hayes, CFI; Brisbane Valley Leisure Aviation Centre STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.6.1 Being prepared for an emergency There is always a possibility of an en route engine problem, or other event, necessitating an off-airfield landing. A reasonable knowledge is required of the procedures with such incidents, particularly those occurring in remote areas and you should have an emergency check list readily available in flight. Read the 'Coping with emergencies' guide. Before departure it is certainly wise — if not mandatory — to leave enough information with a responsible person, so if you fail to check in with them by an agreed time a telephone ring-around can be initiated. If that is unsuccessful the Rescue Coordination Centre of the Australian Search and Rescue organisation [AusSAR] can be notified. AusSAR will attempt to make contact with the missing aircraft. If that is also unsuccessful AusSAR will initiate a search that uses your planned track as a starting point. The information that AusSAR requires is contained in the flight note form recommended by the Australian Maritime Safety Authority [AMSA]. AusSAR is a division of AMSA. For further information on communications when in difficulties, the legal requirement to carry a 406.025 MHz ELT, AusSAR ELT monitoring and search procedures; refer to the 'Safety and emergency communication procedures' in the VHF radiocommunications guide. 4.6.2 Planning and equipment check list There are several civil aviation regulations (a copy of which can be viewed at Some noteworthy sections of the Civil Aviation Act 1988, the CAR 1988 and the CASR 1998) that direct pilot actions before flight. These regulations are CAR 78 'Navigation logs', CAR 92 'Use of aerodromes', CAR 233 'Responsibility of pilot in command before flight', CAR 234 'Fuel requirements', CAR 235 'Take-off and landing of aircraft etc', CAR 235A 'Minimum runway width', CAR 239 'Planning of flight by pilot in command', CAR 244 'Safety precautions before take-off' and CAR 282 'Offences in relation to licences, certificates and authorities'. The requirement for two-place aeroplanes to carry an approved emergency locator transmitter is stated in CAR 252A; single-seat aircraft are exempt from that requirement but it's certainly wise for all aeroplanes to carry an ELT. In addition CAR 232 'Flight check system' requires provision of procedural check lists and the pilot must ensure that the check lists are carried in the aircraft. Note that CASA EX38/2004 exempts owners/operators of aircraft with a MTOW under 5700 kg from compliance with the requirement to obtain prior CASA approval of those flight check systems. It is advisable to run through a flight planning, navigation equipment and aircraft equipment check to ensure that all requirements have been covered. So, have you: established the safest route and minimum safe altitude; avoiding rough or heavily forested terrain, designated remote areas, other hazardous areas, restricted areas and CTA/CTR — or airspace where a functioning Mode C/S transponder is mandatory — and marked that route on the paper or digital chart you will use in flight? double checked that all magnetic bearings and distances on the flight plan have been measured and recorded correctly? checked the locations and the suitability of refuelling stops, of the destination airfield and of alternate landing areas? checked their details in ERSA including ASIC requirements and the landing charges applicable? For airfields not listed in ERSA have you checked with the owner/operator about the airfield dimensions, slope, condition, approach and departure terrain limitations, animals, tree heights, power lines and other obstructions/hazards? Is your skill level sufficient to safely land and take-off at those airfields? checked the en route, destination and alternate airfields' weather, cloud base and visibility (ARFOR, TAFs, METARS and surface chart), end of daylight (bearing in mind the effect of forecast cloud conditions on visibility) and NOTAM (ADs, ALAs plus restricted area and military low jet route status)? checked the BoM weather radars and other internet lightning tracker sites? left a flight note — showing the itinerary and the information required by the Rescue Coordination Centre — with a responsible person who knows what action to take if you fail to check in by the agreed time? checked that watch, compass, ASI and altimeter function okay and that nothing is placed in such proximity that compass operation will be affected? checked that the VHF transceiver is functional and noted/loaded all the required frequencies? checked that the 406 MHz ELT battery is functional, not past the replacement date and that the AMSA registration sticker is current and attached to the device? Shown your passenger how to operate the ELT/EPIRB and firmly attached the device to your body? provided back-up batteries for handheld equipment? life jackets donned, if any part of the flight is over water? decided whether to take an inflatable life raft with emergency survival kit? provided a first aid kit in the aircraft and a helmet for your passenger? demonstrated the intercom system to your passenger? ensured that your passenger is: (a) familiar with the safe operation of the seat, seat-belt, safety harness and the cockpit door and (b) knows to keep their feet off the rudder pedals and not to grab the controls if startled? if intending to carry a child as the passenger, provided an adequate child restraint system plus an appropriate protective helmet? Have you considered whether any child would be legally regarded as a risk-informed participant? selected clothing suitable for the surface conditions, in case of an unplanned landing? packed an extra supply of prescribed medication? provided an adequate fresh water supply in your survival pack? provided additional survival equipment, if operating into a designated remote area shown in ERSA GEN-FIS 'Designated remote areas' or on VNCs? loaded tie-down gear securely? checked that the Aircraft Flight Manual/Pilot's Operating Handbook, cockpit check lists and flight plan, plus all the associated Airservices Australia documents and publications, are current and on board? If the aeroplane is an LSA check that a copy of the CoA and Statement of Conformity is in the aircraft flight manual. (Maps, check lists, AFM/POH and flight plan could be digitally stored in an appropriate and approved electronic flight bag, but see 'Electronic flight planning & the EFB'; the check lists might be stored in an EFIS) physically checked the fuel in the tanks and oil in the engine for suitability, quality and water or other contamination? Stowed the fine fuel filter funnel for en route use? physically confirmed that the actual fuel load provides a reasonable margin — on top of the flight plan needs including the reserve? measured and ensured that aircraft take-off weight and centre of gravity — with everything and everyone loaded — will be within the limits stated in the Aircraft Flight Manual/Pilot's Operating Handbook and retained evidence of the W&B calculation? calculated the density altitude — and the take-off distance required from the Aircraft Flight Manual/Pilot's Operating Handbook to ensure that take-off distance, to clear a 50 feet screen, is amply provided by the dimensions and current condition of the intended runway at the departure airfield? done the same density altitude calculations for the destination and alternate airfields, and ascertained that the effect on landing, take-off, and climb-out performance, at the expected aircraft weights and cg positions, will still provide an adequate safety margin taking into account the forecast weather plus the dimensions and condition of each of those airfields, and including the possibility of a late go-around? 4.6.3 Personal fitness for flight The next check is a personal one, determining if YOU are safe to fly. A mnemonic checklist has been developed for that purpose. Answering 'yes' to any of these questions may mean that your alertness, perception, judgement, general performance or situation awareness capabilities are seriously downgraded; also you are likely to forget to do something that must be done. The I'M SAAFE checklist is: Illness? Do I have an illness or any symptoms of an illness or disorder or a known condition that could present an in-flight hazard? Medication and other drugs? Have I been taking/mixing prescription, over-the-counter or 'recreational' drugs? Stress and distraction? Am I under psychological pressure from the job or personal circumstances? Am I worried about financial matters, health problems, family problems, emotional problems or relationship discord? Am I worried about undertaking this flight? Age? If you are in your later years, have you considered whether your ability to cope quickly and wisely with an emergency or an unfamiliar situation may have reached the point where it would be prudent to be accompanied by another qualified pilot? Alcohol? Have I been consuming alcohol within the previous 8 hours? (Even within 8–16 hours after consumption, blood alcohol levels can be significant. If tested by an authorised person, the permissible blood alcohol reading is less than 0.02, which is to allow for any anomaly caused by — for example — the alcohol (or similar) content of some medical preparations. Would my average alcohol consumption be classified at greater than 'very low risk'? Fatigue? Am I tired, inadequately rested or suffering from lack of sleep? Eating and drinking? Am I inadequately nourished and/or dehydrated? There are several articles, contained in the online version of CASA's magazine Flight Safety Australia, which are recommended reading. See the section titled 'Aviation medicine' in our index to those magazine articles. 4.6.4 Staying within the rules Carriage of passengers Prior to flying an LSA-certificated aircraft, the pilot must inform the passenger that the aircraft does not meet the same airworthiness requirements as an aircraft with a Standard Certificate of Airworthiness. Note: sport and recreational aviators, and the single passenger allowed, are defined by CASA (only in the aviation regulatory sense) as 'informed participants' in the activity being pursued. An informed participant is aware of the risks involved in a particular form of sport and recreational aviation and is willing to accept those risks. How do you make your passenger aware of the potential risks inherent in sport and recreational aviation so he/she can make a risk-informed decision about their participation? Various warning placards must be displayed in the aircraft cockpit but that's hardly sufficient. What if the passenger is legally a child, how can any child be considered a 'risk-informed' participant? Legally, pilots have a duty of care toward their passenger and may not take needless risks or act in an irrational manner. If you intend carrying a passenger have you performed at least three take-offs and landings within the last 90 days? See the RA-Aus Operations Manual section 2.07 para. 11. Passenger care: please read the RA-Aus Operations Manager's advice on passenger care, appearing in the July 2013 issue of the RA-Aus journal 'Sport Pilot'. Aircraft fitness for flight The Technical Manual section 4.2.1 requires that, before departure, the pilot-in-command must ensure that the aircraft is correctly maintained (by reference to the aircraft log book), the daily inspection has been completed, the RA-Aus registration card is in place and current and the aircraft's fitness for flight has been confirmed by means of a personal pre-flight inspection. Carriage of flight documentation CAR 233 (1) (h) states that 'Pilots are required to carry, and have readily accessible in the aircraft, the latest editions of the aeronautical maps, charts and other aeronautical information and instructions, published in AIP, or by an organisation approved by CASA, that are applicable to the route to be flown, and any alternative route that may be flown, on that flight'. Currently (December 2013) that means the paper maps and charts from Airservices Australia, not digital maps stored in an iPad or other tablet computer except if the EFB software is from a CASA approved supplier. The 'other aeronautical information' for VFR operations outside controlled airspace would include the current ERSA and relevant ARFORs, TAFs, METAR, NOTAM etc from the NAIPS Internet Service. Note: these requirements are in addition to the regulations mentioned above. CAR 78 states 'The pilot in command of an aircraft shall keep a log of such navigational data as is required to enable him or her to determine the geographical position of the aircraft at any time while the aircraft is in flight' and that 'The log shall be kept in chronological order'. The content of the log is not specified for aircraft operating within Australia however a fuel log facility should also be included. Weight-shift aircraft operating under CAO 95.32 are exempt from the need to maintain a navigational log. CAR 139 nominates other documents (aircraft maintenance release, aircraft flight manual, pilot licence, medical certificate) to be carried in flight, however sport and recreational aircraft operating under CAOs 95.10, 95.32 or 95.55 are exempt from complying with CAR 139. The RA-Aus Operations Manual section 2.01 requires pilots to carry their Pilot Certificate and produce it on demand from an RA-Aus official, a CASA official or a police officer; but there is no requirement (for non-LSA aircraft) in the RA-Aus Technical or Operations Manuals that mandates carriage of other documents except the aircraft registration renewal card. LSA aircraft are also required to carry at least a copy of their Special CoA, Statement of Compliance, weight and balance information under the requirements of the Technical Manual section 7.5.3(7). There is no requirement in the regulations or the RA-Aus Operations Manual for pilots to carry their personal logbook in flight, however, you may find it useful to carry a copy of the logbook notation of the successful completion of a still current flight review and, if carrying a passenger, something that confirms the recent experience requirements. The Technical and Operations Manuals require that all pertinent cockpit placards, warnings and other labels, be in place and visible to pilot and passenger. Expect a 'ramp check' inspection Generally RAAO members won't come into contact with CASA officers. However, officers from the Self-administering Sport Aviation Organisations Section do carry out 'ramp check' inspections on pilot and aircraft after landing or before take-off, at any airfield where sport and recreational aircraft are operating. Such operational checks are consistent with the Section's safety surveillance role and are the only means by which the CASA officers can sample the professionalism of the RA-Aus membership and, perhaps, the professionalism of the flight school where the more recently qualified pilots were trained. There is no need for pilots to be fearful or antagonistic — regard the check as an opportunity for a useful learning experience. Those Flying Operations Inspectors might be interested in how the flight has been planned, whether appropriate information available from the NAIPS Internet Service (and from other authoritative sources) has been used and what the navigation/fuel log reveals about how closely the flight conformed with the plan. Other checks might be appropriateness of Pilot Certificate endorsements to the journey, the aircraft type and the carriage of a passenger; evidence on currency of the biennial aeroplane flight review; currency of aircraft registration; cockpit labels/warnings/placards in place plus safety harness conditions and appropriateness. The check might include any of the items listed in the preceding planning and equipment check list but perhaps items 7, 10, 23 and 26 stand out. The Self-administering Sport Aviation Organisations Section produced a ramp check booklet in December 2013 that can be supplied in printed form. CAR 232 'Flight check system' is listed under 'Document references' but as it stands the booklet reference to CAR 232 is a little misleading for aircraft weighing less than 5700 kg, so please read an annotated version of CAR 232 on this website. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.5.1 Weather and NOTAM check Area forecast We are planning to depart Oxford for Tottenham around 1400 hrs AEST time on April 30 (0400 UTC) which allows nearly four hours flight time before last light at Tottenham. Shortly before departure time we check the latest area 22 ARFOR briefing material downloaded from Airservices Australia's NAIPS Internet Service. AREA 22 AREA FORECAST AMEND OVERVIEW: FINE APART FROM ISOLATED SHOWERS IN FAR NORTHEAST TILL 11Z. WIND: 2000 5000 7000 10000 14000 18500 070/10 VRB/10 VRB/15 240/15 ZERO 220/15 MS06 210/20 MS15 CLOUD: SCT CU/SC 4500/9000,LOCALLY BKN IN NE TILL 11Z. AMEND WEATHER: SH IN NE. VISIBILITY: 6KM SH. FREEZING LEVEL: 10000 IN SE / 12000 IN NW. ICING: NIL. TURBULENCE: MOD IN CU. AREA QNH 03/07 NW OF YMIA/YMOR 1025, REST 1027 The first part is the general weather forecast for the area as a whole — this states the weather is expected to be fine during the forecast period with some showers in the north-east, which doesn't affect our flight plan region. The wind velocity at 2000 feet amsl will be 070/10 knots and at 5000 feet the speed is shown as 10 knots but the direction is 'variable'. Scattered cumulus and stratocumulus is expected to form with bases no lower than 4500 feet and tops no higher than 9000 feet. The freezing level will be at 10 000 feet so surface temperatures will be mild. The area QNH we will use is 1027 hPa. It looks like a perfect day for flying. Note that it is not mandatory for VFR aircraft to use the area QNH while en route. You may substitute the current local QNH of any aerodrome within 100 nm of the aircraft or, if the local QNH at the departure airfield is not known, you can just set the sub-scale so the altimeter reads the known airfield elevation. Aerodrome weather reports and forecasts If we now look at some actual weather reports [METARs] and the forecasts [TAFs] for airfields in or near our flight region, we can check whether there is any significant variation from the general area forecast. The METAR for our alternate airfield Condobolin, issued at 0300 UTC, reports surface wind velocity 130/7 knots. The TAF, issued two hours earlier at 0107 UTC, forecasts that during the period 0200–1400 UTC the surface wind will be 050/8 knots, the visibility will exceed 10 km (9999 m) and there may be scattered cloud with bases at 4500 feet. There is no weather report for our other alternate. The TAFs are for an area within 5 nm radius of the aerodrome. CONDOBOLIN (YCDO) METAR METAR YCDO 300300Z 13007KT //// 22/04 Q1024 RMK RF00.0/000.0 TAF TAF YCDO 300107Z 0214 05008KT 9999 SCT045 T 19 23 19 15 Q 1027 1025 1025 1026 Be aware that smoke from bushfires or autumn fuel reduction burns can drift over extensive areas and may totally conceal some, or all, landmark(s). Smoke may also dictate flight at a considerably greater altitude than planned and visibility degradation may not appear in the weather forecast. In summer it is advisable to check the state rural fire service website maps for the current fire status. Combined with the area wind forecast it will enable an estimate of the smoke drift. Similar precautions are applicable in the northern dry season. The METAR for Ivanhoe (which doesn't appear on the WAC section shown but is just a few miles west of Oxford) issued at 0300 UTC records a surface wind velocity 120/10 knots. The TAF issued earlier at 0109 UTC, forecasts that during the period 0200–1400 UTC the surface wind will be 080/8 knots, the ceiling and visibility will exceed VMC minima [CAVOK], and from 0800 UTC the wind will shift to 120/10 knots. IVANHOE (YIVO) METAR METAR YIVO 300300Z 12010KT //// 25/10 Q1022 RMK RF00.0/000.0 TAF TAF YIVO 300109Z 0214 08008KT CAVOK FM08 12010KT CAVOK T 24 26 22 19 Q 1024 1023 1023 1024 The METARs and TAFs confirm that, although there is some variation between observed winds and forecast winds. the area forecast is generally representative of the weather in the region we intend to operate. We should also check the 'big picture' — the latest mean sea level analysis or 'surface chart' — issued by the Bureau of Meteorology but which we can obtain from Airservices Australia's NAIPS Internet Service. That chart (below) shows a persistent and strong high pressure system located over south-eastern Australia, which is directing easterly airflows into area 22 and is responsible for the fine weather. The nearest frontal weather is affecting only the south-west corner of the continent. Surface chart I have added the red wind arrows to indicate the surface airflow around the high pressure system. It is the convention that each full barb on wind arrows represents an incremental 10 knots wind speed and a half barb represents 5 knots; thus the wind speeds shown are 25, 15 and 10 knots. (Note that a solid triangular barb on wind charts indicates 50 knots.) Area 22 is located where the 10 knot wind arrow is pointing. Please read the Aviation Meteorology Guide module dealing with southern hemisphere winds. The BoM's aviation weather services provide the latest synoptic surface analysis charts and national forecast charts; area forecasts, TAFs, aviation warnings and a great deal of other information. Wind and temperature forecast charts are available for various flight levels and various times up to 30 hours ahead. To find the wind and temperature charts expand 'aviation charts' and select 'wind and temperature'. The flight levels of interest to recreational pilots are FL050 [5000 feet] and FL100 [10000 feet], note the times are UTC. Using the Aerodrome Weather Information Service [AWIS] While airborne, a radio-equipped aircraft can usually obtain a report of actual weather conditions at the larger aerodromes — see 'Acquiring weather and other information in-flight'. If a mobile 'phone is carried the AWIS (if available) can be used to obtain surface wind and some other weather data. Choosing the cruising level The forecast vertical wind profile indicates there is no cruising altitude which is better suited for an easterly flight than any other. In section 3.5 we determined the lowest safe altitudes were 2000 feet on the first leg and 2500 feet on the others. Thus there is no reason not to plan our flight at the recommended VFR cruising level for an easterly heading (below) of 3500 feet; being below the cloud base it will avoid dodging around scattered clouds. We have to make an estimate of the wind at 3500 feet, which in these light wind conditions will be above the friction layer and, as wind tends to back with height (in the southern hemisphere), we will plan for a wind velocity at 3500 feet of 060/10 knots. Flights operating under the Visual Flight Rules [VFR] outside controlled air space must be operated at levels selected in accordance with the table below when at a height above 5000 feet AMSL, and should be operated at such levels when below 5000 feet whenever practicable. (The cruising levels for aircraft operating under Instrument Flight Rules (IFR) are 500 feet lower.) VFR cruising levels Magnetic tracks 000° to 179° 180° to 359° Cruising altitudes (area QNH) 1500 ft 2500 ft 3500 ft 4500 ft 5500 ft 6500 ft 7500 ft 8500 ft 9500 ft Operating in accordance with the cruising levels does improve safety but pilots should be aware that the risk of collision still exists. For example, consider an aircraft tracking 175°, while to the south another aircraft is tracking 005° at the same correct cruising level. Those two aircraft could well be on a collision course — you must maintain situation awareness throughout all stages of flight. Weather radar The Australian Bureau of Meteorology provides online 'real time' snapshots from about 50 weather radars around Australia. The snapshots are taken at about ten-minute intervals and cover a radius up to 256 km from the antenna. The last four snapshots are retained on the website and can be looped as a progressive image, thus providing an excellent presentation of precipitation and storm movement. Go to the aviation weather services page and select 'Weather Watch Radar Network'. While on the aviation weather services page also have a look at the latest 'Satellite imagery' of the cloud cover over Australia. NOTAM check A check of the NOTAM contained in the area 22 ARFOR briefing material reveals only three that are connected with our planned flight. These three state that the aerodromes are no longer licensed by the Civil Aviation Safety Authority, which is — in effect — a notification that advisory NOTAM aren't issued for those airfields. CONDOBOLIN (YCDO) AD From: 01 090316 To: PERM C0001/02 AD LICENSE WITHDRAWN LAKE CARGELLIGO (YLCG) AD From: 01 090319 To: PERM C0003/02 AD LICENSE WITHDRAWN TOTTENHAM (YTOT) AD From: 01 090317 To: PERM C0001/02 AD LICENSE WITHDRAWN 4.5.2 The set-heading point It is necessary to establish the geographic position, and the point in time, at which navigation will commence after take-off. This set-heading or set-course point should be pre-planned if possible and is usually dependent on the take-off direction, the initial heading and the local environment. If, for example, the take-off direction coincides roughly with the initial heading, and there is no environmental reason to make a turn after take-off, then the set-course point is the take-off point — or very shortly thereafter — and an en route climb technique will be used. However, if the take-off direction is opposite to the initial heading then it is usual to climb out on runway heading until well clear of the circuit area, and at circuit height, then continue climbing in a gentle turn — in the direction of the circuit — that will bring you back over the centre of the airfield, at a height not less than 2000 feet agl, to avoid any aircraft that may be overflying at 1500 feet to join the circuit. Refer to 'The standard circuit pattern'. The set-course point is then overhead the airfield and an en route climb may be indicated if the planned cruising altitude has not yet been reached. Another possibility is to choose an easily identifiable landmark well outside the circuit area as the set-course point. Whichever position is chosen, overhead the airfield or an external location, the flight plan should start from that point and the estimated time required to reach that point should be added to the take-off time allowance in the flight plan. 4.5.3 Heading, ground speed and fuel calculation We plan to cruise at 70 knots CAS and using the rule of thumb that TAS is 1.5% greater than CAS for each 1000 feet of altitude amsl we will increase CAS by 6% to arrive at a TAS of 75 knots at the planned cruising alttude of 3500 feet. That calculation (or perhaps 2% per 1000 feet) is near enough for navigation purposes but it is not actually correct; see the section on density altitude. To complete our flight plan we have to estimate the heading to fly, the ground speed, the flight time for each route segment and the fuel required. We will use the 1-in-60 arithmetic method outlined in section 4.2. Step 1: find the wind correction angle by estimating the angle at which the forecast wind meets the tracks, multiply the result by the wind speed and divide by the aircraft TAS. i.e. WCA = relative angle x wind speed / TAS (a) Segment 1: track = 094° true; w/v = 060/10 knots; relative angle = 34; TAS = 75 knots so WCA = 34 x 10 / 75 = 5°. (b) Segment 2: track = 061° true; w/v = 060/10 knots; relative angle = 1; TAS = 75 knots so WCA = 1 x 10 / 75 = 0°. (c) Segment 3: track = 040° true; w/v = 060/10 knots; relative angle = 20; TAS = 75 knots so WCA = 20 x 10 / 75 = 3°. Step 2: calculate the true heading, remembering that the wind correction is applied in the direction the wind is coming from; so that: (a) Segment 1: wind is from the left, correction angle is 5° and the heading is 094 minus 5 = 089° true. (b) Segment 2: wind is from dead ahead, correction angle is 0° and the heading is 061 plus/minus 0 = 061° true. (c) Segment 3: wind is from the right, correction angle is 3° and the heading is 040 plus 3 = 043° true. Step 3: convert the true headings to magnetic headings. There is an isogonal passing through the chart in the route planning module (the dashed purple line), which indicates the local variation is 10½°E. Applying that to our true bearing — remembering our aide-memoire "variation east, magnetic least" — and we have the following headings: 079°, 051° and 033° magnetic. Flight plan Route segment Distance Track (true) Heading (true) Heading (mag) Oxford – Warraway Mountain 74 094° 089° 079° Warraway – road junction 52 061° 061° 051° Road junction – Tottenham 33 040° 043° 033° Step 4: estimate the ground speed. Deduct the (acute) angle at which the wind meets the track from 115 (for angles up to 60°, use 105 for greater angles) and apply that as a percentage of the wind speed (to the maximum of 100%) subtract the result from TAS if wind coming from ahead to abeam, otherwise add; thus: (a) Segment 1: track = 094° w/v = 060/10 kn: angle = 34; 115 –34 = 81% of 10 = 8 kn headwind and ground speed = 67 knots. (b) Segment 2: track = 061° w/v = 060/10 kn: angle = 0; 115 –0 = 100% of 10 = 10 kn headwind and ground speed = 65 knots. (c) Segment 3: track = 040° w/v = 060/10 kn: angle =20; 115 –20 = 95% of 10 = 10 kn headwind and ground speed = 65 knots. Step 5: estimate the time interval for each leg dividing the distance by the ground speed and converting to minutes; thus: (a) Segment 1: distance = 74 nm; speed = 67 kn: 74/67 × 60 = 66 minutes. (b) Segment 2: distance = 52 nm; speed = 65 kn: 52/65 × 60 = 48 minutes. (c) Segment 3: distance = 33 nm; speed = 65 kn: 33/65 × 60 = 30 minutes. Sum the segment ETIs to produce the estimated time en route [ETE]: 144 minutes. Step 6: calculate the total fuel needed including: (a) The fuel consumed from start-up at the departure airfield to the set-heading point (10 minutes) (b) Extra fuel consumed in the climb (two minutes per 1000 feet = 6 minutes, refer to fuel planning) (c) The summed ETIs (144 minutes) (d) Fuel consumed in the circuit and landing at the destination airfield, including an allowance for delays in the circuit (10 minutes) (e) The fixed 45-minute reserve The total is 215 minutes — well within our full fuel endurance of 240 minutes and giving us 25 minutes margin on top of our fixed 45-minute reserve. We will use the fuel log during the flight. Fuel calculation and fuel log Cruise fuel flow: 16 litres/hr Usable fuel loaded: 64 litres Endurance: 240 mins Estimate Actual Airfield departure: 10 mins Climb penalty: 6 mins En route: Oxford – Warraway: 66 mins Warraway – road junction: 48 mins Road junction – Tottenham: 30 mins Estimated time en route: 144 mins Airfield arrival: 10 mins Fixed reserve: 45 mins Total fuel required: 215 mins Fuel margin (endurance–total required) 25 mins Completed flight plan Segment Altitude Distance Track (mag) Heading (mag) Ground speed ETI Comms Oxford – Warraway 3500 74 083° 079° 67 66 ML 124.9 Warraway – road junction 3500 52 050° 050° 65 48 ML 124.9 Road junction – Tottenham 3500 33 029° 031° 65 30 ML 123.9 QNH: 1027 Last light: 1755 hrs AEST Fuel margin: 25 mins 4.5.4 Marking the chart plot To assist in the in-flight calculations necessary to assess divergence from the required track, it is advisable to add drift lines to the plot and mark the halfway point on each segment. The drift lines are usually dashed lines drawn diverging 10° either side of track from the departure point or turning points, and converging 10° either side of track into turning points or the destination. It is also advisable to add distance marks to the track that roughly equate to ten minutes flight time, say 10 nm intervals for an ultralight. The distance marks can commence from the departure point/turning points or backwards from the turning points/destination, according to personal preference. Alternatively you may prefer to annotate the distance of particular landmarks along the track, from the set-course point or a turning point. Also mark at least one ground speed checkpoint on each segment, maybe 15–20 nm along the leg. A feature that crosses the required track more or less at right angles is quite handy, as the track made good is likely to differ from the track required. These chart markings are shown in the 'En route adjustments' module. The chart(s) and flight plan plus the means of keeping an in-flight log must, of course, be carried in the aircraft; a kneeboard is a handy device. The last part of our flight planning and preparation is to conduct a safety audit of our preparations (remember – Proper Preflight Planning Precludes Piss-poor Performance), but before going on to that I suggest you read a couple of articles contained in the online version of CASA's magazine Flight Safety Australia: ~ 'Visual flight in marginal weather' — July - August 1999 issue. ~ 'Low cloud - Pressing on?' — April 1999 issue. There are many articles of interest to recreational pilots in Flight Safety Australia. A categorised index of such material is available on this site. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.4.1 Vectors and the wind triangle Velocity vectors We know that an aircraft in flight is airborne, and consequently both the path it projects over the ground and its speed relative to the ground are the resultant of the aircraft velocity and the wind velocity. Those velocities are vector quantities having both magnitude (speed) and direction in azimuth, so we can add the two velocities together to produce a resultant vector representing the aircraft's groundspeed and its track over the ground in azimuth. It is common practice to do that non-mathematically by drawing scaled, arrowed lines to represent each vector quantity. The lengths of the lines represent the magnitude (speed) of each vector, and the placements indicate the application points and the directions of motion; the resultant vector represents the aircraft track over the ground and the groundspeed. For example, waypoint Beta is 150 nautical miles north-east (045° true) of waypoint Alpha and an aircraft departs overhead Alpha for Beta, maintaining a heading of 045° true while flying at the aircraft's normal cruising speed of 75 knots TAS. At the time of departure, the wind velocity at the cruise altitude is 135° true at 20 knots; i.e. the 20 kn wind is coming from the south-east. Where will the aircraft be after two hours flight? Certainly not over Beta, as it will have moved 150 nm north-east within the air mass while the air mass has moved 40 nm north-west. So we might surmise that after two hours flight its position will be about 40 nm north-west of Beta, and this is shown in Figure 1. The aircraft has drifted from its intended path or track over the ground and the 'track made good' is about 15° to the left of the 'required track'. We should note that, relative to the aircraft's course, the wind velocity normally has both a crosswind component and a headwind or tailwind component, and that headwind or tailwind component will also affect the aircraft's speed relative to the surface — the ground speed. (I have used the ISO standard symbol 'kn' for knot in the diagrams; the symbol 'kt' sometimes seen is the standard symbol for kilotonne.) Note: in the USA the term 'course' is synonymous with 'track' for air navigation, but the International Civil Aviation Organisation (ICAO) preferred usage is 'track'. The heading is the direction with which the longitudinal axis of the aircraft is aligned at any given time. This heading may be expressed as relative to true north — the true heading; or if adjusted for magnetic variation — the magnetic heading; and if adjusted for variation plus compass deviation — the compass heading. The wind triangle So, if we want to track over the direct route from Alpha to Beta we will have to ascertain both the expected wind velocity at the time of flight and a heading to fly that will provide the necessary crosswind correction angle. In the 3-vector wind triangle only the wind vector is completely known — the forecast wind velocity 135°/20 kn. We know part of the air or heading vector — the true airspeed 75 knots — but not the direction. We also know part of the ground vector — the direction (ground track 045° true) from Alpha to Beta — but not the ground speed. We can determine the two unknowns — the heading and the ground speed — by plotting scaled vectors on paper (figure 2). You will need some drawing instruments, a protractor and ruler, but a pair of dividers can be useful. • Draw a line connecting v1 and v2, marking it with one arrow to represent the heading vector and measure the line's orientation with true north with the protractor to determine the heading (060°T). Thus we have the first unknown — the direction in which to point the aircraft. Annotate the heading (060°T) and TAS (75 kn). Also note the wind correction angle [WCA] — the difference between the required track (045°T) and the heading (060°T) — is 15°, and the drift will be to the left — also known as port drift. Note: the wind correction angle is the angular difference between the required track and the heading, intended to ensure that the track made good will equate with the required track. Note that the terms 'crab angle' and 'drift angle' are very often used instead of 'wind correction angle'. But the latter term is more precise; crab angle and drift angle do have slightly different meanings or associations. Drift angle is measured in flight, and is the angle between the heading and the track made good. Crab angle is the preferred term when associated with crosswind landing. • Now measure the distance between Alpha and v2, which is the distance (72 nm) moved over the ground during one hour. This is the second unknown — the ground speed. Annotate the ground speed (72 kn) adjacent to the bearing (Figure 3). • We can now calculate the sector flight time from overhead Alpha to overhead Beta; this time is called the estimated time interval [ETI]. ETI (minutes) = Distance (nm) / ground speed (kn) × 60 = 150/72 × 60 = 125 minutes. It is interesting to note that even though the wind is a full crosswind to the track required, the ground speed is less than TAS and thus the ETI is a bit greater than you may have expected. This is because the heading of 060° would now include a small headwind component. Direct headwind/tailwind If the wind is aligned directly with the required track then of course it is not possible to construct the triangle, as there is no wind correction angle and the ground speed is the TAS ± wind speed. However, just as an illustration that the wind triangle still provides the correct answers, I have repeated the previous Alpha to Beta plot with winds that are only 10° off the required track; i.e. nearly full headwind and tailwind components. It may be thought that if an out-and-return trip is flown where the wind is directly aligned with the required track, the headwind encountered in one direction will be offset by the tailwind in the reverse direction; thus the total flight time will be equivalent to that in nil wind conditions. Not so — the greater the wind speed the greater the flight time on an out-and-return flight, no matter what the wind direction. Imagine a flight Alpha–Beta–Alpha in nil wind conditions. The ground speed on both the 150 nm outward and return legs would equal the TAS (75 kn) and each leg would take 120 minutes for a total flight time of 240 minutes. Now let's factor in a 25-knot north-east wind. The ground speed on the outward leg would be 50 kn and the ETI would be 180 minutes, whereas the ground speed on the return leg would be 100 kn and the ETI 90 minutes for a total flight time of 270 minutes. 4.4.2 Estimating heading and ground speed Plotting the wind vector triangle is the most accurate method for ascertaining heading and ground speed, but there are two other methods that are quite accurate enough for light aircraft cross-country navigation – use of the 1-in-60 rule and the use of tables. But first a quick look at trigonometrical relationships. Trigonometrical relationships - sine and cosine The trigonometrical relationships of the two wind components — crosswind (that component of the wind velocity that acts at right angles to the track) and headwind/tailwind (that component of the wind velocity that acts inline with the track) — is shown in a modified wind triangle (Figure 5). The sine of an angle = opposite side/hypotenuse, while the cosine of an angle = adjacent side/hypotenuse. In this example the wind angle is 30° relative to the required track of 045° true and the wind speed is 20 knots; the hypotenuse represents the wind velocity vector, the side opposite to the wind angle is drawn from the start of the wind vector so that it forms a right angle with the track so representing the crosswind component of the wind velocity while the side adjacent to the angle represents the headwind component. Reading from the abridged trigonometric table below, sine 30° is 0.5 and cosine 30° is 0.866 — near enough to 0.9, thus the crosswind is 0.5×20=10 kn and the headwind is 0.9×20=18 kn. Abridged trigonometrical table Relationship between an angle within a right angle triangle and the sides: Tangent of an angle=opposite side/adjacent side Sine of an angle=opposite side/hypotenuse Cosine of an angle=adjacent side/hypotenuse Angle Sine Cosine Tangent Angle Sine Cosine Tangent 1° 0.017 0.999 0.017 50° 0.766 0.643 1.192 5° 0.087 0.996 0.087 55° 0.819 0.574 1.428 10° 0.173 0.985 0.176 60° 0.866 0.500 1.732 15° 0.259 0.966 0.268 65° 0.910 0.423 2.145 20° 0.342 0.939 0.364 70° 0.939 0.342 2.747 30° 0.500 0.866 0.577 75° 0.966 0.259 3.732 40° 0.643 0.766 0.839 80° 0.985 0.173 5.672 45° 0.707 0.707 1.000 90° 1.000 0 infinity In wind triangle plots we assume that the forecast wind velocity is accurate and constant, the aircraft's magnetic compass is accurate, and the pilot will maintain a constant heading in flight. However, there will be considerable variability in each (for example read the boundary layer turbulence paragraphs in the microscale meteorology module), so there is no reason to try for absolute accuracy in the initial calculation of heading, ground speed and ETI. So, rather than plotting the wind triangle we can introduce a few shortcuts to the process by using some simple mental arithmetic to estimate the crosswind and headwind/tailwind components of the wind velocity relative to the required track. Even so, it is wise to become familiar with plotting the wind triangle; the experience makes it much easier to mentally envisage the relationship between the vectors thus avoiding flying entirely in the wrong direction — which is remarkably easy to do. The 1-in-60 rule The 1-in-60 rule provides a rule of thumb based on the reasonably accurate assumption that the sine of any angle, up to about 45°, is equal to 0.01666 times (or 1/60) the number of degrees; e.g. sine 30° is 0.01666 × 30=0.5 or 30/60 = 0.5. The sine is the ratio — in any roughly right-angle triangle — of the side opposite the angle to the hypotenuse (the longest side). Thus the 1-in-60 rule is very handy in the mental arithmetic of flight theory and basic navigation, as the angles involved in en route corrections are usually much less than 45°. For angles up to 15° or 20° the tangent (opposite side/adjacent side) is practically the same value as the sine. For angles between 50° and 75° the sine is about 1/70 times the number of degrees, and for angles between 75° and 90° the sine approaches unity. Using 1-in-60 to estimate WCA The two/three-step technique described below approximates the sine/cosine relationships and produces results near enough to the trig calculations. • Step 1. First find the crosswind component of the forecast wind velocity by estimating the acute angle (i.e. less than 90°) at which the wind meets the required track, divide that by 60 and multiply the result by the wind speed. However, if that relative angle exceeds 60° just use 60. For example: (a) track = 045° w/v = 075/20 kn: relative angle = 30 = 30/60 × 20 = 10 kn crosswind. or (b) track = 045° w/v = 135/20 kn: relative angle = 90 [use 60] = 60/60 × 20 = 20 kn crosswind. or (c) track = 045° w/v = 195/20 kn: relative acute angle = 30 = 30/60 × 20 = 10 kn crosswind. • Step 2. Then use the 1-in-60 rule to estimate the wind correction angle by dividing the crosswind component by the TAS and multiplying the result by 60. For example: (a) and (c) crosswind = 10 kn; TAS = 75 kn: 10/75 × 60 = 8° WCA. or (b) crosswind = 20 kn; TAS = 75 kn: 20/75 × 60 = 16° WCA. But combining steps 1 and 2 simplifies the calculation: WCA = relative angle [60 max] x wind speed / TAS Example (a) track = 045° TAS = 75 kn; w/v = 075/20 kn: relative angle = 30 WCA = 30 × 20/75 = 8° And remember that the wind correction is applied in the direction the wind is coming from so that the aircraft crabs along the required track. • Step 3. Then to estimate the ground speed, deduct the (acute) angle at which the wind meets the track from 115 (for angles up to 60°, use 105 for greater angles) and apply that as a percentage of the wind speed. For example: (a) track = 045° w/v = 075/20 kn: angle = 30; 115 – 30 = 85% of 20 = 17 knots headwind. or (b) track = 045° w/v = 135/20 kn: angle = 90; 105 – 90 = 15% of 20 = 3 knots headwind. or (c) track = 045° w/v = 195/20 kn: angle = 30; 115 – 30 = 85% of 20 = 17 knots tailwind. Subtract the result from TAS if wind is coming from ahead to abeam, otherwise add. If you like to try a quick mental calculation with the two plots in Figure 4, you will find the arithmetic will produce much the same results as the plots. You may think it wrong that if the wind is at 90° to the track the ground speed calculation will still come up with a headwind component. This is because the track and the wind velocity are relative to the ground, not to the aircraft's heading. With a wind at 90° to the required track the aircraft must take up a heading having some into-wind component, so that it crabs along the required track; try it by plotting a full wind vector triangle incorporating a wind at 90° to the required track. All the short-cut techniques described are not ultra-precise but they are quite okay for most cross-country navigation in visual meteorological conditions. You should also read the meteorology module dealing with southern hemisphere winds and particularly section 6.3. Using tables to derive ground speed and WCA The third and simplest method for estimating WCA, heading and ground speed is to use tables such as those following. Table 1 is for wind speeds up to 30 knots in 5-knot intervals, and for wind angles relative to either side of the required track between 0° and 180°. In the table you will see that headwinds have a negative adjustment and tailwinds a positive adjustment for ground speed. However if the calculated WCA exceeds about 10° the inbuilt crab problem becomes apparent and a small additional calculation to derive a more accurate ground speed has to be made (Table 2). Note that the sum of the two wind components only equates with the wind speed when the wind angle is 0°, 90° or 180°. Table 1. Wind components Head/tailwind component in knots (for ground speed) Crosswind component in knots (for WCA) WA Wind speed Wind speed 5 10 15 20 25 30 5 10 15 20 25 30 0° -5 -10 -15 -20 -25 -30 0 0 0 0 0 0 15° -5 -10 -15 -20 -25 -30 1 2 4 5 6 7 30° -4 -9 -13 -17 -21 -25 2 5 7 10 12 15 45° -3 -7 -10 -14 -17 -21 3 7 10 14 17 21 60° -2 -5 -7 -10 -13 -15 4 9 13 17 21 25 75° -1 -2 -4 -5 -6 -7 5 10 15 20 25 30 90° 0 0 0 0 0 0 5 10 15 20 25 30 105° +1 +2 +4 +5 +6 +7 5 10 15 20 25 30 120° +2 +5 +7 +10 +13 +15 4 9 13 17 21 25 135° +3 +7 +10 +14 +17 +21 3 7 10 14 17 21 150° +4 +9 +13 +17 +21 +25 2 5 7 10 12 15 165° +5 +10 +15 +20 +25 +30 1 2 4 5 6 7 180° +5 +10 +15 +20 +25 +30 0 0 0 0 0 0 5 10 15 20 25 30 5 10 15 20 25 30 ground speed* = TAS + value shown WCA = value shown / TAS × 60 *If the WCA exceeds 10° then reduce the ground speed by an additional value that is a percentage of the TAS, as shown in Table 2. You will note that the adjustment to ground speed really only becomes particularly significant at WCAs above 20° and then, in such conditions, it is probably unwise for light aircraft to be engaged in cross-country flight. Table 2. Ground speed adjustment applied if WCA exceeds 10° WCA 10° 15° 20° 25° 30° Reduction 2% 3% 6% 10% 12% Example 1. The track required is 090°, the wind velocity is 060°/15 knots and TAS is 70 knots. Then the wind angle relative to track is 30° left and, reading from Table 1, the headwind component is –13 and the crosswind component is 7. Thus the ground speed will be 70 –13 = 57 knots, the wind correction angle will be 7/70 × 60 = 6° (to the left) and the heading = 084°. Example 2. The track required is 300°, the wind velocity is 075°/15 knots and TAS is 70 knots. Then the wind angle relative to track is 135° right and, reading from Table 1, the headwind component is +10 and the crosswind component is 10. Thus the ground speed will be 70 + 10 = 80 knots, the wind correction angle will be 10/70 × 60 = 8° (to the right) and the heading = 308° Example 3. The track required is 360°, the wind velocity is 075°/20 knots and TAS is 70 knots. Then the wind angle relative to track is 75° right and, reading from Table 1, the headwind component is –5 and the crosswind component is 20. Thus the ground speed will be 70 – 5 = 65 knots, the wind correction angle will be 20/70 × 60 = 16° (to the right) and the heading = 016°. However, because the WCA exceeds 10°, Table 2 is consulted. This shows for a WCA of 16° the ground speed should be further reduced by 3% of the TAS — about 2 knots, so the adjusted ground speed is 63 knots. 4.4.3 Navigation calculators Circular slide rules There are several 'do everything' circular slide rules, or 'whiz wheels', marketed for aircraft flight planning usage. These navigational 'computers' also incorporate a wind disc for the solution of the wind triangle. They too find the wind triangle solution by breaking the wind velocity into the crosswind/headwind components, rather than plotting a full wind vector triangle. So the adjustment to derived ground speed for WCAs exceeding 10°, similar to that shown in Table 2, is also an additional step. You will find them very useful on the ground but some can be difficult to read and adjust in a light aircraft, particularly in an open cockpit. You may have enough difficulty just handling the chart, the flight plan notes and a pencil (sharpened both ends). The Jeppesen CR2 — available from the Airservices Australia online store navigation and planning accessories — for about $60 is okay and will fit into your pocket — together with a small folding rule. It can be operated with one hand for time and distance calculations. E-6B calculations 'E-6B calculations' (or just E6B) is now a generic term for all the calculations associated with tracks, TAS, wind velocities, headings, ground speeds, density altitude, time, fuel, weight and balance and so on. The term derives from one of the many model numbers of a hand-held dead reckoning instrument generally known as the Dalton computer. It was extensively used by all the Allied air forces in WW2 and consisted of a circular slide rule with either a fixed or sliding wind scale or a wind scale belt looped inside an instrument box. All subsequent navigation circular slide rules are developments of Dalton's dead reckoning computers. There are E-6B software apps for smartphones, tablet computers and other personal electronic devices, readily available for a few dollars or possibly as freeware. To find sources, google 'E6b software'. However it is my opinion that the whiz wheel does provide a better grasp of the essentials of the wind triangle. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.3.1 The four navigation techniques for flight under the visual flight rules Pilotage Pilotage is navigation by visual reference to landmarks — the art of visual track-keeping — which requires that the ground is generally continually in sight. In the early days, all air navigation was by pilotage with some crude dead reckoning. Indeed the first Pilots' Directions published by Elrey B. Jeppesen in the 1920s, for the early air mail pilots in the USA, were just notes about the landmarks along a route. As accurate aerial charts became available then aerial dead reckoning became much more refined. Map reading is the essence of pilotage. It entails: a continuous in-flight survey of the planned route (pre-plotted on the chart); identification of the upcoming chart features on the ground (i.e. reading from map to ground); and determining the actual location relative to the planned position. Following the determination of that position (and thus the actual path over the ground) dead reckoning is then used to determine the 'navigation solution': the ground speed and the track error (the angular difference between the intended route and the actual path) the subsequent course correction(s) necessary to regain and maintain the intended route a revised arrival time. Only when uncertain of your position will it be necessary to note prominent ground features and their relative positions, and then find those features on the map; i.e. reading from ground to map. Map interpretation is an acquired skill. An inability to relate the map to the ground features in view is a common experience on the initial attempts. Some find it very difficult to master. In the more remote, and rather featureless, areas of Australia what seem to be major features on the surface may not be shown on the chart, and vice versa. Dead reckoning Dead reckoning [DR] is deriving the current position, or a future position, mathematically from a planned position or the last known position. DR for light aircraft is, or should be, essentially simple navigation by clock, compass and mental arithmetic. Most of the DR for RPT and military aircraft is done within the electronic circuitry of advanced navigation systems such as inertial navigation systems [INS], which calculate a new position, from the previous position, about 100 times per second. DR has a limitation in that errors in plotting or wind velocity estimation or course steering and timing are cumulative, and the true position of the aircraft can't be verified unless it can be determined by pilotage (landmark reference) or some other position fixing technique. Supplementary technique: position-fixing Aeronautical position-fixing techniques are usually radio-based. They encompass simple techniques such as plotting the intersection of the bearings from two radio beacons, through to more complex systems such as VOR/DME which is both position-fixing and homing. Such systems usually incorporate some degree of electronic DR. The Global Navigation Satellite System [GNSS] is a continuous position-fixing or electronic pilotage system plus electronic dead reckoning to calculate the new 'heading to steer' to the next waypoint. The use of GNSS by sport and recreational VFR pilots as a supplementary navigation system is described in the 'En route navigation using the GNSS' module. The non radio-based position-fixing techniques are celestial — star sights or sun sights. Charles Lindberg's pioneering 33.5 hour 1927 New York-Paris flight was mostly dead reckoning with some star sightings, when he could see them. Supplementary technique: homing VFR homing is radio-based and encompasses non-directional radio beacon [NDB] and VHF omnirange [VOR] homing; these are described in a 'Supplementary navigation techniques' section. Note: in addition there is a module describing 'Electronic flight planning and the electronic flight bag'. 4.3.2 Creating a route Preliminary route Having decided a destination, the first step is a rough calculation to ascertain the track (the planned path over the ground), the 'distance to run' from your departure point, the approximate sector time and the viability of the proposed flight. The charts needed are a current WAC (or VNC) or adjoining WACs (or VNCs) and — if the departure point, route or destination is under the steps of a CTR — the VTC or VNC. You could also use a digitised NATMAP 250K map on a Windows desktop or laptop in the initial planning stage when you need more surface detail than that provided by a WAC. Google Earth may also be handy in locating or verifying the position of small private strips/ landing grounds. Route construction is often done the day before planned departure, or even earlier if an extensive cross-country flight is planned. With a soft pencil and a rule, draw a preliminary line on the chart between your departure and destination — you may have to overlap charts, but be aware that WAC and VNC are at different scales. Check along the line for areas to be avoided; i.e. 'tiger country' — rough, forested or hilly areas where there is a limited availability of open, cleared, flat land for an emergency landing. If possible, avoid long stretches of featureless terrain and also designated remote areas. If you are using a WAC, check the relevant ERC-L for CTR, PRD and CTAF aerodromes and mark them on the WAC. Note any other airfields near the line. Now decide which areas of terrain to avoid and find a suitable diversion around them. If that diversion takes you quite a distance from the direct line then so be it; it won't make that much difference to the total distance flown. If there are areas of scenic or other interest evident on the chart, you might plan to overfly them — even if it does makes a zigzag path. Tracking around and beneath controlled airspace If your intended track is within the area covered by a VTC you must examine the current VTC for the mandated VFR routes for aircraft flying in Class G and plan to follow those routes. They are indicated as a line of large purple dots. Carefully check the VTC and current ERSA for the altitude at which these routes should be flown. You may find, for instance, that some coastal routes require flight in one direction at 500 feet amsl and at 1000 feet for the opposite direction. The Airservices Australia Flying Guides and Publications contains a lot of information to assist in planning flights around Sydney, Melbourne, Brisbane, Canberra, Adelaide and Perth to avoid violations of controlled airspace; look for the link to 'Visual guides'. Note that on VTCs any area where the elevation does not provide at least 500 feet clearance between the terrain and the lower limit of the overlying CTA is tinted purple; such areas must be avoided. You may also find that when threading your way around CTRs, the clearance between the terrain and the overlying CTA may be so limited that all aircraft in Class G would be flying at much the same low height and tracking over the same ground, — this provides the conditions for a mid-air collision. Also in such terrain there is a significant possibility of strong lee downflows. Never plan to fly such routes unless a reasonable visibility is forecast and the winds around 3000 feet amsl are below 20 knots. The Australian Transport Safety Bureau Web site contains a research report in PDF format Limitations of the See-and-Avoid Principle, which is recommended reading. When planning to track near a CTR be aware that you must apply a tracking tolerance — offsetting it at least one nautical mile from the boundary of controlled airspace — if flight is planned below 2000 feet agl, or two nautical miles if between 2000 and 5000 feet agl; watch out for the overlaid CTA steps. A VHF radio is advisable when planning to operate close to a CTR because it is good airmanship to let them know you are there — if a heavy RPT aircraft is being let down overhead there is always a chance of being caught in sinking wake turbulence. Also if you do get caught between rising terrain and a lowering cloud base you can always call Air Traffic Control and inform them that you "require entry" to pass through the edge of the CTR because of deteriorating weather. ATC are always very helpful but unauthorised entry into the CTA or CTR (the dreaded 'violation of controlled airspace' or VCA) is a safety hazard and may earn a substantial fine. Read the article 'Lost in controlled airspace' in the online version of CASA's magazine Flight Safety Australia: November - December 2001 issue. Also see Airservices Australia's document 'Safety net: safe operations around controlled airspace'. Waypoint selection The VFR rules (ERSA ENR 1.1 para 19.2) state 'the pilot must positively fix the aircraft's position by visual reference to features shown on topographical charts at intervals not exceeding 30 minutes.' You need to find readily recognisable point locations or waypoints for monitoring flight progress and/or to mark the points of diversion and consequent turning points. Suitable waypoints are airfields with formed runways, major road junctions, small towns, grain silos near rail lines, intersecting line features and distinctive permanent water features — though in drought conditions such features may not be obvious. You may also see some highly visible linear features — roads, railways, rivers, beaches — that roughly parallel your intended track for a reasonable distance. Plan a track divergence to intercept and then follow such line features — and be aware of the 'Rules of the Road' that require aircraft to track to the right of a line feature, or when flying within a valley or any air traffic lane. In the more remote areas of Australia the distances between verifiable landmarks are great and in such cases the only viable route is to follow sealed roads. Mark all the turning points on the chart, joining them to form the route segments of the required track. These turning points will also be used as fuel consumption checkpoints. Generally speaking, a route that provides the best visual fixes and reasonably short segments is the best option. Measure the total track distance using the scale (in nautical miles) printed on the map or alternatively use the latitude graticule printed along the meridians; each mark is one minute of latitude or one nautical mile. The printed scale is easier to read and thus less prone to errors. (You can buy a ruler scaled in nautical miles for use with WACs, VNCs and VTCs online from the Airservices Australia online store navigation and planning accessories — and buy a protractor at the same time. ) Divide the total track distance by the cruise speed to get an approximate total time required. If the total time required is greater than the known maximum sector time, then the flight must be broken into two or more sectors by introducing refuelling stops at appropriate distances. This probably necessitates replanning the waypoints so that one or more coincide with an airfield with assured and suitable fuel supplies. Re-plot the route if necessary. If the total time required is less than the maximum sector time, then the first-cut plan for the route to be followed may be viable — but we have not yet taken into account the effects of wind, which may be considerable; these are covered in the next module. Forecast weather and winds should be ascertained as close to the planned departure time as possible, but it is advisable to obtain a preliminary weather forecast the evening before the flight. If a very long flight is planned it is advisable to watch the weather patterns for a few days prior to the trip. Any NOTAM applicable to the area in which you intend to operate should also be obtained at that time. See section 3.6. 'I follow roads' There is a pertinent catch-phrase amongst navigators: "Hold the heading and fly the aeroplane!". It is not possible to consistently maintain an exact compass heading in a very light aircraft at lower altitudes where the atmosphere is more turbulent, but if the variations produce a mean heading near enough to that planned there should be no difficulty. The pilot should be able to maintain the heading within ±5° or so. It is only when the aircraft is allowed to consistently wander 10° or 15 ° off course for 10 minutes at a time that planned DR navigation goes out the window and you would be better just following roads, railways or power transmission lines from landmark to landmark. In some circles that's termed IFR — not Instrument Flight Rules but 'I Follow Roads/Railways' meaning you plan to keep prominent line features in sight throughout the flight. It's a good philosophy if you are only interested in pilotage and not the challenge of dead reckoning. This purely pilotage technique doesn't preclude the requirement for proper pre-flight planning — it's just that there will tend to be a lot more heading changes than otherwise, and estimation of ground speed and re-estimation of arrival times are a bit rougher. The 'I Follow Roads' technique is different from the need to plan dead reckoning flights in remote areas of inland Australia so that they track along or near major roads — which may be the only verifiable landmarks in otherwise featureless or uniform terrain. There is a corollary in that aircraft operating under the Instrument Flight Rules must also track along designated air routes when flying from A to B, and these air routes may not be the straight line track between departure and final destination. 4.3.3 Airfield check Classification and communications Civilian airfields are classified as 'certified' [CERT] or 'registered' [REG] or 'aircraft landing area' [ALA]. Except with prearranged permission, RA-Aus registered aircraft may not enter controlled airspace (other than Class E) or a control zone. An aircraft may only enter the vicinity of, or land at, a certified or registered aerodrome if equipped with a serviceable VHF radio. The pilot, with an appropriate radio endorsement, must make the specified broadcasts and ensure that the appropriate frequency is used. Any non VHF-equipped aircraft may, with due care, land at any civilian CTAF airfield which is not certified or registered. Some aerodromes have a locally operated facility — a universal communications facility [UNICOM] usually on the CTAF (but it may be on another frequency) — which provides information on local conditions. If such a facility is operating at a CTAF airfield it is possibly unwise to enter the circuit if not VHF-equipped, as other aircraft operating in the area may not be as vigilant as they should. For more information see R/T communications and procedures in the 'VHF radiocommunications guide'. Public airfields are usually owned by a local government body and landing permission is generally not required, although it is always wise to check. Private airfields usually cannot be used without prior permission from the owner, except in an emergency — even then there may be problems with trespass. Landing and parking charges apply at many airfields. If the airfield is not shown in ERSA it will be a small private operation — possibly with a listing in the Australian Aircraft Owners and Pilots Association publication 'Airfield Directory', which has details of about 2000 airfields, including whether prior landing permission is required. If an airfield is not listed in ERSA or the AOPA Airfield Directory, then it is most unwise to contemplate using it without contacting the owner. Even if landing permission is not required, you should always pre-check with the owner/operator about hazards and conditions. It is too late to find out the surface has been softened by rain when you are up to the axles and about to flip over or find out about the wire across the landing path by striking it. Using a Google Earth image — found by the location latitude and longitude coordinates — may be a useful source of visual information for airstrips that don't appear in ERSA. Legislative requirements The regulations must also be considered when planning a landing at an unfamiliar airfield, or indeed a familiar airfield. CAR 92 'Use of aerodromes' states in part: A person must not land an aircraft on, or engage in conduct that causes an aircraft to take off from, a place that does not satisfy ... the following requirements ... is suitable for use as an aerodrome for the purposes of the landing and taking-off of aircraft ... and, having regard to all the circumstances of the proposed landing or take-off (including the prevailing weather conditions), the aircraft can land at, or take-off from, the place in safety. The civil aviation advisory publication CAAP 92-1 (1) 'Guidelines for aeroplane landing areas' expands the CAR 92 'circumstances' to be considered by ' setting out factors that may be used to determine the suitability of a place for the landing and taking-off of aeroplanes. Experience has shown that, in most cases, application of these guidelines will enable a take-off or landing to be completed safely, provided that the pilot in command: (a) has sound piloting skills; and (b) displays sound airmanship'. CASA have produced two advisory publications to support CTAF procedures and provide guidance on a code of conduct to allow greater flexibility for pilots when flying at, or in the vicinity of, non-towered aerodromes. These Civil Aviation Advisory Publications (available on this website) are: CAAP 166-1 'Operations in the vicinity of non-controlled aerodromes' and CAAP 166-2 'Pilots responsibility in collision avoidance in the vicinity of non-controlled aerodromes by 'see and avoid'. Note that the 'ultralight' term used in the CAAPs when recommending a 500 feet circuit height, refers only to those minimum aircraft which have a normal cruising speed below 55 knots, or thereabouts. CASA has produced an online interactive learning tool titled 'Operations at, or in the vicinity of, non-controlled aerodromes' which is now available at CASA online learning. Also read 'Operations at non-controlled airfields' and 'Safety during take-off and landing'. The current ERSA should be fully consulted; particularly check the circuit procedures, stated hazards and whether the airfield is certified or registered, thus requiring use of VHF radio. Hazards You must be aware of your aircraft's landing (and subsequent take-off) performance in normal, soft field and short field conditions. You must also perform a safety audit of the destination and alternate airfields for length, slope, surface condition (e.g. roughness, mud, surface water), approach and go-around hazards, stock and wildlife hazards, tyre puncture and wheel hazards, and any commonly occurring micro-meteorological and dust hazards. Check runway directions and expected wind conditions, and be wary of airfields with single runways; crosswind conditions may be beyond your aircraft's capability. Be particularly wary of airfields with 'one-way' strips — they are extremely tricky, if not outright dangerous, for those not familiar with any topographic turbulence, sink or other atmospheric hazards that could exist. Low-lying strips may be badly softened by rain or inundation. The availability and location of suitable fuel should be checked. Remember, just because your assessment concludes that you can safely land at a particular airfield it does not guarantee that you will be able to take-off safely. Note: ERSA location indicator codes. all Australian licensed, and most unlicensed, airfields are assigned a four-letter identity, where the first letter is 'Y' (e.g. Albury = YMAY). Navigation aids such as an NDB or VOR have a three-letter code (e.g. Eildon Weir VOR = ELW) and visual waypoints marked on charts have a three or four-letter code (e.g. Eldorado = ELDO). 4.3.4 Fuel planning The need for maintaining fuel reserves: In Australian general aviation and recreational aviation there is about one reported aircraft accident or incident per week that is caused by fuel exhaustion (all fuel on board consumed) or fuel starvation (mismanagement of the fuel system so that available fuel is blocked from delivery to the engine) — please ensure you are not this week's statistic. Before undertaking a cross-country flight, the pilot must know the total usable fuel capacity and the rate of consumption at the planned cruising speed. The fuel consumption rates supplied by engine/aircraft manufacturers, unless contained in a formal pilot's operating handbook, must be viewed somewhat sceptically; they may be achievable with an 'as new' engine cruising at the best endurance power setting, but are not reflective of the consumption at a more useful cruise speed, say that at 75% power. Fuel must be allowed for consumption at the departure airfield, for the climb and for circuit delays and landing at the destination or an alternate airfield. In addition, the pilot is required to plan a fixed fuel reserve. The reserve amount planned is a matter of personal discretion and the capacity of the fuel tank. It should not be less than 30 minutes in good flying conditions but a greater amount — perhaps 60 minutes — when there is any doubt about the wind velocities or other conditions.* This reserve should not be planned for use; i.e. whether the aircraft is finally landed at the planned destination or the alternate airfield there ought to be at least 30 minutes fuel in the tanks. The fixed fuel reserve concept still applies even if the planned flight is just a local flight terminating at the departure airfield — or a session of circuits and touch'n go's. *Note: The CASA civil aviation advisory publication CAAP 234-1 'Guidelines for aircraft fuel requirements' provides information and guidance on the fuel requirements for aircraft required by CAR 234. CAAP 234-1 suggests a 45 minute fixed fuel reserve for piston engine VFR aeroplanes. For recreational aircraft perhaps 45 minutes for engines of 70 hp and above and 30 minutes fixed reserve for the smaller engines (the 2-stroke and 4-stroke half-VW designs of 'low-momentum ultralight aeroplanes' tend to provide less 'time in the tank'), but read CAAP 234-1. Fuel consumption It is vital to be able to measure fuel consumption during flight, so a reasonably accurate fuel contents gauge, sight gauge or an in-flight view of the fuel tank content is necessary. It is good practice to maintain a history log in the aircraft where the actual fuel consumption per flight hour is entered at the conclusion of each flight. A consumption history log provides valuable information, both for future flight planning and for discerning engine performance trends. When planning a cross-country flight, the objectives are to arrive at the planned destination safely with a reasonable reserve of fuel in hand and without affecting the safety of others while en route; or even creating a possibility that safety might be affected. But remember the first rule of aviation — fly the aeroplane at all times, navigate when able and always be a few minutes ahead of the aeroplane. When navigating a very light aircraft, and particularly an open-cockpit ultralight, a person's capacity for mental arithmetic is not as good as it is when sitting at home. Nor is it easy — or maybe even possible in a weight-shift control aircraft — to manipulate navigation tools in flight and it is very difficult to handle charts, pencils and notepads in the cockpit. Pre-flight preparation should be directed towards reducing and simplifying the in-flight work load. You should have a good acquaintance with the flight envelope of the aircraft, both with and without a passenger. In particular you must know the optimum cruise speeds obtained when cruising at, say, 75% power plus the proven fuel consumption, in litres per hour — at that throttle setting and aircraft weight. Calculate the maximum sector time allowable by dividing the total usable fuel capacity by the hourly consumption to find hours; then deduct 30 minutes reserve fuel to arrive at the maximum advisable sector time. For example let's say our aircraft has a fuel capacity of 66 litres with 64 litres usable; proven consumption at 70 knots normal cruise is 16 litres/hour. Then maximum sector time is 64/16 = 4.0 hours or 240 minutes; less 30 minutes fuel reserve = 210 minutes. Never equate fuel consumption with distance, only time. Light aircraft consume 40% or 50% more fuel in a maximum power climb than at a normal cruise setting. It is normal practice to initially climb away at best rate of climb speed (Vy) until a safe height is reached, then airspeed is allowed to increase to a suitable en route climb speed, while maintaining maximum allowed climb power, until the cruise altitude is reached. The extra fuel consumption during the climb can be estimated from the normal rate of climb achieved. For example, rate of en route climb 250 feet/minute = four minutes per 1000 feet, then extra fuel consumed (~50%) is two minutes fuel per 1000 feet climbed. This extra fuel will be used whatever power setting is used in the climb; it is the chemical energy exchanged for the potential energy of height. There are several articles in the online version of CASA's magazine Flight Safety Australia that are recommended reading. Look under 'Fuel management' in our categorised index of the articles of particular interest to recreational pilots in Flight Safety Australia. 4.3.5 Plotting the route on a chart Reading the chart Shown below is the route we plan to fly from an airstrip on a rural property — Oxford — to an airfield at Tottenham (YTOT). Looking at the chart, the straight (dark brown) line distance is 150 nm, but there is one section of 40 nm or so, at the western end of the higher ground, that traverses a region of hills and gullies. This area may not provide suitable emergency landing sites. (A larger 141 kb plot image is available. It will open in a new browser window.) The chosen route utilises the 100 nm of low (elevation about 300 feet amsl) country, extending eastward from the departure airstrip where atmospheric conditions are likely to be less turbulent, with an east-west railway as a good line reference. There are two sentinel hills (Warraway Mountain) south of the rail line and about 75 nm east of Oxford that will provide a distinctive landmark for a turning point. The elevations shown are 895 and 987 feet, thus rising about 600 feet above the surrounding plain. All elevations on the WAC are in feet. We also note that there are two good alternate airfields in the vicinity, Lake Cargelligo and Condobolin, and find that the latter is 58 nm east of Warraway Mountain while Lake Cargelligo is 20 nm south-east of that hill (off the map image). Checking ERC-L(5) we find there is no special use airspace — restricted or danger areas — in the vicinity of our planned operation. About 50 nm north-east of Warraway Mountain and at the top of the watershed is a distinctive road junction, suitable for the second waypoint. Note the figure 1584 in bold type, just above the road junction — this indicates the highest elevation (Mt Susannah) in that WAC grid section. Similarly the figure 1528 just to the right of the junction indicates the location of the highest elevation in the neighbouring grid section. Thus we will have good indication of track holding from quite a distance if we appear to be tracking towards a position midway between those high points. Also there is a road about 20 nm north-east of Warraway Mountain, which we will cross at right angles, to provide a good ground speed check. The last segment is a 30 nm run following the valley downslope direct to Tottenham, which, from ERSA, has an elevation of 780 feet. There is a cautionary note in ERSA that a significant animal hazard (kangaroos?) exists on the airfield. Tottenham should be readily recognised from a distance by the distinctive pattern of minor roads, the rail line coming from the south-east and terminating at Tottenham, plus the mine (indicated by the crossed pick and hammer symbol) and a large grain silo. The symbol for the latter is difficult to see but it is right against the western edge of the purple circle indicating the airfield. The total distance of the three route segments is about 155 nm, very little more than the straight line route and much easier pilotage. The approximate sector time will be 155 divided by our 70 knot cruise = 2.2 hours or 132 minutes, well within our maximum sector time of 210 minutes. Thus the flight will be viable — if the weather is favourable. Checking ERC-L(5) the relevant Melbourne FIS communications frequencies are ML 124.9 for the first two legs and ML 123.9 for the final. Quantifying the route data We can now measure the non-variable route segment data to initiate the flight plan: • Centre the protractor on Oxford, ensure that the protractor is aligned with the chart meridians and read off the bearing to the first waypoint — about 094° true. • Centre the protractor on Warraway Mountain and read off the bearing to the second waypoint — about 061° true. • Centre the protractor on the road junction and read off the bearing to Tottenham — about 040° true. • Note that you can use the face of the protractor shown on the left as an erasable drawing surface. • Using a scale ruler to measure the length of each route segment we find they are 74, 52 and 33 nm respectively. Checking minimum safe altitude We now have to decide the minimum altitude at which each segment can be safely flown. We will allow a minimum safety margin of about 1000 feet above the highest terrain 10 nm either side of the required track. From the chart the highest terrain for the first segment is 1036 feet, so our lowest safe altitude is 2000 feet above mean sea level. Similarly on the second and third legs the highest terrain is 1584 feet, so our lowest safe altitude will be 2500 feet on both. The cruising altitude will be determined by the wind profile at flight time and the appropriate VFR cruising level; although for best engine performance a cruise altitude, where the throttle is fully open and the engine is delivering 65%–75% power, is indicated. The preliminary flight plan We have now accumulated the non-variable part of our flight plan: Flight plan Route segment Distance Track [true] Lowest safe altitude Comms Oxford – Warraway Mountain 74 094° 2000 ML 124.9 Warraway – road junction 52 061° 2500 ML 124.9 Road junction – Tottenham 33 040° 2500 ML 123.9 Before we can proceed further we must: ascertain the weather and the wind velocities that are forecast for the period of our planned flight check for NOTAM that may affect us determine the times of first light / last light, remembering that VFR pilots should not plan to be airborne before first light plus 10 minutes nor after last light minus 10 minutes if the air temperatures are above average and/or the departure, destination and alternative airfields are elevated, calculate the density altitudes and decide whether all possible take-offs and landings can be conducted safely. First light/last light; the official times for 'first' and 'last' light are purely a mathematical calculation and do not take into account diminution of light caused by terrain shadowing, cloud overcast, haze or mist. In southern Australian winter conditions it may be advisable that lengthy VFR flights should be planned to conclude at least one hour before the official last light. 4.3.6 Obtaining weather forecasts, NOTAM, first light and last light Aeronautical briefing information — the NAIPS Internet Service Airservices Australia provides an online forecast service for 30 or so aviation forecast areas — ARFORs — shown on the PCA. First we need to locate our flight area on the PCA — outlined in green on the PCA section shown and thus located in ARFOR 22. The black grid on the PCA is the individual WAC coverage so our planned flight area (outlined in green) is more or less contained in WAC 3457 but we would certainly need to also take along WAC 3356 adjoining the northern edge of WAC 3457. Weather forecasts, NOTAMs and other pre-flight information are downloadable from Airservices Australia's [AsA] NAIPS Internet Service [NIS], 'a multi-function, computerised, aeronautical information system. It processes and stores meteorological and NOTAM information as well as enabling the provision of briefing products and services to pilots and the Australian Air Traffic Control platform'. NIS is accessed through the internet with any web browser or access may be integrated within flight planning software. The Bureau of Meteorology provides all the weather products to the NIS. The old AIS/MET service is, or will be, discontinued. You must register with AsA before you can access the NIS. You are required to create a 'user name' and a password. If you don't have an ARN or Pilot Licence Number leave that field blank, don't use your RA-Aus or other sport and recreational organisation membership number, it may conflict with someone's Aviation Reference Number. Download the NIS user documentation When registered, you can log in; enter user name and password, and then click the required link. If you choose 'Area Briefing' you can select up to 5 briefing areas by clicking on the map or by entering the required areas or sub areas (4-digit codes) in the entry boxes, place a check in the 'Head Office Notam — summary' request box, enter the validity period and then click on the 'Submit' button. Alternatively, click on the Area Directory link to select any of the area or sub areas from the the directory list. An area briefing provides area meteorological information, NOTAM and meteorological information on aerodromes, NOTAM for restricted areas within the selected area, and relevant Flight Information Region meteorological and NOTAM information. The aerodrome meteorological information is in the form of Meteorological Reports [METAR] and Aerodrome Forecasts [TAF]. We will look at the meteorological information in the flight plan preparation module for which I have downloaded an area 22 ARFOR and added some comments (this opens in a new browser window). Plain English conversions of current ARFOR, METAR and TAF for all Australian ARFOR areas are available from Ian Boag's website. However pilots must still get the NOTAM from the Airservices site. Also student pilots should be aware that the ability to decode the BOM information will be tested in some of the aviation examinations. Bear in mind that CAR 120 imposes penalties for use of forecasts that were not made with the authority of the Director of Meteorology or by a person approved for the purpose by CASA and it may be that plain English conversions are not authorised by the Director or CASA. The times of first light and last light for any Australian location are accessed from the opening page — click 'First Light-Last Light'. Current UTC date and time may be derived with the 'Time Zone Converter (Local To UTC)'. Bureau of Meteorology aviation weather services You can also download the current and forecast national weather charts (plus a great deal of other information) from the Australian Bureau of Meteorology website aviation weather services page. The colour images from the BoM's Weather Watch Radar Network indicate the intensity of atmospheric precipitation overlaid on a surface map for an area up to 512 km radius from the radar. The radar images are updated every 10 minutes or so and the latest four images can be rolled into a progressive display that gives an indication of the development and velocity of such weather phenomena. The weather radars provide the most accurate and up-to-date rain and storm information, and should always be checked prior to a flight within areas covered by the radars. Lightning trackers such as Weatherzone provide useful information on current storm location and movement. 4.3.7 Educational material available from the Civil Aviation Safety Authority website Several eLearning tutorials are accessible from the 'Online learning' page in the Education section. Each tutorial topic takes 5–10 minutes to complete and the whole tutorial can be completed at your own pace. Fuel management Class D Airspace Procedures Operations at, or in the vicinity of, non-towered aerodromes OnTrack is a series (currently 12) of online, interactive guides to VFR operations in and around controlled airspace associated with six Class D general aviation aerodromes plus six Class C CTRs. They are accessible from the 'OnTrack' page in the Education section. OnTrack features interactive maps with added visual terminal chart (VTC) information utilising video, audio, pop-up alerts and text. OnTrack is not of much interest to most RA-Aus pilots because, unlike the Visual Pilot Guides, the Class C and Class D bypass routes don't seem to be included (yet?). Five Visual Pilot Guides are accessible from the 'Visual Pilot Guides' page in the Pilot guides & information section, the last revision was 13 December 2010. These publications are pdf format guides to VFR operations near Australia's major cities. The main purpose is to facilitate route planning into or from the Class D general aviation aerodromes — which is not of much interest to most RA-Aus pilots. However the guides also help familiarise recreational aviators with the recommended VFR routes, altitudes and VHF frequencies that provide safe navigation around and under the Class C and Class D airspace steps, military control zones and restricted areas. Melbourne Basin Visual Pilot Guide 11.3 MB Sydney Basin Visual Pilot Guide 10.9 MB Jandakot Visual Pilot Guide 4.8 MB Archerfield Visual Pilot Guide 4.5 MB Parafield Visual Pilot Guide 3.9 MB CASA's Visual Flight Rules Guide (November 2011 version) is now only available in pdf format, downloadable in six sections and accessible from the Pilot guides & information section. General: the rules, licensing, pilot responsibilities, radio procedures 5 MB Pre-flight planning: meteorology, briefing, notification, information services 6 MB Operations: communications, non-controlled aerodromes, controlled airspace, sport aviation 8 MB Helicopter operations 3 MB Emergency procedures 3 MB Index 2 MB Safety-related videos may be viewed at the CASABriefing YouTube channel. Stuff you don't need to know • DR was born in the early days of oceanic sailing vessels. Every hour or two during the voyage the log (a quadrant shaped piece of wood weighted to float upright with an attached log-line knotted at intervals) was dropped over the stern of a vessel under way and the vessel's speed was reckoned from the amount of line paid out over a particular period of time. In 1637 an English mathematician and navigator, Richard Norwood, calculated that the spacing between knots should be 47.25 feet with a 28 second sand glass used as the timer. If you do the calculation, using the then estimated 6075 feet to the nautical mile, you will see that the number of knots that passed over the stern rail during the 28 second period equals the ship's speed in nautical miles per hour — hence knots. The log was presumed to be 'dead in the water'; i.e not dragged by the ship or affected by tide, drift or current. Each reading was marked on a log-slate and, during each watch, the course, speed and distance reckonings — adjusted for tide and current estimates — were entered in the logbook. (Note: some obviously non-nautical people reckon that 'dead reckoning' is a diminutive of 'deduced' reckoning, but I reckon their reckoning is wrong. According to the Oxford English Dictionary the term 'dead reckoning' first appeared in print in 1613 in a work titled 'Magnetic Bodies' written by one M. Ridley; so the term has been in use for at least four centuries. The term also appeared in Richard Norwood's work, The Seaman's Practice, published in 1637.) STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.2.1 Defining position – latitude, longitude, altitude and time Lateral dimensions In aerial navigation any point on the Earth's surface may be precisely defined in terms of a latitude and longitude graticule reference. Meridians of longitude are half 'great circles', perpendicular to the equator, that extend from pole to pole. The meridians are identified by the angle that they subtend, at the centre of the Earth, with the prime meridian. That angle is measured in degrees, minutes and seconds east or west from the prime meridian: the WGS84 International Reference Meridian — or 0° longitude or prime meridian — passes about 102 metres east of the originally defined Greenwich meridian at the Greenwich Observatory, England subsequent meridians are identified as degrees east or west around to 180° there are 60 minutes of arc in a degree and 60 seconds of arc in a minute. Parallels of latitude are 'small circles' drawn around the Earth starting from the equatorial plane, north and south of the equator and parallel with it and reducing in circumference toward the poles. For our purposes we can say that the parallels appearing on aviation charts are identified by the angle that they subtend with the equatorial plane, i.e. they are geodetic, measured in degrees, minutes and seconds and whether they lie north or south of the equator: the north pole is a point position having a latitude of 90°N the south pole is a point position having a latitude of 90°S the equator has a latitude of 0° and is a great circle, in that it is formed by a plane that passes through the Earth's centre, bisecting the Earth's sphere. One nautical mile is the length, at the Earth's mean sea level surface, of one minute of arc of a great circle. The International Nautical Mile is 1852 metres or 6076.1 feet. Consequently, one degree of latitude (measured along a meridian) has an equivalent surface distance of 60 nautical miles, and one second of latitude is about 31 metres, while 1/100th of a second is about 0.3 metres. Seconds of arc are generally not used in those aeronautical publications intended for navigation under the Visual Flight Rules; latitude and longitude is expressed in degrees plus minutes to (generally) one decimal place — about 185 metres. For example the reference point for Mount Beauty airstrip in Victoria is located at S36° 44.1' E147° 10.2'; aerodrome reference points (usually regarded as the centre of the airfield) are defined in degrees, minutes and tenths of minutes. However, when necessary, the location of a point position may be specified much more precisely; some point locations for instrument landings are required to be specified to 1/100th of a second. Some systems may use degrees only, in which case the degrees may be expressed to five decimal places, e.g. S36.73499 Incidentally, a 'knot' is a speed of one nautical mile per hour. It is logical to express 'Lat/long' coordinates with the direction from the equator/prime meridian first (e.g. S and E), then a numeral group representing the degrees followed by a group for the minutes. The symbols for degrees and minutes are omitted, e.g. S36 44.1 E147 10.2. That is the standard format for geographic locations in ERSA. However in the global navigation satellite system (GNSS), and other systems, the northern hemisphere latitude coordinates may be represented as a positive value and the southern hemisphere as a negative value, while the longitude coordinates for the western hemisphere have a negative value and those for the eastern hemisphere have a positive value, so S36 44.1 E147 10.2 is represented as −36 44.1 +147 10.2. The positive sign is usually omitted for the northerly and easterly coordinates. Effect of continental drift on precise location The Earth's latitude/longitude reference graticule is regarded as fixed relative to the Earth as a whole, but the continents are in motion. The Australian tectonic plate, for example, is moving north north-east towards the North Pacific at the rate of seven centimetres per annum*. So, during the last 14 years every fence post in Australia has moved one metre north north-east and their precise latitude and longitude reference position has changed, and will continue to change. Of course these tectonic plate movements have no discernible effect on aerial navigation but they do complicate land survey activities. In Australia, to overcome this the 1994 Geocentric Datum of Australia [GDA94] uses a reference meridian that is fixed relative to the Australian tectonic plate rather than the International Reference Meridian. The map projection for GDA94 is the Map Grid of Australia [MGA94]. *For comparison, it is estimated that the average fingernail growth is 3.5 cm per annum. The third positional dimension – altitude Contour lines and spot points on topographical maps provide an indication of terrain elevation — i.e. height above the Australian Height Datum. The aircraft's altimeter reading provides the aircraft's vertical position and thus the current height above the terrain indicated on the chart — height above ground level [AGL] or the terrain clearance — may be determined. Universal Coordinated Time Time is a most important dimension in aerial navigation; the reference time is Universal Coordinated Time (symbol UTC — a compromise between the initialisms of the preferred French and English names) rather than local times. UTC is the time at the International Reference Meridian and is an average of a large number of atomic clocks. The suffix 'Z' is used to identify dates and times as UTC, so it may be referred to as 'Zulu' time — the phonetic for 'Z'. UTC and the 24-hour clock system — rather than local time — are used throughout the aviation information, communication and meteorological services. UTC is 10 hours behind Australian Eastern Standard Time, 9.5 hours behind Australian Central Standard Time and 8 hours behind Australian Western Standard Time. Add an additional hour in a daylight saving time period. 4.2.2 Defining the shape of the Earth – geoids and ellipsoids The Australian chart elevation reference datum — the Australian height datum The Australian height datum* [AHD] is based on the average local sea level as observed throughout 1966-1968 at each of 30 tidal gauges distributed around the coastline and is the zero elevation reference for Australian aeronautical charts; i.e. the elevations shown on the charts are height above the AHD or mean sea level [msl]. * A datum is the fixed reference or starting point of a scale or measurement system e.g. an aircraft weight and balance pre-flight check. In this context the plural is datums not data. The Australian geoid The Earth's density is not uniform throughout, thus gravity and its perpendicularity — and consequently msl (or AHD) distance from the geometric centre of the Earth — varies irregularly around the surface of the globe. A geoid is a notional surface of equal potential gravity within the Earth's gravity field, that describes that irregular shape and basically follows mean sea level over the oceans and extends through the continents. The current Australian geoid is AUSGeoid09 but for aerial navigation it can be regarded as equivalent to the Australian Height Datum ±0.5 m. Check Geoscience Australia for more information about geodetic datums. The World Geodetic System 1984 A geoid is not the same as an ellipsoid (a smooth, slightly flattened sphere), which is a mathematically (rather than physically) derived representation of the Earth's underlying shape. The WGS84 ellipsoid is a mathematical representation of the Earth's underlying shape with an equatorial radius of 6 378 137.0 metres, a polar axis radius of 6 356 752.314 metres and a flattening ratio of roughly 1:300. There are many ellipsoids in use but WGS84 is of most interest to Australian aviators because it is the reference ellipsoid used by the Global Positioning System and is its basis for GPS altitude, whereas the Australian Height Datum (a 'geoid') is the basis for elevations on Australian navigation charts. GLONASS uses other ellipsoids. For aerial navigation and cartography purposes the shape of the Earth is defined by the WGS84 ellipsoid providing the standard coordinate frame for navigation/cartography systems. Some Australian charts may also show the GDA94 as the datum, which is fixed relative to the Australian tectonic plate as mentioned above, however for navigation purposes, this is compatible with WGS84. Geoid-ellipsoid separation and GPS altitude The difference in elevation of a particular point on the Earth's surface — when measured against both the ellipsoid and the geoid — can be quite considerable, as much as ±100m ; this is known as the geoid-ellipsoid separation. In Australia the degree of geoid-ellipsoid separation is quite unusual. The image below shows the substantial geoid undulation that slopes across Australia. In the south-west corner of the continent AUSGeoid09 is 33m below the WGS84 ellipsoid while at the tip of Cape York in the north-east corner it is 72m above the ellipsoid. As shown in the image the geoid and ellipsoid coincide (i.e. zero separation) on a rough line between Port Hedland and Melbourne. (Image courtesy of Geoscience Australia). The local value (known as the 'N-value') of the geoid-ellipsoid separation might be shown on aeronautical navigation charts but the values are not shown on Australian charts. The local N-value is of little significance to recreational aviators (although it should be noted that a GPS instrument may give an apparently incorrect height if the software doesn't adjust for the local N-value*) but may be of great significance to IFR pilots and designers of GPS approaches when the GNSS achieves sole-means navigation status for all flight phases. A table of the geoid-ellipsoid separation value for each cell of a roughly one nautical mile square grid covering Australia is produced by Geoscience Australia's National Geographic Information Group — previously known as AUSLIG. AUSGeoid09 provides the AHD-to-ellipsoid separations, see the AustGeoid09 on the Geoscience Australia site. *Note: some GPS receivers may store just a single N-value for each 10° latitude/longitude graticule cell. As can be seen from the image above some 10 x 10 degree cells have a 40-50m variation diagonally across the cell. If the N-value is not used or just approximated, the calculated GPS altitude may be incorrect. 4.2.3 Aeronautical charts Chart system basics A chart system is built on three basics that must be defined for use: the projection employed — generally the 'Lambert conformal conic projection' for air navigation. the coordinate system — latitude and longitude for air navigation. the geodetic datum — WGS84 [or GDA94] is the standard horizontal (area) datum for most Australian aeronautical charts and the Australian Height Datum is the vertical datum. Note: when using a GPS receiver ensure that these three formats have been selected correctly, particularly the WGS84 datum. A map intended for aerial or marine navigation is usually referred to as a 'chart'. The chart graticule is latitude and longitude, with the meridians more or less vertical on the sheet but converging slightly. As the Earth is ellipsoid, there has to be a technique to map the image of the surface of the three-dimensional ellipsoid onto a flat two-dimensional chart without overly distorting the represented areas. The most suitable projection technique for world aeronautical charts is the 'Lambert conformal conic projection'. Although this projection distorts areas a little, distances anywhere on the chart have the same scale. The great circle arc* — the shortest distance between two points on the surface of a sphere — can be represented reasonably accurately by the flight planner drawing a straight line between two points on the chart. However you will note that the angle at which that straight line crosses each meridian changes because of the convergence of the meridians. *Note: the shortest distance between, say, Sydney and Perth, is a straight line (a tunnel) joining those cities and passing through the Earth. The great circle route follows that 'tunnel' on the surface. The Lambert chart legend will indicate the latitudes of two 'standard parallels'. There is no scale distortion at these parallels, however scale distortion increases with distance from a standard parallel. For an explanation of standard parallels see www.icsm.gov.au/mapping/about_projections.html and look for the heading 'Multiple standard parallels or central meridians'. Those meridians of longitude shown on Lambert conformal aeronautical charts are straight lines, that converge towards the poles*. On a southern hemisphere chart the meridian spacing between the meridian lines at the bottom of the sheet is a little less than that at the top — about 5 mm on an Australian 1:1 000 000 World Aeronautical Chart. A central meridian drawn on each chart is vertical and the others converge towards it. The parallels of latitude as shown on the chart are arcs of circles and cross all the meridians at right angles because of the slant of the meridians. If a straight line is drawn diagonally across the chart, the angle that this great circle route subtends with each meridian varies slightly across the chart. Aircraft flying very long legs would alter their heading slightly every 500 nm or so to maintain the great circle route and thus the shortest distance. *Note: that convergence of the meridians is why the 'grid' on such charts is called a 'graticule'; the meridians and parallels do not form true rectangles, i.e. a 'grid'. If you joined a number of WACs together by matching parallels and the edge meridians the maps would form an arc. On Mercator (a 16th century Flemish geographer) cylindrical projection charts, straight line plots are 'rhumb lines' and great circle plots are curved. A rhumb line is a line drawn so that it crosses the meridians of the Mercator projection at a constant angle, but it is not the shortest distance between two points; an aircraft flying a constant track heading would be following a rhumb line plot. The concept of choice between a great circle route or rhumb line route is interesting but inconsequential to a light aircraft navigator because a constant track heading (i.e. a rhumb line track) is usually flown for each leg; except, perhaps, if planning a direct route from Australia to New Zealand. The scales used for aeronautical charts are the representative fractions 1:1 000 000, 1:500 000 and 1:250 000. The latter scale means that an actual distance of 2.5 km (250 000 centimetres) is represented by one centimetre on the chart. The 1:1 000 000 scale is a small-scale chart; i.e. it covers a large area but with minimum detail, one centimetre represents 10 km. The 1:500 000 and 1:250 000 are larger-scale charts that cover progressively smaller areas but with increasing detail. The Australian Intergovernmental Committee on Surveying and Mapping's Fundamentals of Mapping is well worth visiting. Recommended VFR charts The paper charts recommended for sport and recreational aviation VFR flight planning, in-flight navigation and sourcing VHF radiocommunications data are: Planning Chart Australia: the PCA is a single sheet showing the coverage of the WACs (below), the meteorological area forecast [ARFOR] boundaries, the estimated FIS VHF coverage from both 5000 feet amsl and 10 000 feet (but not the frequencies), and the areas without FIS VHF coverage. The FIS HF communication frequencies are shown. The spot location of about 700 named airfields is indicated. PCA is designed to assist in initial VFR flight planning and it is amended semi-annually. It is of rather limited use in initial planning of flights below 5000 feet (i.e. most ultralight flights) in eastern Australia because straight-line tracks between departure point and destination may be precluded because of the topography, and there are no indications of such on the PCA. But it is generally okay for use west of the Dividing Range. Also it is the only chart that indicates FIS VHF coverage; essential knowledge if a flight is being planned into the less accessible areas of Australia. World Aeronautical Charts: the 43 Australian WACs are small scale (1:1 000 000 or 1mm=1km), derived from aerial photography, and designed for pre-flight planning and pilotage. They are part of an ICAO international series. They do not indicate CTR or PRD, nor is there any FIA, radiocommunications or radionavigation information. As the reissue frequency is 2–4 years (i.e. 50% of the 43 maps are supposed to be re-issued every two years) the base can be slightly out of date, particularly in regard to the infrastructure. Amendment lists for each edition are published in AIP SUP but these amendments generally relate to location of airstrips and special activities rather than topography or infrastructure. Each WAC generally covers 6° of longitude and 4° of latitude. Sheet dimensions are about 70 × 60 cm and the scale is such that a real distance of one nautical mile is represented by less than 2 mm on the chart; thus WACs are really not suited to low-altitude navigation in slow aircraft, but it is wise to always have the latest edition of the WAC/WACs — relevant to the journey — in the cockpit. Visual Navigation Charts: the VNCs are a larger scale at 1:500 000 and show airspace information and FIS detail laid over the topographic base. All VNCs are reissued at six-monthly intervals but the base topographic detail may not be up to date. They are far superior to the WACs for both flight planning and pilotage. VNC sheet dimensions are about 100 × 60 cm and contain the following airspace detail: CTR, CTA dimensions and lower levels Flight Information Area and Surveillance Information Service boundaries where available Flight Information Service and Surveillance Information Service frequencies and providers communication and navigation aid frequencies for licensed airfields PRD and designated & remote areas. There are only 15 VNCs, those available covering the more populous areas of Australia — Tasmania to North Queensland, plus areas around Perth, Adelaide, Darwin and Tindal. Visual Terminal Charts: the 25 or so VTCs provide both aeronautical and topographic information around major airports at a scale of 1:250 000. They are essential for VFR operations in the vicinity of such airports to avoid violating controlled airspace. In some cases, these charts show the details of tracks to be flown and significant landmarks to be used by pilots of VFR aircraft to avoid inadvertent entry into controlled airspace. All VTCs are amended and reissued every 6 months. The charts are based on the NATMAP 250K series maps and use the Universal Transverse Mercator [UTM] projection but with a latitude/longitude graticule rather than the normal UTM grid; their dimensions are around 90 × 50 cm and show the following details: PRD areas CTR and associated CTA dimensions including the lower levels of the CTA steps surrounding the airport, lanes of entry, ATC check points Surveillance Information Service frequencies where available communication and navigation aid frequencies for licensed airfields VFR approach points. En Route Chart (low level): the ERC-L series is drawn to various scales to accommodate significant air traffic route areas and shows controlled airspace, PRD areas, air routes and segment distances, ATS and radio-navigation services, ATS frequencies and location, plus communication and navigation aid frequencies for licensed airfields — but no topography. It also indicates those airfields where VHF radio contact with FIS is possible from the ground. The FIS area boundaries are shown together with an information box showing the provider of the flight information service (e.g. Brisbane Centre), the frequency and the location of the area transceiver. The series of eight sheets cover Australia and are intended primarily for IFR flights conducted below 20 000 feet. The multitude of air routes that radiate from major cities make the charts difficult to read but they are the only chart series that show all the FIS frequencies, PRD areas and give indications of sports aviation activities — thus they are an essential document for VFR navigation. Each route segment is a great circle route with the magnetic track angles measured at the end points rather than the middle of the segment, which is why there is an apparent discrepancy in the reciprocal track angles. Reissue frequency is twice per year. PCA, WAC, VNC, VTC and ERC-L can be purchased from the Airservices Australia online store. You can see the coverage for each sheet in each series by clicking 'Coverage Map' on their 'Aviation charts' page. These charts can also be ordered from pilot supply shops. Possibly the TPCs may be purchased from the National Mapping Division of Geoscience Australia. Satellite and aerial images of the Earth's surface are also available via the Google Earth and Google Map geobrowsers and provide help in flight planning; for example, the ability to locate an unlisted airstrip and establish the exact lat/long coordinates for entry into a GPS. Carriage of flight documentation AIP ENR 1.10 para 5.1 states: 'Pilots are required to carry, and have readily accessible in the aircraft, the latest editions of the aeronautical maps, charts and other aeronautical information and instructions, published: a. in AIP, or b. by an organisation approved by CASA, that are applicable to the route to be flown, and any alternative route that may be flown, on that flight.' (The AIP entry is an extract from CAR 233 'Responsibility of pilot in command before flight') Digitised aeronautical charts The WAC, VNC, VTC and ERC-L charts, and others for flight under the instrument flight rules [IFR] , are also available in digitised format — raster or vector images — for use in tablet computers with flight planning software and for inflight use with portable electronic devices with moving map software. They have the same reissue frequency as the paper charts. This is discussed in the 'Electronic planning and electronic flight bag' module. 4.2.4 Map topography Aircraft operating under the VFR must navigate by visual reference to the ground. The lower the level at which a flight is planned, the more important it is that the pilot is able to visualise a three-dimensional image of the terrain from the graphical details presented by the two-dimensional topographic chart — by the usage of colour, symbols and lettering. To assist this visualisation, WACs and VNCs display tinted topographic contours signifying surface areas between the 660 feet (200 m) and 1639 feet elevations, 1640+ feet (500 m), 3280+ feet (1000 m), 4920+ feet (1500 m) and 6560+ feet (2000 m) levels. The shape of the contours and the width between them indicates the form of the land and the gradient. The closer the contour lines (i.e. the narrower the colour bands) are to each other, the steeper the gradient. Also the WAC utilises relief shading of elevated ranges and ridges so that they are more evident. In addition, spot elevations are shown and the highest spot elevation within each chart graticule is recorded in a bolder lettering than other spot elevations. The graticule on the WACs and VNCs is spaced at 30 minutes of latitude and 30 minutes of longitude: 30 nm in latitude and, for much of Australia, around 24 nm in longitude. The contours on VTCs are at 500+, 1000+, 2000+, 3000+, 4000+ and 5000+ feet amsl, but in addition all areas are shaded purple where there is less than 500 feet of clearance between the terrain and the lower limit of the overlying controlled airspace. Like WAC and VNC, the highest spot elevation within each chart graticule is shown in a bolder type than other spot elevations. The graticule is spaced at 10 minutes of latitude and 10 minutes of longitude: 10 nm in latitude and around 8 nm in longitude. The VTCs generally cover an area within a 40–50 nm radius from the major airport and are the essential chart for visual navigation within that area. Vegetation is usually not shown on WACs, nor are many structures except for towers and similar obstructions to low-flying aircraft; although grain silos — which are an excellent navigation aid usually associated with a railroad — are shown. Railroads, power transmission lines and some roads are depicted. 4.2.5 Defining direction — the aircraft direct reading magnetic compass Sport and recreational navigation under the VFR is basically azimuth and distance and is also short-range i.e. each leg is usually less than 500 nm or so. Directions in azimuth are usually expressed as the angular distance from the north pole — true north — in whole degrees from 0° at north clockwise to 360°; i.e. north is both 0° and 360° (though is usually expressed as 360°). For example, the direction due east from any particular location is 090°. These directions may be described as bearings, headings, courses or tracks depending on the application. Direction is usually paired with distance expressed in nautical miles, thus the bearing and distance of a location 55 nm due east would be expressed as bearing 090°/55. However, the prime navigational direction instrument — the magnetic compass — aligns itself with the north magnetic pole and, in Australia, the variation between the direction to true north and that to magnetic north can be as much as 13 , so there is a need to define directions in terms of 'degrees true" or 'degrees magnetic'. Civil Aviation Order 20-18 specifies just four mandatory flight and navigational instruments for flights under the day Visual Flight Rules. These basic instruments are: an airspeed indicating system an altimeter, with a readily adjustable pressure datum setting scale graduated in millibars an accurate timepiece indicating the time in hours, minutes and seconds, which may be carried on the person of the pilot and a direct reading magnetic compass. If the aircraft is a Light Sport Aircraft for which a current special certificate of airworthiness or an experimental certificate has been issued it need not carry the individual instruments as defined above, if equipment is carried that provides a pilot with the same information, i.e. an electronic flight display. Magnetic variation The simple direct reading compass is essentially a bar magnet freely suspended in a lubricating fluid designed to damp out oscillations, vibrations and swings caused by aircraft accelerations. The bar magnet, which may be a needle or part of a circular compass card, aligns itself with the Earth's local magnetic lines of force with the north-seeking end pointing roughly north. The Earth's magnetic field is systematically surveyed so that the difference between the direction at which a compass points — magnetic north— and the direction of true north is measured. That difference is called variation, or declination if you are of a scientific bent, and is expressed in degrees of arc east or west of true north. The magnetic lines of force at any location may also be substantially varied by local magnetic anomalies — substantial iron ore deposits for example. Lines on a chart joining locations with equal magnetic variation are isogonals, or isogonic lines, and are shown on WACs and VNCs as dashed purple lines at half-degree intervals. The local variation may also be shown numerically on some charts. The isogonals on Australian charts vary from 3° west in the south-west corner of the continent to 13° east on the eastern coast. This means that if you want to fly from A to B, the direction ascertained from the chart will be relative to true north — the true course — and let's say it is due west, 270°. If you then set 270° on the aircraft compass and fly that heading then your track over the ground will not be due west but will vary according to the variation. Let's say the variation is 10° east then the true course you are flying will be 280°. This small complication requires that when you have finally calculated the true course you have to fly to get from A to B, after allowing for the effects of wind, then you need to convert it to a magnetic heading. The conversion rule used for at least the past 70 years is: "Variation east, magnetic heading least; variation west, magnetic heading best". So if the local variation is 12° east the magnetic heading will be the true course minus 12°; e.g. true course 010°, magnetic heading 358°. If the variation is 2° west the magnetic heading will be the true course plus 2°; e.g. true course 010°, magnetic heading 12°. For all wind velocities, given in meteorological forecasts and actuals, the directions are relative to true north, except if you happen to hear a broadcast from a CTR tower controller (or an Automatic Terminal Information System [ATIS] broadcast) who provides the wind direction as magnetic, because the airfield runway numbers are relative to magnetic north. The air route directions shown on ERC-L are also relative to magnetic north. Compass deviation Aircraft compasses are also deflected by magnetic fields within the aircraft, some related to ferrous engine/structural metals, others related to electrical currents. These aircraft magnetic fields produce heading errors — compass deviation — which vary according to the aircraft course, either reducing or increasing the Earth's magnetic field. These errors can be quite significant, 30° or more, and any magnetic field within about one metre of the compass may have a discernible effect. Mobile telephones in the cockpit may also affect the compass. Compass error is the combination of variation and deviation adjustment necessary to determine the compass heading that will provide the true course. A bar magnet aircraft compass will have screw-adjustable compensating magnets to negate or at least reduce the effect of these magnetic fields. The compass and aircraft must be 'swung' to make these adjustments, and the residual deviation errors noted on a compass correction card displayed in the cockpit. Residual deviation errors should not exceed 10° at any compass point. The procedure for 'swinging the compass' is time-consuming and difficult but necessary. We will go further into compass deviation in the 'En route adjustments' module. Airfield runway numbers are stated as their magnetic heading rounded off to the (supposedly) nearest 10°; thus an east-west runway will be numbered 09/27. The ERSA entry in the "Physical characteristics" section for the airfield usually shows the actual magnetic heading following the runway numbers, but only for one direction. For example at Dubbo aerodrome '05/23 043' indicates the actual magnetic heading for runway 05 is 043° magnetic, and consequently 223° for runway 23. Thus, when stationary and accurately lined up for take-off on such a runway, you can measure deviation on that heading; but make sure the compass has stopped moving. Flying to a few airfields and checking deviation at various runway headings is one way of producing a compass correction card. Always make sure the compass fluid level is okay. A vacuum chamber for de-aerating the compass fluid must be used in the re-filling process — using the proper fluid, not alcohol. Bar magnet compasses are also affected by vibrations, aircraft accelerations and inertia when turning; thus they tend to be shifting constantly. Compass acceleration errors are most apparent when the aircraft is on an east/west heading and least apparent when on a north/south heading. The turning errors require the pilot to make an undershoot/overshoot adjustment when changing heading. To overcome these errors, normally the magnetic compass is accompanied by a gyroscopic instrument that indicates the direction in which the aircraft is heading, without being subject to external forces. This electrically or suction-operated directional gyro [DG] or direction indicator [DI] is initially aligned with the compass before take-off and needs to be realigned occasionally during flight; however, few ultralights are equipped with DGs. Electronic flight information systems [EFIS or 'glass cockpits'] are now becoming much cheaper and thus a reasonable proposition for amateur-built light aircraft. These systems use solid-state electronic componentry plus software to present a cockpit display incorporating the functions of most single flight instruments. In such systems magnetic field strength sensors (magnetometers) are used to provide a three-dimensional magnetic compass that displays magnetic heading without acceleration, attitude or turning errors; thus it also incorporates the DG facility. The simple direct reading magnetic compass must still be part of the aircraft equipment. Things that are handy to know • There are other maps such as the Australian NATMAP 250K series. This is intended mainly for surface use, with the GDA 94 datum and using the Map Grid of Australia projection (MGA 94) which conforms with the Universal Transverse Mercator (UTM) projection with usually the metric UTM Eastings and Northings grid — rather than a latitude/longitude graticule. This coordinate system is more complex than latitude/longitude — the Earth's surface is divided longitudinally into 60 six-degree numbered zones and Eastings are measured in metres (to 3 decimal places) from the central meridian of each zone. Northings start from zero at the equator in the northern hemisphere but, for the southern hemisphere, start from a 10 000 000m base Northing at the equator. See en.wikipedia.org/wiki/UTM_coordinate_system. Fortunately the digitised NATMAP 250K series are also available with a latitude/longitude graticule, so these larger-scale (1:250 000) maps could be used for the limited leg distance of recreational aircraft navigation, particularly with GPS. Each map covers an area of 1.5° longitude and 1° latitude. VTCs, being based on the NATMAP 250K, use the Transverse Mercator projection with a lat/long graticule. Some UTM maps may show the lat/long graticule* in one colour with the UTM grid* in another. *Note: 'grids' are rectangular in shape; the 'graticule' is not — the meridian lines converge poleward. The digitised NATMAP 250K series may be purchased from Geoscience Australia. The 513 maps of the NATMAP 250K series are available on DVD for about $100 which is less than 3% of the cost of the paper series and well worth having as home reference material — even if you don't use them for aeronautical navigation. They are in ECW format and software is supplied for viewing and for export to geoTIFF, TIFF, JPEG, PNG, bitmap or OziExplorer format. Image resolution is 200 dpi and the pixel size is around 30 metres with a positional accuracy of 127 metres. The 'Map Viewer' software supplied is currently (2012) confined to Windows operating systems. View 'About NATMAP Digital Maps 2008'. Stuff you don't need to know • Maps that lack contours, like street maps, are planimetric; i.e. flat. • 'Large scale' maps are those with a scale of 1:70 000 or less. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
4.1.1 Controlled airspace 'Controlled airspace' is airspace of defined dimensions within which air traffic control service is provided in accordance with the airspace classification. There are two types of controlled airspace: A Control Area [CTA] is controlled airspace that extends from a specified limit above the surface (e.g. 8500 feet amsl) to some upper level (e.g. 18 000 feet amsl — or FL180). A Control Zone [CTR] is controlled airspace, surrounding a civil or military aerodrome (with a manned Air Traffic Control tower), that extends from ground level and is stepped up to the lower limit of the overlying CTA. The steps provide the airspace for the airport approach and departure paths. Please note: the CTA abbreviation is commonly used when referring to the generic controlled airspace (i.e. CTA plus CTR) rather than just Control Area(s). There is no abbreviation listed in the Australian Aeronautical Information Publication [AIP] for the generic 'controlled airspace'. OCTA is the AIP abbreviation for the term 'outside control area' and OCTR is the abbreviation for the term 'outside control zone'. However the OCTA term is commonly used by pilots and Air Traffic Services personnel when referring to operations outside controlled airspace. Airspace classification Four of the International Civil Aviation Organization [ICAO] controlled airspace classes are currently used in Australia; A,C, D and E. Recreational Pilot Certificate holders flying an aircraft operating under the CAO 95.55, CAO 95.32, CAO 95.12 and CAO 95.10 exemption orders may only enter and fly in Class C and D airspace if they meet specified requirements; see 'Operating airspace allowed, pilot qualifications and equipment required'. For flight in Class A airspace, a recreational pilot must seek and receive written permission from the Civil Aviation Safety Authority for the flight. It is solely the pilot's responsibility to operate legally; even obtaining an air traffic controller's permission to enter controlled airspace does not make the flight legal, nor does it absolve the pilot if something goes wrong. It is always the VFR pilot's responsibility to see and avoid other traffic. In Australia, Class A is high-level en route airspace, and Class C surrounds major city airports and military airfields starting at ground level and stepped up into mid-level Class C or the high-level Class A airspace. Also, when active, military restricted areas are Class C controlled airspace. The control area — generally within secondary surveillance radar [SSR] coverage — between Sydney and Melbourne is designated Class E between 8500 feet amsl and FL125, Class C between FL125 and FL180, and Class A above FL180. The control area — generally within SSR coverage — between Sydney and Cairns is designated Class E between 8500 feet and FL180, and Class A above FL180. (For explanation of the 'CTAF' and '126.7' aerodrome notations appearing in the diagrams see Operations at non-controlled aerodromes and airstrips in Class G.) CTRs at smaller regional airports (which lack primary radar coverage) are Class D airspace; these are only active as such when the control tower at that CTR is manned. They revert to Class G CTAFs at the times when the tower is not manned. The CTR starts at the surface and is stepped up into the Class C approach/departure areas for that or neighbouring towered aerodromes. The upper boundary of Class D is generally between 2500 feet and 4500 feet amsl. Transponders are not required in Class D CTRs. In Australia, there are six major city aerodromes (Jandakot, Parafield, Moorabbin, Camden, Bankstown and Archerfield) dedicated to general aviation purposes (i.e. no regular public transport [RPT] operations). They were formerly designated as General Aviation Aerodrome Procedure [GAAP] control zones but, in accordance with the national airspace policy, Airservices Australia implemented revised Class D air traffic procedures at those aerodromes on 3 June 2010. Thus, the 'GAAP' designation disappeared from Australian aviation regulations and airspace terms now generally conform with the ICAO standards. Class E airspace Australian Class E is mid-level en route airspace, the general base of which is at 8500 feet amsl within SSR coverage and at FL180 in the remaining continental area. However, there are three Class E corridors with the base at FL125 and extending up to the overlying Class A. All aircraft require a clearance from ATC before entering Class A, B and C airspace, and a transponder must be operated. VHF radio-equipped VFR aircraft (including RA-Aus/HGFA/ASRA aircraft) may operate in Class E airspace without an Air Traffic clearance, but the pilot must: maintain a listening watch on an appropriate frequency fly VFR cruising altitudes below 10 000 feet (or cruising flight levels above the transition layer) activate anti-collision lights and the aircraft must be equipped with a properly functioning Mode A/C or S transponder with code 1200 selected and operating. In addition, the aircraft altimeter should be accurate to within 100 feet. There is a general transponder exemption (AIP GEN 1.5 para 6.1.2) for aircraft not equipped with an engine-driven electrical system capable of continuously powering a transponder. Some specific transponder exemption conditions may be allowed subject to prior agreement with ATC; see AIP GEN 1.5 para 6.2.2. RA-Aus/HGFA/ASRA aircraft operating in Class E must be equipped with a serviceable VHF communications system. The AIP Book is perhaps at variance with the CARs and CAOs, so it is not absolutely clear whether a hand-held unit is acceptable in controlled airspace. Hand-held transceivers approved by the Australian Communications and Media Authority are acceptable for use in RA-Aus/HGFA/ASRA registered aircraft operating in Class G airspace. See AIP GEN section 1.5 paragraphs 1.1, 1.2 and 1.5. The pinkish tinge covering most of the continent in the image indicates the general FL180 Class E base, the tan colour indicates the areas within radar coverage where the Class E base is either at 8500 feet or FL125, and the green colour indicates where the Class E does not exist (i.e. Class C CTRs extend up to the base of Class A airspace) or Class C extends to the upper level of a Class D CTR. In Class E, all flights operating under the instrument flight rules [IFR] are provided with an air traffic control separation service; hence, it is controlled airspace even though VFR flights within the same airspace are not provided with a traffic separation service — though they may be provided with a Surveillance Information Service [SIS] on request if the controllers have the capacity to do so. However, "due to the nature and type of radar coverage (in Class E), not all aircraft will be observed on radar". An aircraft operating under the VFR that encounters instrument meteorological conditions must then obtain a clearance to continue the flight under the IFR. 4.1.2 Airservices Australia and the Civil Aviation Safety Authority Airservices Australia [AsA] AsA is a government-owned corporation providing air traffic management and control together with related services within the Australian aviation industry. This includes airspace management, aeronautical information, communications, radio navigation aids plus airport rescue and fire fighting services. Air traffic services [ATS] are provided by the air traffic controllers of Airservices Australia [AsA], using their HF and VHF radiocommunications networks or their data uplink facilities. There are two main ATS centres; Brisbane Centre [BN CEN] holds international responsibility for a flight information region [Brisbane FIR] covering the northern part of Australia plus the oceanic airspace to the east while Melbourne Centre [ML CEN] is responsible for the flight information region [Melbourne FIR] covering the southern part of Australia plus Southern Ocean and Indian Ocean airspace. Those two FIRs make up the Australian FIR covering 50 million square kilometres — about 10% of the Earth's surface. BN CEN and ML CEN air traffic controllers and area radar controllers provide the area control service for the en route traffic. Approach controllers and approach radar controllers — plus aerodrome controllers associated with the 28 civilian international, domestic and regional towered airports — manage the terminal area traffic. There are also two 'joint-user' airport towers (Darwin and Townsville) manned by RAAF personnel. In Australia, the assistance provided to sport and recreational aviation by ATS consists of a flight information service [FIS] — both preflight and in-flight — for traffic in the Class G and Class E airspace and an in-flight emergency response service including a search and rescue authority alerting service. The in-flight FIS consists of an ATC initiated FIS, automated broadcast services together with an ATC 'on-request' assistance service with the generic call-sign 'Flightwatch'. Standard information delivered by Flightwatch includes aerodrome weather and NOTAM. A Surveillance Information Service [SIS] (previously known as the Radar/ADS-B Information Service [RIS]) including 'ATC flight following' may be available in any Class G and Class E airspace that is within the ATS radar surveillance coverage near the major cities, but availability is dependent on the controller's work load. If available SIS 'flight following' is of great value to transponder-equipped recreational aircraft threading their way around a control zone — thereby avoiding any unintentional violation of controlled airspace. Navigation assistance, position information and traffic information services may be provided. ATC also provides the SARWATCH search and rescue alerting service; primarily for aircraft operating under the instrument flight rules but also automatically provided to recreational aircraft in two-way communication with ATC and operating under an airways clearance. The Airservices Australia communications network delivers air-ground-air communications to individual ATS operating positions using around 600 radio transceivers located at more than 150 sites across Australia. Remote radar, VHF and HF transceivers are linked to ML CEN and BN CEN by about 110 satellite ground stations plus microwave radio bearer links and fibre-optic link facilities. The FIR work load from 'en route' aircraft is apportioned among the FIR personnel by dividing the region into multiple 'Flight Information Areas [FIA], each FIA using a particular VHF frequency. Each air traffic controller may monitor several frequencies. Communications with aircraft in the vicinity of the major airports may be handled by operators in terminal control units such as 'Sydney approach'. The Civil Aviation Safety Authority [CASA] CASA is an independent statutory authority whose mission is to 'enhance and promote aviation safety through effective regulation and by encouraging the wider aviation community to embrace and deliver higher standards of safety'. CASA is responsible for safety regulations, licensing of pilots and aviation engineers, certification of aircraft and aircraft operators, and certification and registration of aerodromes. 4.1.3 Class G non-controlled airspace In Australia, all airspace that is not promulgated as class A, C, D, E or restricted is Class G, and is open for flight up to, but not including, 10 000 feet amsl to all holders of a valid Pilot Certificate flying any RA-Aus/HGFA/ASRA registered aircraft. Flight at or above 5000 feet requires VHF radio. Class G extends over most of Australia from surface level to the overlying CTA base at 8500 feet amsl, FL125 or FL180. The total volume of Class G airspace included between the average land mass elevation of 1100 feet and 10 000 feet is some 20 million cubic kilometres. All sport and recreational powered aircraft operating at or above 10000 feet amsl, whether in controlled airspace or Class G airspace, must have written CASA approval for the flight and must be equipped with an operating Mode A/C or S transponder. Also Australian Civil Aviation Order part 20.4 specifies use of supplemental oxygen systems. Operations at non-controlled aerodromes and landing areas in Class G Most of the roughly 2000 (excluding 'home' strips) Australian aerodromes and landing areas are in Class G airspace and have no air traffic control service; i.e. they are 'non-controlled'. To maintain safe separation in the vicinity of such airfields, pilots are required to exercise 'see and avoid' techniques supplemented by VHF monitoring and particular radiotelephony communications and procedures in Class G airspace. These are designed to maintain traffic awareness and to self-administer circuit priorities, where appropriate, in the vicinity of the airfields. Discrete radio frequencies known as common traffic advisory frequencies [CTAFs] are generally assigned for use in those circumstances — that class of airfields then tend to be known as 'CTAFs'. Carriage and use of VHF radio transceivers is generally not mandatory — but highly recommended. However, there are about 300 certified, registered or military non-controlled aerodromes — usually those which have regular or perhaps occasional RPT movements — where the carriage and use of VHF radio, confirmed to be functioning on the CTAF, is mandatory for all aircraft (including recreational aircraft) operating at that aerodrome. That type of mandatory radio location was previously known as 'CTAF (R)' but the CTAF (R) term disappeared from the regulations 3 June 2010. The VHF radio communications recommended when operating in the vicinity of non-controlled aerodromes are defined in the AIP Book section ENR 1.1 sections 40–50 "Operations in Class G airspace". All radio-equipped (whether fixed installation or hand-held) aircraft, including recreational aircraft, should make the one mandatory broadcast plus the recommended broadcasts, when appropriate, on the CTAF. Some non-controlled aerodromes may have a private ground-based Unicom communications operator. 4.1.4 Restricted, danger and aerial sporting areas Special use airspace, extending to varying heights, is defined on the charts used for air navigation. For safety reasons, flight into those defined special use areas may be prohibited (P), restricted (R) or marked 'danger' (D); the latter as a warning to take extra care if entering the area. Flight within a prohibited area is forbidden at all times but usually (except for Pine Gap in central Australia) prohibited areas are of a temporary nature. Most of the restricted areas are used by the defence forces for exercises such as operational flying training or live weapons firing including air-to-air, air-to-ground and ground-to-air. Restricted areas extend from a lower level (often the surface) to a nominated upper level. Flight within that airspace may be restricted at all times, or may be allowed at times when the restricted area is not active. Flight within an activated area without clearance may be extremely hazardous; even the declaration of an emergency will not guarantee safe passage although, in a declared emergency, ATS will make every effort to obtain approval to transit a restricted area, irrespective of its status. Read the article 'Military restricted areas' in Flight Safety Australia. The air navigation charts show a reference number that refers to a detail entry in the Airservices publication 'En Route Supplement - Australia' [ERSA PRD]. Details of the activation of restricted areas are promulgated by Airservices Australia in the form of NOTAM. When activated a military restricted area usually becomes Class C airspace so is automatically denied to recreational aircraft unless the pilot and aircraft meet all the conditions specified in CAO 95.55 paragraph 7.3, CAO 95.32 paragraph 7.3, CAO 95.12 paragraph 6.3, CAO 95.12.1 paragraph 7.4 or CAO 95.10 paragraph 6.4; that is, the pilot must be authorised to operate in Class C airspace. Also the aircraft must be fitted with an operating transponder if the controlled airspace in which the aeroplane is operating requires a transponder to be fitted. All restricted areas are allocated a 'R(estricted) A(rea) conditional status' — RA1, RA2 or RA3 which appears in ERSA to give pilots an indication of the likelihood of receiving an ATS clearance to fly through a restricted area — if there is an Air Traffic Service associated with that area and contactable via VHF radio. The status conditions are for flight planning and pilots without a submitted flight plan may request a clearance in RA1 and RA2 at any time. Conditional status can change from day-to-day, and changed status will be notified on the activation NOTAM. RA1 – pilots may flight plan through the restricted area and under normal circumstances expect a clearance from ATC RA2 – pilots must not flight plan through the restricted area unless on a route specified in ERSA GEN FPR or under agreement with the Department of Defence, however a clearance from ATC is not assured. Other tracking may be offered through the restricted area on a tactical basis RA3 – pilots must not flight plan through the restricted area and clearances will not be available. Please note. CAO 95.55 section 7.1 also states: (e) the aeroplane must not be flown inside an area designated as an area where the operation of an aeroplane, to which this Order applies, would constitute a hazard to other aircraft. CAOs 95.10, 95.12, 95.32 and the other recreational aviation part 95 CAOs contain similar rules. Danger or alert areas usually relate to mining or quarrying sites, and to special aviation activities such as fixed training areas or aerobatic areas; it may be prudent to avoid such areas, but there is no restriction on entry. Other special use areas, for example those for hang-gliding or radio-controlled model aircraft flying, are also symbolically marked on aeronautical charts as a warning device, but there are no details available for these in any publication. Similarly, mines and quarries marked on charts, but not within a danger area, should only be overflown at a safe height to avoid blasting debris. Designated Remote Areas are also shown on Australian charts. No VFR aircraft should attempt flight within those areas unless equipped with adequate survival gear and some form of satellite compatible radio distress beacon. The main designated remote area roughly covers all the mainland north of lines between Kalgoorlie and Bourke and between Mount Isa and Townsville. There are two other designated remote areas, the mountainous regions in the south-east corner of the mainland and in western Tasmania. Aerial sporting activities. Aircraft, who are unaware of (or who don't take steps to avoid) gliding and hang-gliding operations or parachuting operations at drop zones, present a danger to the aerial sporting participants. The rules for gliding, parachuting and ballooning are contained in AIP ENR 5.5. 4.1.5 Aerodromes and aircraft landing areas The ground sites used for powered aircraft operations range from the extremely costly international airports to the basic natural surface, private airstrip or paddock. The larger airfields are known as 'aerodromes' [ADs], the smaller are officially identified as 'aircraft landing areas' [ALAs]. The ALA term includes 'aeroplane landing areas' [also ALA] and 'helicopter landing sites' [HLS] and also seaplane water alighting areas. So, the ALA initialism can mean both 'aircraft' and 'aeroplane' landing areas. Prior to 1992 (when the CASA authorisation under CAR 89 was removed) the ALA initialism described a CASA authorised landing area for aircraft under 5700 kg engaged in private, aerial work and charter operations. Since 1992 pilots are required to determine suitable places for their operations, but unfortunately the old, but now erroneous, 'authorised landing area' term still persists in the descriptive material of some airfields. So, do not think that CASA has determined that something described as an "authorised landing area" is a suitable place for operating your aircraft. Civil aviation regulation 92 deals with the use of ADs and ALAs and states: A person must not land an aircraft on, or engage in conduct that causes an aircraft to take off from, a place that ... is suitable for use as an aerodrome [or ALA] for the purposes of the landing and taking-off of aircraft and, having regard to all the circumstances of the proposed landing or take-off (including the prevailing weather conditions), the aircraft can land at, or take-off from, the place in safety. See the CASA advisory circular 'Guidelines for aeroplane landing areas'. The Civil Aviation Safety Authority recommends that aircraft with a MTOW greater than 5700 kg use ADs only. The aerodromes approved by CASA as being suitable and available for RPT operations are classified as 'certified' [CERT] or 'registered' [REG] depending on the CASA standard achieved. Only 26 of the civilian certified ADs have control towers manned by Airservices Australia personnel, the remainder (other than military [MIL] ADs) are classified as 'non-controlled'. There are about 300 CERT and REG aerodromes across Australia, ranging from the international airports to small town airfields. I have compiled a listing in text file format of CASR Part 139 Manual of Standards certified aerodromes [184] and registered aerodromes [120] but it may not reflect current status. Only 300 or so of the uncertified and unregistered [UNCR] aeroplane landing areas [ALAs] appear in ERSA but that entry does not signify that such ALAs are superior to those many ALAs lacking an ERSA entry. 4.1.6 AIP Book, ERSA and NOTAM Airservices Australia publishes online versions of the AIP Book, SUPS, AICs and ERSA at www.airservicesaustralia.com/publications/aip.asp (click the 'I agree' button to gain entry). To find a particular section of AIP or ERSA you have to click through a number of index pages. The section/subsection/paragraph numbering system was designed for a readily amendable looseleaf print document, so you may find it a little confusing as an online document. AIP Book The ICAO requires that the Aeronautical Information Service [AIS] of each member nation publish a standardised 'Aeronautical Information Publication' [AIP] that is included in a package of books, charts and other documents which together make up an 'Integrated Aeronautical Information Package' [IAIP]. The primary publication is the AIP Book, which contains longer-term operational reference information of rules and procedures written in plain language and covering civilian operations in Australian airspace. In the AIP Book, the term 'should' implies that users are encouraged to conform with the procedure, whereas the term 'must' (or 'shall') means that the procedure is mandatory and is supported by CARs or CAOs. Amendments are issued quarterly and supplements are issued monthly. It is not a vital document for the individual pilot certificate holder to have in print form — and it is an ongoing task to cope with the amendments — but each recreational aviation club and flight school should maintain an AIP Book print amendment subscription. AIP is essential for operations in controlled airspace. The three standard sections of the AIP Book are 'General' [GEN], 'En route' [ENR] and 'Aerodromes' [AD]. The subsections of most interest to recreational aviation are: General [AIP GEN] GEN 1.5 section 1 — Radio communications systems GEN 2.2 — Definitions and abbreviations GEN 2.3 — Chart symbols GEN 2.7 — Sunrise/sunset tables GEN 3.2 — Aeronautical charts GEN 3.3 sections 1 to 3 — Air traffic services GEN 3.5 all sections — Meteorological services GEN 3.6 — Search and rescue En route [AIP ENR] ENR 1.1 section 17 — Operations in Class E airspace ENR 1.1 section 20 — Radio communication and navigation requirements ENR 1.1 sections 40 to 50 — Operations in Class G airspace ENR 1.1 sections 51 to 53 — Operational requirements — general ENR 1.2 — Visual flight rules ENR 1.4 all — ATS airspace classification ENR 1.7 all — Altimeter setting procedures ENR 5.5 all — Aerial sporting and recreational activities Aerodromes [AIP AD] AD 1.1 — Aerodromes/heliports availability AIP Supplements and Aeronautical Information Circulars [AIC] SUPs include operational information appropriate to the AIP. A SUP is published when the information is of a temporary nature and requires advanced notification such as planned military exercises that may close airspace to civil traffic. AICs contain information of a technical nature and are generally educational, giving advance notice of new facilities, services and procedures En Route Supplement The AIP 'En Route Supplement Australia' [ERSA] is recommended to all pilots with a cross-country endorsement — it is an essential document for cross-country flight planning and operations. ERSA contains details of PRD areas, area weather forecast codes and weather report decodes, pre-flight and in-flight information services, navigation aids, and emergency procedures. Its main purpose is to provide, within the facilities [FAC] section, full physical details of all licensed aerodromes [ADs] with current updates relating to those aerodromes available via NOTAM. The aerodrome entry includes the VHF and HF frequencies used for air traffic services, self-announce broadcasts, flight information service, Unicom and automated weather information services. It also provides control tower operating hours and thus the times at which a Class D CTR reverts to Class G airspace. ERSA is the only publication that indicates if a non-controlled aerodrome is certified, registered or military and thus mandates carriage and use of VHF radio when operating at the aerodrome or in its vicinity. ERSA also lists limited detail of a number of generally privately owned 'Aeroplane Landing Areas' [ALAs]. NOTAM are usually not issued for ALAs. All ADs and ALAs listed in ERSA are identified with an unique four-letter location indicator or identity code; the first letter of which is always 'Y'. There is no information in ERSA regarding recognised water alighting areas for seaplanes. The Aircraft Owners and Pilots Association of Australia [AOPA] publishes a biennial airfield directory containing limited information for about 2000 airfields (i.e. ADs, ALAs and airstrips), including those detailed in ERSA. More than 98% of those listed airfields are non-controlled — there are only 26 towered civilian aerodromes. Contact information for the owners/operators is included but the communications and navigation aid frequencies shown may not be current. The directory cost is about $50. NOTAM NOTAM, derived from the old term 'notices to airmen', are issued by Airservices Australia and contain "information or instructions concerning the establishment, condition or change in any aeronautical facility, service, procedure or hazard, the timely knowledge of which is essential to persons concerned with flight operations." The NOTAM (current at the time) are available from the Airservices Australia online pilot briefing service, which we discuss in the 'route planning' module. The Civil Aviation Safety Authority's Visual Flight Rules Guide is recommended reading and a PDF version of the November 2011 edition is downloadable from CASA's website. Check the Airservices Australia Publications Centre for purchase or subscription details for the publications mentioned. The charts within AIP are detailed in section 2.3. 4.1.7 VMC and the visual flight rules The two ICAO rule sets previously mentioned in section 1.1 are the Instrument Flight Rules [IFR] and the Visual Flight Rules [VFR]. Aircraft operating under the IFR are navigated by reference to cockpit instruments that process data received from ground stations or satellites. IFR flights may operate in both visual meteorological conditions [VMC] or instrument meteorological conditions [IMC] — see below. VFR flights may only operate in VMC. All national and international RPT jet flights into or between the major Australian cities would operate only in controlled airspace (Class A while en route) and under the IFR, but turbo-prop and piston-engined regional RPT aircraft, travelling to or from a smaller city, may operate some route sectors in Class G and under the VFR. Charter and business aircraft would tend to operate in both controlled airspace under the IFR or the VFR, and in Class G under the VFR. Agricultural aircraft would normally be operating in Class G and under the VFR, and may be encountered working at low levels close to airfields. General Aviation training aircraft would tend to operate in and out of a CTR under the VFR. Military aircraft operate everywhere but particularly important to light aircraft are their low jet routes where they may be flying at very low levels using terrain-following radar. Beware: fast-flying camouflaged military aircraft may also be encountered at very low levels outside the designated low jet routes. Visual Meteorological Conditions in Class E and Class G airspace RA-Aus/HGFA/ASRA operations and non-instrument rated pilot operations may only be conducted in VMC. The visual meteorological conditions (minima) applicable below 10 000 feet amsl in Class E and Class G airspace, and thus the VMC for most light aircraft operations (take-off, en route and landing) are: minimum average range of visibility forward from the cockpit — 5000 metres. ('Visibility' means the ability to see and identify prominent objects. A problem is that there may not be any prominent identifiable objects when flying over featureless areas. Also, few people are adept at judging distance from the cockpit.) horizontal cloud clearance — 1500 metres vertical cloud clearance — 1000 feet (i.e. above and below) if the visibility is less than 5000 metres or cloud clearance is below the minima, then IMC exist. (The image above is courtesy of CASA's Flight Safety Australia, March–April 2002 issue) If operating in Class G airspace at or below 3000 feet amsl or 1000 feet agl, whichever is the higher, an aircraft may operate 'clear of cloud' but remaining in sight of the ground — provided the aircraft is equipped with a serviceable VHF radio, the pilot has a radio endorsement, and the pilot listens out and transmits on the appropriate frequency. The 5000 metre visibility still applies. Note that this low-level 'clear of cloud' concession in the VMC does not apply in Class E. Note that a non radio-equipped aeroplane can then only operate in conditions where the cloud base is 1000 feet above the flight level. Thus such an aircraft can only take off and land when the cloud base is 1000 feet higher than the circuit height, and the horizontal cloud clearance is at least 1500 metres. Even when there is no regulatory requirement, carrying VHF radio and continually maintaining a listening watch is highly recommended. If holding a valid pilot licence enabling operations in Class D airspace (under Air Traffic Control) the VMC cloud clearance rules are relaxed to 600 metres horizontal, still 1000 feet above the cloud but 500 feet clearance below the cloud. Flight visibility remains at 5000 metres. (ATC may also permit 'special VFR' operations — within the Class D airspace — in weather conditions that do not meet the preceding criteria.) Visual Flight Rules The Visual Flight Rules applicable to most light aircraft operations are primarily 'see and avoid' other traffic, plus the following specifics: VMC must be maintained during the entire flight (climb, cruise and descent) and the flight conducted in daylight hours the pilot must be able to navigate by reference to the ground position fixes must be taken at least every 30 minutes. VFR 'on top' In addition, an aircraft cannot be operated on top of cloud that is more extensive than scattered, unless it is fitted with serviceable flight and navigation instruments as specified in CAO 20.18 Appendix IV — which includes an artificial horizon and directional gyro. Other restrictions apply — see AIP ENR 1.1 section 18.2 'Flight under the VFR'. Taking all into account, it is probably unwise for recreational aircraft to operate above any cloud cover. See adverse weather. Quiz question "You are at a non-controlled airfield (elevation 2700 feet and situated in flat terrain) and the base of an extensive layer of stratocumulus has been confirmed as 4000 feet amsl but visibility exceeds 10 km. Can you legally take off and depart the airfield?" Recreational aircraft operations (or any flight operation where the pilot in command [PIC] does not hold a night VFR rating or Command Instrument Rating) may only be conducted in VMC, and flight below 500 feet agl is forbidden except when taking off or descending to land. The visual meteorological conditions applicable below 10 000 feet amsl, and thus the VMC for take-off, en route and landing are: visibility of 5000 metres horizontal cloud clearance of 1500 metres vertical cloud clearance of 1000 feet. If operating in Class G at or below 3000 feet amsl or 1000 feet agl, whichever is the higher, an aircraft may operate 'clear of cloud' but in sight of the ground — provided the aircraft is equipped with a serviceable VHF radio, the pilot has a radio endorsement and the pilot listens out and transmits on the appropriate area frequency. Thus take-off for an aircraft that is not equipped with a serviceable radio would not be legal. The minimum altitude that a non-radio flight could be undertaken is 3200 feet amsl (2700 feet elevation plus 500 feet agl), and the vertical cloud clearance is then only 800 feet. However, a radio-equipped aircraft would be legal, provided operations were conducted between 500 and 1000 feet agl, thus 'clear of cloud'. The rationale for this is that radio provides the ability to alert other aircraft — possibly operating in the same restricted flight conditions — to your presence. VFR cruising altitudes Recreational aeroplane flights operating in Class G under the VFR must fly at cruising altitudes, selected in accordance with the table below, when at a height above 5000 feet amsl and, whenever practicable, should be operated at the appropriate cruising altitude when below 5000 feet. The cruising altitudes for aircraft operating under the IFR are in 1000 feet steps from 2000 to 10 000 feet; thus 5000 feet amsl is an IFR cruising altitude and not available to VFR aircraft. Operating in accordance with the cruising altitudes does improve safety, but pilots should be aware that the risk of collision still exists; for example, consider an aircraft tracking 175°, while to the south another aircraft is tracking 005° at the same correct altitude. Those two aircraft could well be closing on a collision course. As there is only 500 feet clearance between a VFR altitude and the IFR cruising altitudes above and below, it is most important that VFR pilots hold their altitude reasonably well. The aircraft flying IFR at the cruising altitudes will tend to be smaller turboprop and piston engine aircraft so not as visible as the large transport aircraft. Sailplanes of course are not subject to these rules. Also there is nothing in the rules that prevents a situationally aware recreational pilot in an appropriately equipped aeroplane from taking off, climbing to 100-200 feet below 10 000 feet in Class G airspace, doing a couple of 360° turns to admire the landscape and descending for landing. Magnetic tracks 000° to 179° 180° to 359° Cruising altitudes (area QNH) 1500 feet 2500 feet 3500 feet 4500 feet 5500 feet 6500 feet 7500 feet 8500 feet 9500 feet Note: there are no cruising levels available in the transition layer so VFR aircraft must not use 10 500 feet (FL105), and 11 500 feet (FL115) is not available if area QNH is below 997 hPa. Flight at the control area lower level boundary AIP ENR 1.4 paragraph 1.1.7 states: "When ATS airspaces adjoin vertically (one above the other), flights at the common level must comply with the requirements of, and will be given services applicable to, the less restrictive airspace." In this context Class G is the least restrictive airspace, followed by Class E, Class D, Class C and finally Class A as the most restrictive. Thus if the lower limit of a Class C control area step was 5500 feet with Class G below, a VFR aircraft could legitimately cruise at 5500 feet in that area without requiring ATC clearance — provided of course that height keeping is good, the altimeter is very accurate and the correct QNH is set. Air traffic controllers keep aircraft at 500 feet plus above the lower level of the controlled airspace to provide clearance from Class G traffic. However, be aware that the wake turbulence from heavy aircraft sinks and drifts downwind. Also there is a problem with selecting which QNH altimeter setting to choose. So, taking everything into account, it is not a good idea to fly at the airspace intersection level. 4.1.8 RA-Aus/HGFA/ASRA powered aircraft flight operations RA-Aus/HGFA/ASRA registered aircraft must operate in VMC and in Class G or Class E, except with special permission (see below) to operate within a Class C or D control zone — such permissions are usually applied on a long-term basis and only to pilots who also hold a valid pilot licence plus the RA-Aus/HGFA/ASRA Pilot Certificate. Recreational aircraft operating within Class E airspace should be radio and transponder equipped. Suitably equipped recreational aircraft should also operate under the VFR. The minimum equipment list [MEL] required to do so is a serviceable magnetic compass, altimeter (accurate to 100 feet) and airspeed indicator, plus an accurate watch or clock available to the pilot. All aircraft, including recreational aircraft, operating above 5000 feet amsl, must be equipped with a serviceable VHF radio; and the pilot, with an appropriate radio endorsement, must make the broadcasts specified in AIP Book. RA-Aus/HGFA/ASRA aircraft may only be flown at a height of 10 000 feet above mean sea level or higher if a written approval for that flight has been issued by CASA. Flight over cities and towns Generally a factory-built aeroplane must not be flown over a closely-settled area at a height from which it cannot glide clear of the closely-settled area to a suitable landing area and the minimum height is 1 000 feet above ground level. 'Suitable landing area' means an area in which an aeroplane can be landed without endangering the safety, or damaging the property, of persons unconnected with the aeroplane. Home-built — and some factory-built — aeroplanes are prohibited from flight over closely-settled areas, but for expanded information see 'Flight over the built-up area of a city or town'. Recreational aircraft operations in Class C and D control zones To operate in Class C and D control zones, the recreational aircraft and the engine must either be certificated to the design standards specified in CAO 101.55 para 6.1 or meet criteria specified in the exemption CAOs (e.g. see paragraph 7.3 (a) ii and iii) in CAO 95.55); be fitted with a certificated or CASA-approved engine and is fitted with a radio capable of two-way communication with air traffic control; and the pilot in command must hold a valid Pilot Licence ( i.e. Private Pilot Licence — PPL, Commercial Pilot Licence — CPL, Air Transport Pilot Licence — ATPL) in addition to the Pilot Certificate. Even so, it is unlikely that, if it came to a judicial test, a recreational aircraft would be legally be able to operate from, or enter, most Class D CTRs as the 'lanes of entry' to such airfields usually involve overflight of closely-settled areas, and overlying Class C airspace may severely limit available altitude (and thus gliding distance) in such lanes. A transponder must be operated in Class C CTRs and CTAs. Recreational aircraft must comply with the flight conditions specified in the relevant exemption CAO. For example section 7.1 (h) of CAO 95.55 forbids flight of factory-built aircraft over a closely-settled area at a height from which it cannot glide clear of the closely-settled area to a suitable landing area; and that is lower than 1000 feet above ground level. Home-built aircraft must not be flown over a closely-settled area except under conditions and limitations that CASA or an authorised person considers necessary. Be mindful that it is the legal responsibility of the pilot, not the ATS personnel, to ensure compliance with the exemption CAOs and other regulations. Air traffic controllers presume that the pilot of an aircraft requesting entry into their airspace is legally, medically and practically qualified to do so and a subsequent airways clearance does not absolve the pilot of legal responsibility. Also bear in mind that the entities owning Class C and D aerodromes (and others) may publish their own 'conditions of use' which users should be aware of, and comply with. Recreational aircraft, operating under the Visual Flight Rules with area QNH set, may cruise at any safe altitude below 5000 feet above mean sea level. However, a prudent pilot undertaking a flight of reasonable length would choose a hemispherical VFR cruising altitude whenever practicable. For any aircraft track with an easterly component, the VFR cruising altitudes are 1500 and 3500 feet below 5000 feet; plus 5500, 7500 or 9500 feet if the aircraft is radio-equipped. 4.1.9 Communication and navigation aids Civil aviation radio communications are conducted primarily in the aviation very high frequency [VHF] communications [COM or COMMS] band, 118.00 to 136.975 MHz, where, at 0.025 MHz steps, there are 760 channels possible. In the less accessible areas of Australia, where there is no VHF ground coverage, communications must be in the various high frequency [HF] network bands between 3400 and 9500 kHz. The PCA shows VHF coverage (but not FIA boundaries or frequencies) and the appropriate short-wave frequencies in the three domestic HF network areas. Military aircraft primarily use UHF communications. There is an inter-pilot air-to-air communications frequency available at 123.45 MHz. More information on frequency allocation for club, sport aviation and other aviation activities is contained in the aircraft station operating frequencies section of the VHF Radiocommunications Guide. In Australia, the VHF Omni-directional Radio Range [VOR] primary air route, homing and position-fixing navigation aids operate in the 112.1 to 117.975 MHz aviation VHF navigation [NAV] band. The Instrument Landing System runway localisers at larger airports operate in the 108.00 to 112.00 MHz VHF NAV band. Thus the aviation VHF NAV/COM band is from 108.00 to 136.975 MHz, with some 200 channels (at 0.05 MHz intervals) in the NAV band and 760 in the COM band. Some hand-held airband COM transceivers have a very limited VOR receiver capability, but the full NAV/COM capability is confined to more expensive panel-mounted transceivers/VOR receivers/VOR indicators coupled to a VOR antenna. Non-directional aviation radio beacons [NDBs], installed to provide a homing facility for smaller aircraft, transmit in medium wave bands between 190 and 535 kHz. The companion airborne automatic direction finding receivers [ADFs] can also pick up transmissions in the 520 to 1611 kHz AM broadcast band, depending on the power output of the radio station. The broadcasting frequency, latitude and longitude, power output in kW and the height of the mast agl (quite a few are over 600 feet agl and situated on the high ground) for all AM broadcast stations, is contained in the ERSA NAV/COMMS section. The location of some AM broadcast stations' transmitter masts is shown on World Aeronautical Charts [WACs], with the station identification but not the frequency. Most licensed aerodromes have an NDB and many would have a VOR. 4.1.10 Distress frequencies and AusSAR When a pilot is experiencing in-flight difficulties, it is advisable to inform others as early as practical and to advise whether the pilot considers the situation to be an emergency or something less. The frequency on which a distress call (a MAYDAY transmission) or an urgency message (a PAN-PAN transmission) is made should be that which is likely to provide a quick response — usually the area frequency. If a registered civil or ultralight aircraft comes to grief away from a controlled aerodrome or is reported missing, Australian Search and Rescue [AusSAR] has national responsibility for coordinating the search and rescue. More information is contained in the safety and Safety and emergency communication procedures module of the 'Coping with Emergencies Guide'. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
Hi to all I wanted to post to keep you informed on how the site is going. Basically I think the features, the functionality and even the way the site looks is absolutely great and it is exciting for me as I know how much more is coming. Yes, I have tormented you by going back and forth with software solutions and for that I am truly sorry but both solutions have their pros and cons but overall now I can see what we have is the best solution for the future. There are a lot more FREE resources, Downloads and Tutorials to come, we have free books and manuals to download, great online calculators and tools and comprehensive educational tutorials all FREE for everyone to use. We have weekly competitions running where users can win prizes and soon we will have a weekly Clear Prop special. Also coming soon are Aviation Articles, News, Classifieds, Educational Quizzes, Airfields Directory and much more all helping to make Recreational Flying (.com) an extensive and powerful online resource for all aviators. What we all need to do now is to get as many more people to come on in and start using the site and that is where I need your help. Not only in letting people know about everything the site has but also to populate 2 very important sections of the site; Groups and Suppliers. For Groups I would like to get every aviation club and school to have their own presence here on Recreational Flying by them creating their own Group, a mini website itself with heaps of features, where they can promote their club/school to all users of the site, not just registered users but the thousands of visitors that come to the site. I am always available to help them to create their own presence here on Recreational Flying and remember you can create your own group for any special interest as well...perhaps an aircraft type group or an engine type group etc. So please get your Club or School to create a group here. The other powerful area that needs your help is the Suppliers Section. This new section has a vast range of features to help suppliers promote their products and services in the aviation arena. i would like to see all aircraft manufacturers, distributors, agents etc along with all the different suppliers of everything we aviators need listed in the Suppliers Section to help all Recreational Flying users visiting this great resource...but I need your help to do it. If you know any Aircraft Distributor or Supplier please klet them know they can get a presence here on Recreational Flying and its FREE or to help the site they can get many extra features for a small monthly contribution to the site. Please help me to help you in making Recreational Flying (.com) the best resource on the internet for every fellow aviator.
-
Try and use the normal attachment process in a post...like adding an image to a post except select your video...let's see how that goes
-
The recomendation is to upload it to YouTube and then add the YouTube link to your post or add it to the Video Section which enables you to add it wherever you like. I can turn on the ability to upload it directly to here but the storage and hosting costs would increase dramatically and the CDN (Content Distribution Network) cache is limited to a 100mb. If I do enable video upload then I may see enormous video uploads being made...the thing is do I risk it???????????????????????
-
Designed for ground instructors, flight instructors, and aviation maintenance instructors, this Aviation Instructor's Handbook was developed by the Federal Aviation Administration (FAA) in cooperation with aviation educators and industry to help beginning instructors understand and apply the fundamentals of instruction. It provides up-to-date information on learning and teaching and how to apply this to the task of teaching aeronautical knowledge and skills to learners. Experienced aviation instructors will also find the information useful for improving their effectiveness in training activities. This book is a key reference tool to all the information necessary for operating as an authorized instructor and passing the Fundamentals of Instructing (FOI) FAA Knowledge Exam. Subjects covered include risk management and single-pilot resource management, human behavior, the learning process, effective communication, the teaching process, assessments, planning instructional activity, instructor responsibilities and professionalism, and techniques of flight instruction. Appendices include a comprehensive bibliography of references, information on how to develop a test item bank, certificates and ratings endorsements, and a personal minimums checklist. This new edition expands and updates the existing material, including scenario-based training relative to assessments, the submission process of an Airman Certificate and/or Rating application through IACRA, and endorsements. It also incorporates new areas of safety concerns and technical information not previously covered, such as referencing the Airman Certification Standards (ACS) alongside the Practical Test Standards (PTS), teaching practical risk management during flight instruction, and information for Remote Pilots. This book is the official FAA source for teaching flight and many test questions for the FAA Knowledge Exams for instructors come from this reference. Complete with chapter summaries; detailed, full-color drawings and photographs throughout; and a glossary and index. 2016 Edition book is available for download from the Recreational Flying Downloads section
-
Version FAA-H-8083-9A
17 downloads
Designed for ground instructors, flight instructors, and aviation maintenance instructors, this Aviation Instructor’s Handbook was developed by the Federal Aviation Administration (FAA) in cooperation with aviation educators and industry to help beginning instructors understand and apply the fundamentals of instruction. This handbook provides up-to-date information on learning and teaching, and how to relate this information to the task of teaching aeronautical knowledge and skills to students. Experienced aviation instructors will also find the information useful for improving their effectiveness in training activities. This book is a key reference tool to all the information necessary for operating as an authorized flight instructor and passing the Fundamentals of Instructing FAA Knowledge Exam. Subjects covered include human behavior, the learning process, effective communication, the teaching process, assessments, planning instruction activity, instructor responsibilities, techniques of flight instruction, and risk management. Appendices include a comprehensive bibliography of references, information on how to develop a test item bank, certificates and ratings endorsements, personal minimums checklist, flight instructor endorsements, and relationships of decision-making models. Newly illustrated with full-color drawings and photographs.Free -
Some preliminary refresher notes The airspeed at which an aircraft stalls depends in part on the wing loading — the ratio of lift force generated to aircraft all-up mass expressed in units of 'g'. If a wing reaches the critical angle of attack under an aerodynamic load higher than 1g, the stalling speed will be higher than the normal 1g stall speed for that particular mass and wing configuration, and the effects of that accelerated stall are usually more pronounced than a 1g stall. An accelerated stall is not a 'high-speed' stall — the latter is one form of accelerated stall. Uncoordinated or cross-controlled flight: applying pressure to the rudder in one direction with opposite aileron applied is cross-controlling. This is, normally a rather sloppy way to fly but also a condition that can lead to an uncommanded roll if you inadvertently exceed the critical angle of attack — particularly in uncoordinated climbing or lower speed descending turns, such as in the approach to landing. It may also be the condition when a mis-rigged aircraft flies 'one wing low'. That being said, a planned and properly executed cross-controlled sideslip during final approach IS a normal and safe flight manoeuvre. Once established in a coordinated level turn, the lower inner wing has a slightly lesser airspeed and thus less lift than the outer wing, which produces a tendency for the outer wing to rise and the bank angle to increase. This requires the pilot to apply a slight opposite pressure to the control column which is known as 'holding-off bank'. This is quite normal and the pilot may not notice doing so because it is just part of maintaining the chosen bank angle throughout the turn. In a climbing turn, the outer wing has a slightly greater effective aoa than the inner wing and thus additional lift. Combined with its faster speed, this reinforces the tendency for the bank angle to increase and the need to hold-off bank. However, in a descending turn, the steeper path of the inner wing means that it will have a larger effective aoa than the outer — which may compensate, or over compensate, for the faster velocity of the outer wing. In order then to maintain the required bank angle, it may be necessary to apply a slight inward pressure to the control column; i.e. in a coordinated descending turn, the bank may be 'held on'. Should an aircraft be stalled inadvertently in a coordinated turn, both wings usually display the same progressive stall pattern — thus there should be no pronounced wing drop. When flying at speeds below 1.3 times Vs, the aileron moments are much less effective than at cruise speeds and larger aileron deflections are needed to bank the aircraft. There is always a tendency to be more forceful than necessary, thus overbanking the aircraft at a critical stage. The same applies to rudder effectiveness, so more coordinating rudder is required at slow speeds. In the following text, 'top/bottom rudder' refers to the relative position of the rudder pedals when turning; 'top' being the rudder pedal opposite the lower wing. Thus, if the aircraft is banked and turning to the left, then pressure on the right rudder pedal will apply top (or outside) rudder or pressure on the left rudder pedal will apply bottom (or inside) rudder. An excess of bottom rudder produces a skidding turn. Too much top rudder produces a slipping turn or may even halt the turn, thus producing a full sideslip. In a coordinated turn, there is just sufficient bottom rudder applied to keep the slip ball centred. 3.16.1 Awareness of angle of attack increase in a turn As a consequence of providing the centripetal force for a sustained turn, the lift force (i.e. wing loading) must be increased as angle of bank increases. Lift increases rather slowly up to a bank angle of 30° — where it is 15% greater than normal level flight loading — after which it increases rapidly, being 41% greater at a 45° bank angle. At this angle, the load on the airframe is 1.41g. The right-hand column in the following table shows the increase in stall speed, which is proportional to the square root of the wing loading. You can see that the percentage increase in stall speed is about half the increase in lift force. Bank angle Cosine g load (lift increase) Vs multiplier (increase) 10° 0.98 1.02 (+2%) 1.01 (+1%) 15° 0.965 1.04 (+4%) 1.02 (+2%) 20° 0.94 1.06 (+6%) 1.03 (+3%) 30° 0.87 1.15 (+15%) 1.07 (+7%) 40° 0.77 1.30 (+30%) 1.14 (+14%) 45° 0.71 1.41 (+41%) 1.19 (+19%) 50° 0.64 1.56 (+56%) 1.25 (+25%) 54° 0.59 1.70 (+70%) 1.3 (+30%) 60° 0.50 2.00 (+100%) 1.41 (+41%) The lift force increase in a constant-speed turn is provided by an increase in the lift coefficient [CL], which in itself is brought about by increasing aoa. Obviously, increasing CL implies an increase in drag and loss of height, or change in the rate of climb/descent, unless power is increased. A rule of thumb for light aircraft with normally cambered wings is that each 1° aoa change — starting from 2° and continuing to about 14° — approximates to a 0.1 CL change, and each 0.1 CL increase/decrease at a constant airspeed represents a wing loading change of roughly 8%. So, from the table above, a 30° bank angle in a sustained turn adds 2° to the basic aoa for the airspeed, a 45° bank angle adds 5° and a 60° angle adds 12°. The basic aoa for normal descending and climbing speeds in the circuit are probably around 6–8° and 6–10° respectively. Anything more than a moderate 30° banked turn decreases the safety margin between the effective aoa of some sections of the wing and the critical aoa. Wing loading must also change with the payload carried, as does the stall speed and the performance speeds. If a recreational light aircraft is normally flown with just the pilot on board, the aoa associated with a particular calibrated airspeed is significantly less than when flying at the same airspeed with a heavy passenger and perhaps a full fuel load. For example, suppose the aircraft is normally flown with only the pilot on board and an all-up weight of 400 kg. But when flown with a heavy passenger and full fuel, then all-up weight increases to 540 kg. Then the wing loading increases by 35%, thus CL and the aoa for any particular CAS will be greater than the pilot is accustomed to — maybe 2° or 3° at low airspeeds — and much less at high airspeeds. 3.16.2 Loss of control in an uncoordinated level turn If an aircraft is being held in a level turn at a particular bank angle with constant power, and excess bottom rudder is applied and held, the aircraft will rotate about the normal axis (yaw) in the direction of rudder deflection. Airspeed over the outer wing increases slightly while that over the inner wing decreases, thereby producing a lift differential; thus there will be a secondary roll effect that increases the bank angle. At the same time, the yaw increases fuselage drag and decreases airspeed — and thus lift — and the nose drops a little. This is an uncoordinated skidding turn, which often happens when the pilot tries to 'hurry' the turn with bottom rudder instead of increasing bank. We have a situation where the aircraft is overbanking with the nose yawing inward and downward. If the pilot reacts by applying and holding opposite aileron to restore the required bank angle — i.e. holding off bank — then due to the downward deflection of the inner aileron, the outer 30% or so of the lower wing is flying at a much higher aoa than the corresponding section of the higher wing. (If equipped with flaperons, the whole lower wing would be flying at a higher aoa.) The lower wing will also be producing more aileron drag, so the inward and downward yaw will increase and there will be a tendency for the pilot to raise the nose by increasing control column back pressure. This increases aoa overall, while at the same time speed will continue to decrease because of the increased fuselage drag, unless power is increased. The pilot is now 'pushing the design manoeuvring flight envelope'. Any consequent tightening of back-pressure on the control column to raise the nose (or any inadvertent back pressure applied when, for instance, looking at something of interest below you; looking over your shoulder; being distracted by fiddling with something in the cockpit; using the radio; or even any encountered atmospheric turbulence, wake turbulence or gust shear) may take the aoa of the inner wing past the critical angle. The aircraft loses its lateral stability (i.e. positive roll damping) and it is most likely that the lower wing will drop in an uncommanded roll, and thus become increasingly more deeply stalled than the upgoing wing — which may not be stalled or just partly stalled. If that initial roll is not promptly recognised as a stall or partial stall and it is allowed to continue — or perhaps it is incorrectly countered with opposite aileron without first unstalling the wing(s) by easing forward on the control column — then the increasing aoa of the lower wing deepens the stall and causes greatly increased asymmetric drag. Additional yawing forces in the same direction as the lower wing come into play, the nose-down pitching moment increases and the nose drops further. This is the incipient spin condition where autorotation is about to commence, which will happen quickly and in some aircraft very quickly indeed. The result is the stall/spin fatality you hear about when an unwary pilot allows such to develop without sufficient height to recover; and of course you say 'How sad it is for the family' — while thinking — 'but I'm too wary to get caught by such a simple mistake! But you don't know how many times you have come within a hair's breadth of eternity without being aware of it. If the cg is aft of the rearward limit, the amount of elevator deflection needed to bring the aircraft to the critical aoa is reduced; i.e. just a relatively small rearward movement of the control column may rotate the aircraft to the critical aoa. If MTOW exceeds the design limit and/or the cg is aft of the rearward limit then recovery from the initial stall may be impossible. The rules to avoid such situations are: • always maintain a safe speed near the ground consistent with the bank angle employed • continually envisage the wing aoa; i.e. how it's flying • keep the slip ball centred; i.e. never apply an excess of bottom rudder in an attempt to tighten any turn if height is below the safe recovery height (3000 feet agl perhaps) for a fully developed spin. Height loss in a stall/spin incident The height lost during a normal stall and recovery incident in a very light aircraft is probably between 50 and 250 feet depending on the aircraft type, the aircraft attitude at stall and the pilot's awareness. Loss of height in a stall/spin event is very much greater — perhaps 100–300 feet during the incipient stage, 200–400 feet to stop the autorotation and 300–500 feet during the recovery: a total of 600–1200 feet. This is why low-level stall/spin events are so deadly. 3.16.3 Loss of control in an uncoordinated descending turn The precursors to a stall/spin event in a low-power descending turn are the same as those for such an event in a level turn: if an excess of bottom rudder is applied, the aircraft will be skidding. Unless some other factor is dominant, then whenever an aircraft is slipping or skidding in a turn, the wing on the side to which the rudder is deflected will usually stall before the other, resulting in a consequent instantaneous roll in that direction. At descent speeds, the aircraft is usually flying at a higher CL and thus higher aoa, than when on the downwind leg (for example) — so there is a reduction in available aoa margin before allowing for the additional aoa required for the turn. The descending turn from base leg onto the final approach to landing is the most obvious place for a pilot to attempt to hurry a turn with rudder, because of the need to align with the runway. A tailwind component on base leg in a crosswind landing will increase the tendency to hurry the turn with rudder, as may other crosswind situations. If skidding, the excess bottom rudder is yawing the nose down and the tendency is to use elevator to keep it up, which is going to bring the aoa towards critical. Also, because of illusory ground reference cues, there may be a tendency to increase the rate of turn by applying additional bottom rudder whilst maintaining the bank angle with opposite aileron — 'holding off bank'; and you should never hold-off bank in a descending turn. If control column back-pressure is purposely or inadvertently applied, the aircraft may enter a cross-controlled stall where it is going to snap further into the bank and enter an incipient spin. Apart from the turn from base to final, such stalls might occur on final when avoiding a bird strike; or attempting a late correction to an out-of-line crosswind approach; or any time when you try to hurry a turn with bottom rudder. Stalls on the final approach — caused by failing to increase power when raising the nose to stretch the approach or reduce a high sink rate — will be exacerbated if the aircraft is also slipping. Probably the most dangerous low-level descending turn is the turn-back following engine failure after take-off; see 'The turn-back; possible or impossible — or just unwise? '. If flying cross-controlled when banked with an excess of top rudder — as in the sideslip manoeuvre, or a slipping rather than skidding turn — then if the aircraft stalls, the roll will probably be anti-spin; i.e. in the direction of the upper wing — towards an upright position — which is not quite so alarming and provides a little more time to react and reduce aoa. 3.16.4 Loss of control in a low-level climbing turn As we saw above, the increased lift force in the turn is provided by an increase in aoa. Now what will happen if you are climbing at Vx (the speed for maximum climb angle) using maximum power and decide (because of rising terrain or other obstruction, an approaching aircraft or just natural exuberance) to make a quick 30° left turn using a 45° bank angle, while still maintaining the climb? Coordinated climbing turn: if you do not keep a close eye on the ASI and the airspeed has decayed just a little, the general aoa at Vx could be around 12°. To initiate a 45° bank turn, wing loading and thus aoa must increase by 41%, which will take the aoa to 17°; i.e. past the critical stall aoa of 15° or 16°. Such full-power stalls in a coordinated climbing turn tend to result in the outer wing stalling first — because in a climbing turn, the outer wing has a slightly higher aoa than the inner — with a fairly fast outer wing and nose drop. The roll towards the outside of the turn would initially level the wings, but the increasing aoa of the down-going wing continues to accelerate the loss of lift and increases the drag on that wing. This is a particularly rapid action if the propeller torque effect is such that it also reinforces the roll away from the original direction of turn. P-factor may also cause the aircraft to yaw when flying with high power at high angles of attack. Such stalls are likely to result in a stall/spin event if corrective action is not taken as soon as the initial loss of roll stability — the uncommanded roll, or just a wing rocking warning — is apparent. Cross-controlled climbing turn: if the turn is skidding — i.e. with excessive bottom rudder applied —then the lower wing may stall first with the consequent roll into the turn because only one wing is stalled. This may be sufficiently pronounced to flick the aircraft onto its back. The propeller slipstream from a tractor engine will also be slightly asymmetric, as it supplies more dynamic pressure and thus lift to one wing while reducing the effective aoa. We will discuss cross-controlled climbing turns further when we look at illusory ground reference cues. Even a 30° banked climbing turn at a Vx will produce an aoa of 14°. This is very close to the critical aoa and provides no margin for even minor turbulence, slight mishandling or inattention. Of course, climb performance will be degraded unless extra power is available, which is unlikely because full power is normally used for the climb until a safe height is reached. The aoa margin, which you should always have in hand to cope with such likely events, is 3 or 4°. This indicates that, when climbing at Vx, turns should not be contemplated. When climbing at Vy — the best rate of climb airspeed with aoa around 8° — until a safe height has been gained, turns should be limited to rate 1 (180° in azimuth per minute, requiring about 15° bank) to ensure an additional margin if wind/gust shear is encountered in the climb-out. When entering a turn during a full-power climb, the aircraft must slow because of the increased drag at the higher aoa with no excess power available to counter it, so the aircraft's pitch attitude must be reduced sufficiently to maintain safe airspeed. Although recreational light aircraft — with their low wing loading — normally display quite benign stall characteristics when slowly decelerated to stall speed in straight and level flight, they will exhibit quite different behaviour when a stall is initiated during an uncoordinated turn; and such is the usual unintentional stall situation. Under these circumstances, the height lost during the incipient spin plus recovery — i.e. before developing autorotation — may be 200 to 400 feet or more. Thus, a wing-dropping stall event is highly dangerous when occurring in the circuit pattern or in any other low-level flight situation. 3.16.5 Standard recovery procedure for all stall types One standard recovery procedure is generally applicable to all stall events or attitude upsets in a three-axis aircraft, whether or not overbanked and/or overpitched — i.e. nose high/low — though this recovery procedure is not applicable to a fully developed spin, whether erect or inverted. Stall recovery generally requires the following concomitant stages: Ease stick back-pressure to reduce aoa of the most stalled wing below critical — which immediately gets the aircraft flying and restores normal 3-axis control. For any aircraft type, the amount of elevator deflection required to unstall the most stalled wing depends on many variables and may range from just an easing of back-pressure to a firm but smooth push towards the neutral position. All aircraft have their own handling idiosyncrasies and pilots must be aware of them. The nose should be positioned sufficiently below the horizontal to achieve safe flying speed while still well clear of the terrain. It's a matter of balancing height loss and proximity to terrain against a quick return to a safe flight speed. If the forward stick movement is both excessive and abrupt, the result could be an aoa movement below the zero-lift aoa, in which case there will be a reversed lift force on the wings that hinders recovery. This may be particularly apparent with trikes. The negative g due to the bunt could adversely affect some engines at a critical time. In instances of extreme overbanking (past 60° or inverted) — where although the upset may be the result of a cross-controlled stall or perhaps wake turbulence — the inverted or near-inverted wing will not be stalled but the aircraft will be in an inverted descent. The forward control column movement is needed to reduce the angle of descent. However, there may be the possibility of an inverted stall if the control column is pushed into its extreme forward position. Warning: never pull BACK on the control column as the initial response to a perceived stall or an overbanked nose-low attitude. Halt downward wing movement with rudder or centre the slip ball. Increase power smoothly, possibly up to maximum. The slipstream will also increase rudder and elevator authority, and aircraft stability, through its effect on fin and horizontal stabiliser; though if the aircraft is near the wings-vertical position — or is inverted — the throttle must be closed. In the recovery from a stall in a climbing turn, full power should be maintained unless the nose is pitched too far down. Roll the wings level with aileron so that all the lift force will be directed away from the ground. If inverted, choose the roll direction that provides quicker return to a wings-level attitude and, of course, the right way up. Following the preceding actions: adjust power as necessary; if flaps were fully lowered then adjust by stages to take-off position; hold attitude until speed has built up to Vy (perhaps Vx if there are terrain problems); then ease into a climb to a safe altitude, where you can assess what went wrong. Never attempt to continue a landing approach after such an event; go around, allowing plenty of time to assess the environment before re-approaching. If the aircraft is properly balanced (i.e. cg is within the limits for that all-up weight), any cross-controlled stall condition is readily countered. Of course if the pilot doesn't wait for the airspeed to build to a safe speed before again applying control column back-pressure, there will be a high risk of a secondary stall which may be very hazardous, depending on the height loss from the first stall. A document titled 'Don't stall and spin in from a turn' expands the material presented on this page and is available in the 'Decreasing your exposure to aerodynamic risk' guide. 3.16.6 Succumbing to illusory ground reference cues It is thought that some ground reference optical illusions may be a contributory factor in situations of loss of control near the ground. Such illusions can cause no problem in the circuit if the pilot confines external scanning to the intended flight path and checks for conflicting aerial traffic, while maintaining the appropriate instrument scan and a minimum safe flying speed. The latter is 1.5 times Vs, or perhaps as low as 1.3 times Vs in the latter part of a stabilised final approach as long as bank angle never exceeds 20°. Fixing the circuit pattern on particular ground reference points, rather than the landing strip (for example "turn downwind around the big tree"), may contribute to illusory ground reference cues. Wind drift illusions When wind speed is reasonably high relative to aircraft speed, then the aircraft's drift with reference to the ground is very apparent to the pilot operating at lower levels, and particularly at short, difficult airstrips. The diagram above represents the ground track of an aircraft conducting a level 720° coordinated turn with constant speed and constant bank angle, such that in the second 360° turn, the aircraft would be encountering its own wake from the first 360° turn — assuming that the wake didn't sink below the flight path. The movement of the air mass in which the aircraft is borne is toward the west (with an easterly wind) and the turn is clockwise when viewed from above. When in the region above the red line, ground speeds will be lower; when below the red line, ground speeds will be higher. The separation of the tracks for each 360° is exaggerated for clarity. When entering the south-west quadrant of the first 360°, the ground speed is initially high but reducing. The drift away from a central ground reference would provide the illusion of skidding out of the turn. Passing through the north-west quadrant, the skidding illusion will disappear as ground speed reaches the minimum. Ground speed starts to increase through the north-east quadrant. However, the increasing drift towards the reference point provides a very noticeable illusion of a slip into the turn. This reaches a maximum as the aircraft enters the south-east quadrant, where it abates as ground speed increases. So, in a 360° coordinated level turn with constant speed and constant bank, the aircraft (and its wake) drifts downwind relative to the ground at the wind speed rate. The cockpit instruments will of course show a constant airspeed, bank angle and a centred slip ball. However, the reference cues seen by a pilot looking at the ground during a low-level turn indicate increasing and decreasing airspeeds, alternating with decreasing and increasing slip. The downwind turn An unaware pilot may get into a difficult situation in the low-level circuit when an aircraft is turning 90° from crosswind to downwind (as in the progress through the SE quadrant of the diagram above), when drift cues create an illusion of slipping into the turn. At the same time, the increasing ground speed might suggest increasing airspeed. The reaction of an unwary pilot is to increase bottom rudder pressure. This will increase the bank angle and lower the nose. The pilot's reaction may well be to apply opposite aileron to reduce the bank, while increasing control column back-pressure to bring the nose up and possibly reducing power to reduce airspeed. Thus the aircraft is cross-controlled and flying at an aoa with little margin in reserve. This is coupled with decreasing airspeed, reducing lift and the aircraft sinking with a consequent increase in effective aoa. Under such circumstances, there is a likelihood of the aircraft stalling and snapping over. The downwind turn illusion seems to have more potential for error if the aircraft is climbing in a downwind turn. Note: sometimes you may read material which purports that an aircraft loses airspeed and might stall when turning from crosswind to downwind because the aircraft is changing direction relative to the wind direction, which of course is nonsense. However, airspeed must decrease in the turn if power is not increased to counter the extra induced drag. Although an aircraft can only stall if the critical angle of attack is reached, a combination of aircraft inertia and a wind shear or turbulence event encountered in the turn could result in a stall (particularly if it is still climbing) or, more likely, a loss of height. If turning very close to the ground to follow a particular ground path (close to trees when stock mustering, for example) the increasing drift into the turn must be allowed for. If you have doubts then imagine operating at 3000 feet agl with a 35 knot gradient wind and using the upper side of a layer of smooth stratus as the airfield surface for simulated circuits. Fly three squares, each leg of one minute duration, at 1.5xVs1 and 400–500 feet above the layer, making 30° balanced turns at the corners. Vary the alignment of each square by 30° in order to achieve near-crosswind alignment in at least one circuit. You will notice that 'ground-speed' does not vary in any part of each circuit (except for a small reduction in airspeed during every balanced turn), there are no drift illusions and nothing changes when turning from crosswind to downwind. You will also notice that in these conditions there is little turbulence. Pivotal height and reversal height Pivotal height or pivotal altitude is a term used by proponents of ground reference manoeuvres such as 'eights on pylons'. It is one particular height above ground at which, from the pilot's sight line, the extended lateral axis of an aircraft doing a 360° level turn (in nil wind conditions) would appear to be fixed to one ground point, and the aircraft's wingtip thus pivoting on that point. Imagine an inverted cone with its apex sitting on the ground reference point and an aircraft flying around the periphery of its inverted base while maintaining a constant airspeed. The vertical distance from the reference point to the centre point of the inverted base is the pivotal height, and the distance from the edge to that centre point is the turn radius. The bank angle is formed between the outer wall of the cone and the radius line. The pivotal height in nil wind conditions is readily calculated by squaring the TAS in knots and dividing by 11.3. So any aircraft circling at a speed of 80 knots would have a pivotal height (80 × 80 / 11.3) around 550 feet, no matter what the bank angle. In other than still air conditions the pivotal height varies with the ground speed. If the wind was northerly and the aircraft was turning anticlockwise (viewed from above), then ground speed would be lower on the eastern side of the turn and higher on the western side. When in the northern quadrant the aircraft would be drifting towards the centre point, while in the southern quadrant it would drift away. Drift would not be noticeable in the eastern and western quadrants but changed ground speeds would. At 70 knots ground speed, the pivotal height is reduced to 450 feet; at 90 knots it is about 750 feet. (Thus an exercise requiring a continuous 360° balanced turn at constant speed around a ground reference point, whilst holding pivotal height, involves continually changing the height above ground so that the line of pivot around each point is held constantly — rather than maintaining a constant distance from the 'pylon'. The bank angle must also be changed constantly as the wind drifts the aircraft towards or away from the pivot point. It is not an easy exercise to do well, and requires an ability to manoeuvre accurately whilst including the ground reference point in the normal scan pattern. Usually two ground reference points, about five seconds apart, are included for a figure eight pattern — otherwise known as 'eights on pylons'.) Now imagine two cones — the upper one is the inverted cone with the aircraft flying around the edge of its inverted base and below that is a second cone with its base on the ground and its apex connecting with the apex of the upper cone. The vertical distance from the ground through the cone intersection to the centre point of the inverted base is the aircraft height. So when an aircraft is turning at pivotal height in nil wind conditions, the wingtip appears to be fixed to a single point in the landscape. But when at any height other than the pivotal height, the wing tip will appear to move across the landscape. When an aircraft is turning at a height greater than the pivotal height, which is the normal situation in flight, the wingtip appears to move backwards over the landscape — path A in the diagram. However, when an aircraft is turning at a height less than the pivotal height (thus close to the ground), the wingtip appears to move forward over the landscape — path B in the diagram. Thus, when a turning and descending aircraft descends below pivotal height there is an apparent reversal of the wingtip movement from backward to forward, which is the reason pivotal height is sometimes termed reversal height. There is some thought that the reversal illusion may cause problems to unaware pilots during the final turn on approach to landing, because the turn may well pass through reversal height — at 50 knots ground speed, the reversal height is about 200 feet, at 60 knots it is about 300 feet and at 70 knots it is about 450 feet. If the aircraft is in a banked turn below reversal height, and if the pilot looks down over the wingtip, she/he may get the impression that the aircraft is not turning and may then add additional bottom rudder so that the wingtip then appears to move backwards in the turn — the normal movement. This will cause a yaw and the aircraft's nose will slide down. The aircraft may then appear to be nose-low, and the pilot's reaction is to increase back pressure on the control column. Low speed, excessive bottom rudder and an increasing control column back pressure are the prerequisites for the aircraft to stall and roll toward the lower wing — an incipient spin entry. All pilots should be aware of this illusion and that wind drift will exacerbate it — the turn to final approach is probably the most important ground reference manoeuvre that recreational pilots regularly perform. 3.16.7 Effects of wind shear Shear sources Air flow in the atmospheric boundary layer is normally turbulent to some degree but such turbulence does not significantly alter the aircraft's flight path. Bear in mind that what is a minor variation in flight path at a reasonable altitude may be hazardous when operating at slower speeds very close to the ground in take-off, landing, 'go-around' or perhaps cattle mustering operations. The velocity of near-surface winds is changing constantly; fluctuations in direction of around 20° and in speed around 25% either side of the mean occur every minute. In an unstable boundary layer, the rising air in thermals is accompanied by down-currents from the top of the layer, where the wind velocity approximates the gradient wind — i.e. the direction is backed by 20–30° from the wind at the surface, and the speed is greater. The descending air retains most of these characteristics when it arrives at the surface, thus the gust will back and increase in speed. Except for the vortex turbulence from the wake of preceding aircraft — which is extremely hazardous to light aircraft at low levels because of its horizontal rotational properties — practically all turbulence hazardous to flight is a result of wind shear, a sudden "variation in wind along the flight path of a pattern, intensity and duration, that displaces the aircraft abruptly from its intended path and sufficiently that substantial control action is needed." The shear is the rate of change of wind speed and direction, and its effect on flight can range from inconsequential to extremely hazardous. Vertical shear is the change in the (roughly) horizontal wind velocity with height; i.e. as the aircraft is climbing or descending. Horizontal shear is the change in horizontal wind velocity (i.e. speed and/or direction — gusts and lulls) with distance flown. Updraught, downdraught or vertical gust shear is the change in vertical air motion with horizontal distance. Wind shear can derive from many sources — orographic, frictional, air mass instability, convective downbursts, wave disturbance and thermalic; for a full description see microscale meteorology and atmospheric hazards. The closer to the surface that the shear occurs, the more hazardous it is for aircraft, particularly for low-momentum aircraft. For an aircraft taking off or landing, the shear may be large enough and rapid enough to exceed the airspeed safety margin and the aircraft's capability to accelerate or climb. Thermals as such contribute relatively minor amounts of hazardous turbulence in temperate climates, but can produce very severe turbulence when flying in the superadiabatic conditions common to inland Australia. Changes in aoa and lift Imagine an aircraft flying straight and level that suddenly encounters an area of substantial atmospheric downflow. Due to its inertia (which is a function of mass), the aircraft will momentarily maintain its velocity and flight path relative to the Earth. During that time the 'effective airstream' around the wings will no longer be aligned with the flight path but will have acquired a vertical component. The effective aoa, and consequently CL, will reduce. This produces a momentary reduction in wing loading, the airframe will experience a negative acceleration and the pilot will be restrained by the harness while the seat drops away from her/him. Following initial entry into the downflow, the inertial effects are overcome and the aircraft will restore itself to its trimmed angle of attack. Flight will continue normally, except that the new flight path will incorporate a rate of descent relative to the Earth and equivalent to the atmospheric downflow; i.e. drift now includes a vertical component — sinking air. When the aircraft flies out of the downflow it will again momentarily maintain its flight path relative to the Earth. During that time, the effective airflow around the wings will no longer be directly aligned with the flight path but will have acquired a vertical component opposite to that at entry. The aoa, and consequently CL, will increase. This produces a momentary increase in wing loading, the airframe will experience a positive g load and the pilot will feel the seat pushing up before the aircraft is finally re-established in level flight. A reversed sequence is applicable when encountering upflow; thus encountering changes in vertical flow causes momentary changes in aoa and wing loading, with some variation in the vertical profile of the flight path. If an aircraft is flying straight and level and suddenly encounters a head-on increase in wind speed, then due to its inertia the aircraft will momentarily maintain its velocity (and flight path) relative to the Earth. Thus there will be a momentary increase in air velocity over the wings with subsequent increase in lift. The aircraft will rise until the inertial effects are overcome, then the aircraft restores itself to straight and level flight at an altitude a little higher than previously. Similarly, if the aircraft encounters a head-on decrease in wind speed, then lift will momentarily decrease and the aircraft will sink until the inertial effects are overcome. Thus encountering changes in horizontal flow causes momentary changes in lift with consequent variation in the vertical profile of the flight path. The foregoing is just illustrative, because wind shear events are always a combination of speed variations and three-dimensional variations in direction. Various scenarios have been outlined above where the aircraft could be flying with little margin between effective and critical aoa; it is on occasions like these that Murphy's Law springs into action. What can and will go wrong at those worst possible times is an encounter with wind shear that suddenly increases the effective aoa of the wing and instantly switches on a stall/spin event. A more detailed document about coping with wind shear and turbulence' is available in the 'Decreasing your exposure to risk' guide. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)
-
3.15.1 Airframe strength and elasticity Aircraft structures are designed to be as light as possible with some degree of structural flexibility while still providing adequate strength for the aircraft's operational category. To receive type certification, the design of a general aviation or recreational aircraft must conform with certain standards — among which are the in-flight structural load minimums — for the category in which the aircraft may be operated. In FAR Part 23, a recognised world standard for light aircraft certification, the minimum load factors that an aircraft at maximum take-off weight [MTOW] must be designed to withstand are: +3.8g to –1.5g (or –1.9g) for the normal operational category (which would include most factory-built recreational aircraft). +4.4g to –1.8g (or –2.2g) for the utility category (which includes most GA, and perhaps some RA, training aircraft). +6g to –3g for the acrobatic (i.e. aerobatic) category. +4g to –2g for the Light Sport Aircraft category manufactured in compliance with ASTM F2245-07 standard specification for design and performance of a Light Sport Airplane. Sailplanes and powered sailplanes are generally certificated in the utility or acrobatic categories of the European Joint Airworthiness Requirements JAR-22, which is the world standard for sailplanes; aerobatic sailplanes have limit loads of +7g and -5g. For more information see 'Limiting loads and ultimate loads'. There is an increasing risk of failure when exceeding the minimum load factors, and each instance of excessive loading will compound the failure risk. We use load factors in terms of g for convenience, but what we are also considering is total aerodynamic loading — remember that dynamic pressure increases with the square of the velocity; i.e. dynamic pressure = ½rV². Notes: 1. Uncertificated minimum ultralight aircraft, even with their low wing loading of perhaps 12 kg/m², can be overstressed readily just by flying at maximum level speed and increasing g in a pull-up (positive g) or a push-over (negative g). 2. Many aircraft are type certificated in both normal and utility category, and some are certificated in those plus the acrobatic category. In this case, the MTOW and cg limits are not fixed values, but vary according to the flight operating category. See the table in weight/cg position limitations. Weight and balance There are fixed limits to the payload an individual aircraft may carry safely. The payload must be distributed so that the aircraft's balance — the position of the aircraft's centre of gravity — is maintained within calculated limits. In addition, there is a maximum safe operating weight permitted by the aircraft designer. However, for many recreational aircraft, the MTOW will be limited by national legislation, which has nothing to do with aeronautical engineering. The aircraft's weight and balance very much affect control and stability at high speeds. Excess weight reduces the designed structural load limits, while cg positions outside the designated fore and aft limits may enhance unfavourable reactions to aerodynamic loads, affect stability, reduce controllability, or delay (or prevent) recovery from unusual or high-speed situations. Aeroelastic effects Elasticity is a property of certain materials that enables them to return to their original dimensions after an applied stress has been removed, see the notes on 'stress and strain' in the 'Builders guide to safe aircraft materials'. Elastic structures have a natural frequency of vibration and all aircraft structures exhibit some degree of elasticity; that is, they distort or deform a little, changing shape — flexing, elongating, compressing, bending and/or twisting — under applied aerodynamic loads; each type of distortion produces a particular mode of vibration. Transient structural distortions also contribute to a change in the aerodynamic forces, so the distortions and forces are mutually dependent. This is particularly so with the wings and tailplane. Wings have a low frequency bending mode of vibration where the tips flex up and down (i.e. flap) relative to the wing root, under changing flight loads — in turbulence for example. The degree of oscillation or flapping is more pronounced with high aspect ratio wings. While bending upward the wing adds a vertical velocity* to its forward velocity — the true airspeed — which results in a decreasing angle of attack (aoa) reducing the lift of the up-moving portion of the wing and thus causing an aerodynamic damping of the flapping oscillation. Similarly a downward bending motion results in an increasing aoa, increasing the lift of the down-moving wing and again causing an aerodynamic damping of the flapping oscillation. *A similar resultant velocity concept to a vertical gust encounter. Wings also exhibit a higher frequency torsional mode of vibration where they twist about the wing's elastic axis as the centre of pressure moves chordwise and consequently produces a spanwise variation in the aoa and changes the lift force and its distribution. If the centre of pressure moves forward it can then again increase the wing twist, aoa and lift, developing a non-stable situation. Twisting and bending distortions result in independent oscillations or vibrations and alter the effectiveness of lifting surfaces, though structural and inertial forces provide a natural positive damping that normally keeps vibratory energy in check. The elastic axis is defined as the line along the span of the wing where no torsion occurs when a loading is applied to the wing. A lifting force centred aft of the elastic axis will tend to twist the outer wing leading edge down, reducing aoa and thus aerodynamic force. A lifting force centred forward of the elastic axis will tend to twist the leading edge up, increasing the aoa and the aerodynamic force. The wing aerodynamic centre is usually designed to be close to or behind the elastic axis; if the aerodynamic centre is forward of the elastic axis then wing twist will increase aoa leading to the non-stable situation described in the preceding paragraph. The degree of torsional distortion is dependent on (1) the area of wing surface affected, (2) the distance between the aerodynamic centre and the elastic axis plus (3) the torsional stiffness (rigidity) of the surface. The torsional stiffness designed into the wing resists twisting, and structures usually revert to the normal status when the load is normalised. Aeroelasticity may lead to some problems at high speed, but reducing elasticity means increasing rigidity, which perhaps involves an unwarranted increase in structural weight. So, aircraft structural engineering must be a compromise between rigidity and elasticity. 3.15.2 Aerodynamic reactions to flight at excessive speed Flutter Wing structures are akin to a very-low-frequency tuning fork extending from the fuselage. When a tuning fork is tapped, the fork vibrates at a particular frequency; the stiffer the structure, the higher its natural frequency. The natural frequency of a wing or tailplane structure may apply another limiting airspeed to flight operations related to a self-exciting interaction between elastic, aerodynamic and inertia forces that result in 'flutter' of control surfaces and the structure to which the surface is attached. For example, when the airflow around a wing, tailplane or control surface is disturbed (by aerodynamic reactions, turbulence or pilot inputs) the structure's elastic reactions – twisting and bending – may combine as an oscillation or vibration of the structure that will quickly damp itself out at normal cruise speeds because of the structure's resistance. It is possible that the oscillation does not damp out but is sustained at a constant amplitude (perhaps felt in the airframe as a low-frequency buzz) that is not, in itself, dangerous but may contribute to structural fatigue. At some higher airspeed — the critical flutter speed, where the oscillations are in phase with the natural frequency of the structure — the oscillations will not damp out but will become resonant, rapidly increasing in amplitude. (Pushing a child on a swing is an example of phase relationships and amplification.) This flight resonance – flutter – is very dangerous, and unless airspeed is very quickly reduced, the increasing aerodynamic forces will cause control surface (or even wing) separation within a very few seconds. In 1966 NASA recorded a 24-second video of an in-flight flutter test on a Piper PA30 Twin Comanche demonstrating how rapidly stabilator oscillations increase in amplitude; Google 'NASA flutter video'. The following is an extract from an article by William P. Rodden which appeared in the McGraw-Hill Dictionary of Science and Technology; it provides a succinct description of flutter: "Flutter (aeronautics) — An aeroelastic self-excited vibration with a sustained or divergent amplitude, which occurs when a structure is placed in a flow of sufficiently high velocity. Flutter is an instability that can be extremely violent. At low speeds, in the presence of an airstream, the vibration modes of an aircraft are stable; that is, if the aircraft is disturbed, the ensuing motion will be damped. At higher speeds, the effect of the airstream is to couple two or more vibration modes [e.g. bending plus twisting ... JB] such that the vibrating structure will extract energy from the airstream [JB's emphasis]. The coupled vibration modes will remain stable as long as the extracted energy is dissipated by the internal damping or friction of the structure. However a critical speed is reached when the extracted energy equals the amount of energy that the structure is capable of dissipating, and a neutrally stable vibration will persist. This is called the flutter speed. At a higher speed, the vibration amplitude will diverge, and a structural failure will result." So, flutter is a vibrational instability that (if the structure is not sufficiently stiff) is generally related to the aerodynamic forces and thus the airspeed; but there are many flutter modes. Providing high torsional stiffness in an airframe structure — particularly a high aspect ratio wing — may incur weight penalties that are unacceptable for those aircraft whose MTOW is limited by national legislation, rather than normal design parameters. Mass inertia and the status of the control actuation systems are also involved in flutter development. Consequently ailerons, elevator or stabilator and the rudder (in that order) should be considered for mass-balancing, i.e. their centre of gravity is made coincident with their hinge centre line. This limits the mass moment of inertia so that the control surface does not rotate about its hinge line when the main structure moves; e.g. when the wing bends upward the aileron does not rotate downward but maintains the same floating position relative to the wing. It may be acceptable for the control surface to be over-balanced; i.e. its cg is slightly forward of the hinge line but under-balancing may achieve little. Mass-balancing of the control surfaces, including the rudder, should prevent flutter of that control surface, but the possibility of, for example, wing flexing/twisting flutter might still exist. Mass-balancing of ailerons might be accomplished by attaching moulded lead weight/s, or a lead-filled steel tube, within the nose of the control structure forward of the hinge centre line. As the moment arm, between that centre line and the centroid of the added weight, could be quite short the balance mass needed could be twice the mass of the unweighted control, so it is possible the end effect could be close to tripling the total weight of the aileron. Mass balancing of elevators might be achieved using a weight on an arm contained within the tail structure or within control horns, while a rudder might also be balanced by a weight within a control horn. The friction within aircraft control surface actuating systems adds to the damping of control surface oscillations and this damping ability increases as the oscillation frequency increases. So, it is important that all parts of the control actuating systems are made as rigid and secure as possible and checked to ensure that rigidity is always maintained so that control surfaces cannot deflect without a corresponding movement in the cockpit control. The possibility of destructive flutter increases if any of the following conditions exist: wear in control surface hinges, pulleys, fairleads or guides lack of tension, wear or slop in actuating pushrods/cables/conduited push-pull cables/cranks/torque tubes/turnbuckles safetying wire improperly installed faulty trim tabs. Also water or ice inside control surfaces or absorbed within a foam core; mud outside; additional surface coatings applied after mass balancing; tail buffeting caused by unsteady airflow related to, for example, alterations to the engine exhaust system; or other system anomalies that alter structural reactions also play a role in flutter development. Also see AC43.13-1B Chapter 7 'Aircraft hardware, control cables, and turnbuckles'. This is an extract from an RA-Aus accident investigation report: "(Witnesses) observed the aircraft in a steep dive at what appeared to be full power. The port wing appeared to detach from the aircraft ... The wing that tore away from the fuselage had the attach points intact but had pulled the mountings out of the top of the cockpit. This action would have released the door, which landed close to the wing. The wings were intact but the ailerons were detached. There was no delamination of the fibreglass structure. The ailerons were not mass balanced. The (prototype) aircraft was a conventional design being a high wing, monoplane of composite construction. While the fuselage was a proven design the pilot /builder had designed his own wing including the aerofoil section. The workmanship was excellent and there is no evidence of any lack of structural integrity. The eyewitnesses reported seeing a sort of 'shimmying' from the aircraft. It is believed that this shimmying was aileron flutter which led to the detaching of both ailerons. This same flutter condition would account for the massive forces required to detach the wing from the aircraft in the manner that occurred. Flutter could have been triggered by the wing aerofoil design combined with the manoeuvre the pilot was conducting or from the aileron control design ... The aircraft suffered a massive inflight structural failure almost certainly caused by severe aileron flutter and the aircraft speed in the dive. Any flutter would have been exacerbated by the lack of mass balancing." Vne — the standard limiting airspeed If an aircraft is operated within its specified flight envelope, observing the limiting accelerations and control movements, and maintaining airspeed commensurate with atmospheric conditions, then the only possibilities of in-flight structural failure relate to: improper modification, repair or repainting of the structure control actuating system deficiencies cumulative strain, or minor damages, in ageing aircraft failure to comply with the requirements of airworthiness notices and directives poor care and maintenance of the airframe. Flight at airspeeds outside the envelope (or at inappropriate speeds in turbulent conditions, or when applying inappropriate control loads at high speed) is high risk and can lead to airframe failure. Vne is the IAS, specified by the designer, which should never be intentionally exceeded in a descent or other manoeuvre. For a fuller description of Vne and how it is calculated see 'How fast is too fast?' in the 'Decreasing your exposure to risk' tutorial. Wing divergence Wing divergence refers to a state where — at very low angles of attack and high speed (when the nose-down pitching moment is already very high) — pressure centres develop, which push the front portion of the wing downward and the rear portion upward. This aerodynamic twisting action on the wing structure — while the rest of the aircraft is following the flight path — further decreases the aoa and compounds the problem. The action finally exceeds the capability of the wing/strut structure to resist the torsional stress, and causes the wing to separate from the airframe with no warning. This could be induced if turbulence is encountered at high speed. Control reversal As airspeed increases, control surfaces become increasingly more effective. They reach a limiting airspeed where the aerodynamic force generated by the ailerons, for example, may be sufficient to twist the wing itself. At best, this results in control nullification; at worst, it results in control reversal. For example, if the pilot initiates a roll to the left, the downgoing right aileron will twist the right wing, reducing its aoa and resulting in loss of lift and a roll to the right, probably with asymmetric structural loads. All of which would make life difficult when attempting to roll the wings level during recovery from a high-speed dive. Many of the uncertified minimum ultralights, and perhaps some of the certificated aircraft, have low torsional wing rigidity. This will not only make the ailerons increasingly ineffective with speed (and prone to flutter), but will also place very low limits on Vne and g loads. Vne may be so low that it can be achieved readily in a shallow descent at 75% power. Effect of wing washout Wings incorporating geometric washout have a significantly lower aoa towards the wing tips. At high speed when the wing is flying at low aoa, there are high aerodynamic loads over the wings. However, the outer sections could well be flying at a negative aoa and the reversed load in that area will bend the wingtips down, possibly leading to outer spar fracture. See the accident technical report below. Vertical gust shear and gust loads The effective aoa of an aircraft encountering an atmospheric gust with a significant vertical component (updrafts, thermals, downdrafts, microbursts, macrobursts and lee waves) will be increased momentarily if the air movement is upward relative to the aircraft's flight path, or decreased momentarily if the air movement is downward. Thus, an updraft will increase CL and lift, increasing the aerodynamic loading and lead to an upwards acceleration of the aircraft. The magnitude of the acceleration is determined largely by the change in aoa, the aircraft speed (the higher the speed, the greater is the g load), the design wing loading and the aspect ratio. The lower the design wing loading and/or the higher the aspect ratio, the greater is the change in load factor for a given increase in aoa and the easier it is to overstress the wings at high speed. The effects of shear and gust loads are expanded in the section on wind shear and turbulence. Other effects It is not just the preceding items that may be a problem at high speed. The maximum speed may be limited by the ability of the fuselage to withstand the bending moments caused by the loads on the tailplane necessary to counter the wing's substantial nose-down pitching moment at very low aoa, or the aoa changes due to vertical gust shear, or the extreme loads caused by a high speed pull-up. Applying rudder in a high speed pull-up applies twisting loads to the rear fuselage. Even a very small bird can cause severe damage in a high-speed bird-strike. When nearing the zero-lift angle of attack in a high-speed descent, many cambered wings suddenly experience a strong nose-down pitching moment and the aircraft will 'tuck under' rapidly; this will certainly make the pilot wish she/he was somewhere else. The symmetrical aerofoil wings often used in aerobatic aircraft don't have this problem. Also, the possibility of a runaway propeller in a high-speed dive is always there for those aircraft with a constant-speed propeller governor or perhaps an in-flight adjustable system. The following is a condensed version of an Australian Transport Safety Bureau Technical Analysis Occurrence Report. Note: the Coroner's findings in relation to the fatal accident near Atherton does not support any view that the accident was caused by pilot mishandling; rather, the Coroner's "preference is towards port side wing tip separation as a consequence of the un-airworthy state of the aircraft ..." "An Airborne Edge microlight aircraft impacted terrain during a 2005 flight to Atherton, in Far North Queensland. The pilot, the sole occupant of the aircraft, was fatally injured. In 2006 a similar Airborne Edge aircraft impacted terrain at Cessnock, New South Wales, also fatally injuring the pilot, the sole occupant of the aircraft. In both instances, RA-Aus initiated safety investigations to determine contributing factors to these accidents. During the course of these investigations, similarities in the structural failures of both aircraft were observed. In addition, a third accident involving an Airborne aircraft registered with HGFA with similar structural failure was identified. This accident had occurred in 1996 in Hexham, NSW. In order to determine possible connections between all three accidents, ATSB was asked to conduct technical examination and analysis on recovered parts from the Atherton and Cessnock accidents, to assist the RA-Aus investigation. Information regarding the 1996 accident was taken from coronial findings. In all three accidents, the failure of the main wingspars had occurred near the wingtip. Qualitative analysis of the structural design and loading of the part during this safety investigation and the examination of the coronial findings from the Hexham accident, revealed that all main wingspars had failed under negative G loading. Such loading was likely if the aircraft entered or encountered flight conditions outside the manufacturer's specified flight envelope. Examination of material characteristics of the failed wingspars did not show evidence of material deficiencies that could have contributed to these accidents. The manufacturer's operating handbook prohibited all aerobatic manoeuvres including whipstalls, stalled spiral descents and negative G manoeuvres. The manual specified that the nose of the aircraft should not be pitched up or down more than 45 degrees, that the front support tube of the microlight and the pilot's chest limit the fore and aft movement of the control bar, and that the aircraft should not exceed a bank angle of 60 degrees. Review of photographs of the Airborne Edge, indicate that the wing adopts a degree of twist while in flight. Twist will effect the load distribution by shifting some of the lift from the tips inboard (i.e. more lift is generated in the middle of the wing). Given the structural restraint of the tip struts and battens located at the tip of the trailing edge of the wing, the aerofoil at the wing tip must adjust and try to align with the relative airflow. This results in a smaller amount of lift generated near the wing tips due to a reduced angle of attack to the relative airflow." (Or an aoa reduced below the zero lift aoa, i.e. reversed lift ... JB) 3.15.3 Recovery from flight at excessive speed Generally, excessive speed can only build up in a dive, although just a shallow dive can build speed — and rate of descent — quite quickly. The table below is a calculation of the rate of descent after a few seconds at dive angles of 10°, 30° and 45° for a moderately slippery light aircraft. Dive angle Airspeed (knots) Rate of descent (fpm) 10° 100 1700 30° 150 7500 45° 180 12 500 Recovery from an inadvertent venture into the realm of flight near, or even beyond, Vne is quite straight-forward, but requires pilot thought and restraint in initiating recovery procedures, particularly so if the aircraft is turning whilst diving. Considerable height loss will occur during recovery, so the restraint is required when terra firma is rapidly expanding in the windscreen. Halt the buildup in airspeed by closing the throttle. Unload the wings to some extent by moving the control column to the neutral position or just aft of it. Keep the slip ball and the ailerons centred — the twisting action of excess rudder at very high airspeed may strain the tailplane and rear fuselage. Gently roll off any bank while using coordinated rudder; this will ensure the total lift vector is roughly vertically aligned. Maintain the control column position at neutral or slightly aft to avoid any asymmetric loading arising from simultaneous application of aileron and elevator at high speed. When the wings are level, start easing back on the control column until you are pulling the maximum load factor for the aircraft : +3.8g or +4.4g, perhaps less for some ultralights. Do not pull back so harshly that the aircraft enters a high-speed stall. Hold the applied loading near the maximum until the aircraft's nose nears the horizon, then level off. The aircraft will have sufficient momentum to reach this position before opening the throttle. If you have ample height at the commencement of recovery, then there is no need to pull such high g — particularly if the atmosphere is bumpy when gust loads, added to the high manoeuvring g, may prove excessive. In aircraft not certified for aerobatics, it is best to wait until airspeed is less than Va before pulling g — if circumstances permit. A problem with this procedure is that most light aircraft do not have an accelerometer [g-meter] fitted, so it is difficult to judge the g being pulled. However, if properly executed 60° steep turns are practised, then some idea of the 2g load on your own physiology can be gained. At the higher end of acceleration the average fit person will probably start feeling the symptoms of greyout by 4g. 3.15.4 Recovery from a spiral dive In a well-developed spiral dive, the lift being generated by the wings (and thus the aerodynamic loading) to provide the centripetal force for the high-speed diving turn, is very high, and much of it is directed inward. The aircraft is near the extremes of its design flight envelope, with very high aerodynamic loading and very high speed, well above Va. The pilot must be very careful in the recovery from such a dive, or damaging structural loads will be imposed. If rearward stick force is applied to pull the nose up while the aircraft is turning, the result will be a tightening of the turn and further lowering of the nose, thus dramatically increasing the applied loading or possibly prompting a very punishing high-speed stall. Also aileron to level the wings must be applied with restraint, the aileron on the lower wing will increase the aerodynamic force on the portion of wing ahead of it and move the centre of force further towards that wingtip, so increasing the moment of force at the spar root. The downgoing aileron is also applying a twisting force to the outer wing structure. Sudden or excessive aileron deflection at airspeeds well above Va could well lead to outer wing or full wing separation. Control reversal could also be a factor, see 'High-speed control reversal: will it always roll in the direction you want?' The recommended procedure — for a fixed undercarriage aircraft without propeller pitch control — is: Reduce power. Carefully centralise controls: the forward movement of the control column will partially unload the wings. Smoothly level the wings with aileron while the rudder and elevators are held in the neutral position. As the wings become level with the aircraft still diving at high speed, much of the lift that was providing the centripetal force will now be directed vertically (relative to the horizon); and if up elevator is applied, the aircraft may start a high g pitch-up — even into a half loop. Thus to prevent this, the pilot must hold the elevators in the neutral position while rolling level or even applying further FORWARD stick pressure — before applying aileron — to reduce aoa; but not below the zero-lift aoa, i.e. the load factor must remain positive. At high speed, the stick force required will be high, but the position of the elevator trim should not be altered. Also it is probably not wise to apply two controls simultaneously at very high speeds because of the consequent asymmetric airframe loading. Read this Australian Transport Safety Authority analysis of an inflight breakup most likely caused by excessive control force during spiral dive recovery. The theme common to all problems encountered when moving at very high speed is that there is no warning and little time to do anything about it! The only safe procedure is not to push the high-speed end of the envelope at any height: make gentle, smooth control movements and avoid asymmetric flight loads and never put yourself in the position where you may encounter non-visual flight conditions at low levels. 3.15.5 Notes: compressibility of airflow and Mach number These notes have little value for the recreational aviator, but are included for interest. Except for a slight EAS correction to IAS/CAS, and the possible propeller effects, the compressibility/elasticity of airflow (i.e. the density change resulting from pressure disturbances) does not have any significant airframe aerodynamic effects for aircraft operating at speeds below 200 knots TAS and altitudes below 10 000 feet. Pressure disturbances, or waves, propagate through the atmosphere in all directions, at the speed of sound. Mach 1.0 is the notation for the speed of sound. For aerodynamic purposes airflow speeds are classified within five ranges: Hypersonic flow — airflows greater than Mach 5.0 Supersonic flow — airflows between Mach 1.5 and Mach 5.0 Transonic flow — airflows between Mach 0.8 and Mach 1.5 Subsonic flow — airflows between Mach 0.3 and Mach 0.8 Incompressible flow — airflows below Mach 0.3 The term 'incompressible flow' doesn't mean that air is incompressible; it just indicates that at flow speeds below Mach 0.3 (30% of the speed of sound or about 200 knots TAS), local density variations within the flow — due to compressibility — are insignificant; so aerodynamicists can assume constant density within the flow. At subsonic velocities, significant density changes may occur in the airflow around wings, which will produce flow separation and a turbulent wake — wave drag. The associated drag coefficient builds rapidly at airspeeds above Mach 0.75 then reduces as Mach 1.0 is exceeded. The speed of sound in the atmosphere varies with air temperature. The Mach number is the measure of an aircraft's TAS in relation to the ambient speed of sound. For example, Mach 0.6 indicates that the aircraft's true airspeed is 60% of the speed of sound. The speed of sound is proportional to the square root of the absolute temperature. In the ISA, Mach 1.0 at sea level = 663 knots, and temperature at sea level = 15 °C [288 K]. Thus, if the temperature = −36 °C (237 K) then the ambient Mach 1.0 = 663 × √237/√288 = 601 knots. Thus, Mach 0.60 at 15 °C would be 398 knots TAS, while Mach 0.60 at −36 °C would decrease to 360 knots TAS. Below the tropopause — the speed of sound decreases as altitude increases. A machmeter is an instrument that measures and compares the speed of the aircraft and the speed of sound, using the outside air temperature. It adjusts for actual air density but is still subject to the same position errors as the ASI. The machmeter is usually incorporated within an ASI; the numeric Mach appears in a small window within the ASI dial. You may see references to design diving speed presented as 'Vd/Md' which indicates the speed may be expressed as IAS or Mach number. Other reference airspeeds are presented in similar fashion. For interest, the following table is the maximum permissable speed/altitude for a late 1940s/early 1950s piston-engined naval fighter — the Seafire 47: Altitude feet Max. IAS knots Mach no. Approx. TAS Sea level – 10 000 455 0.78 505 10 000 – 15 000 410 0.78 495 15 000 – 20 000 375 0.78 485 20 000 – 25 000 340 0.78 472 25 000 – 30 000 300 0.78 459 30 000 – 35 000 270 0.78 448 35 000 + 240 0.78 432 Subsonic jet transport aircraft are designed to cruise close to their maximum allowable speed — Vmo/Mmo. Vmo is the limiting indicated airspeed and Mmo is the limiting Mach number. Mmo is probably between Mach 0.80 and Mach 0.85. In normal operations the limiting airspeed is Vmo, up to a change-over pressure altitude (perhaps around 25 000 feet). Above this altitude Mmo becomes the limiting speed value because of compressibility problem restraints. Vmo could be shown as a fixed red line on the ASI (or 'Mach/Airspeed Indicator') but, because the speed of sound decreases as altitude increases, Mmo can't be represented by a fixed marking on the indicator. So, a moving red-and-white striped pointer, the 'barber pole', shows the limiting Vmo/Mmo varying with altitude. It shows the IAS corresponding to the lower of Vmo or Mmo for the current altitude. For further explanation read this Boeing flight operations review document. STRICT COPYRIGHT JOHN BRANDON AND RECREATIONAL FLYING (.com)